Differentiation on R^n ....need/ use of norms ....

[Math Amateur]

I am reading Hugo D. Junghenn's book: "A Course in Real Analysis" ...

I am currently focused on Chapter 9: "Differentiation on Rn" role="presentation">Rn"

I need some help with an aspect of Theorem 9.1.10 ...

Theorem 9.1.10 reads as follows:

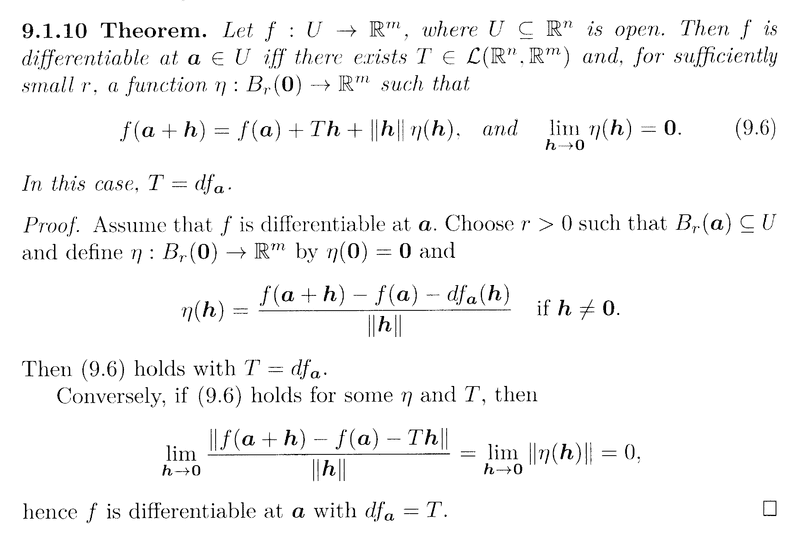
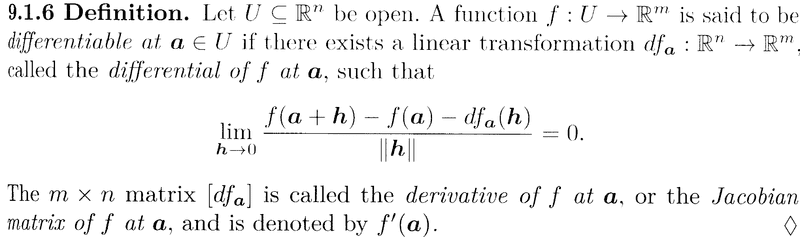
The proof of Theorem 9.1.10 relies on the definition of the derivative of a vector-valued function of several variables ... that is, Definition 9.1.6 ... so I am providing the same ... as follows:

In Junghenn's proof of Theorem 9.1.10 above, we read the following:

" ... ... and

##\eta (h) = \frac{ f(a + h ) - f(a) - df_a (h) }{ \| h \| }## if ##h \neq 0##

... ... "Now there are no norm signs around this expression (with the exception of around ##h## in the denominator ...) ... and indeed no norm signs around the expression ##\lim_{ h \rightarrow 0 } \eta(h) = 0## ... nor indeed are there any norm signs in the limit shown in Definition 9.1.6 above (with the exception of around ##h## in the denominator ...) ...

... BUT ...

... ... this lack of norm signs seems in contrast to the last few lines of the proof of Theorem 9.1.10 as follows ... where we read ...

" ... ... Conversely if (9.6) holds for some ##\eta## and ##T##, then##\lim_{ h \rightarrow 0 } \frac{ \| f( a + h ) - f(a) - Th \| }{ \| h \| } = \lim_{ h \rightarrow 0 } \| \eta(h) \| = 0##... ... "Here, in contrast to the case above, there are norm signs around the numerator and indeed around ##\eta(h)## ... ...
Can someone please explain why norm signs are used in the numerator and, indeed, around ##\eta(h)## in one case ... yet not the other ...
Help will be appreciated ...

Peter

 

[andrewkirk]

The norm signs are not needed in the numerator. The line that contains those is still true if they are removed. It's just that the 0 being referred to is the zero of the vector space ##\mathbb R^m## rather than the scalar 0 of ##\mathbb R_+##.

It may be that the author put the norm signs in the numerator in order to exactly match what was used in the definition of 'differentiable'.

You should satisfy yourself that, if ##g:\mathbb R^n\to \mathbb R^m##, then:
$$\lim_{\mathbf x\to \mathbf a}g(\mathbf x) = \mathbf 0 \Rightarrow
\lim_{\mathbf x\to \mathbf a}\|g(\mathbf x)\| = 0
$$
You can use the theorem for the limit of the composition of two functions, each of which have certain limits.

 

[Math Amateur]

The norm signs are not needed in the numerator. The line that contains those is still true if they are removed. It's just that the 0 being referred to is the zero of the vector space ##\mathbb R^m## rather than the scalar 0 of ##\mathbb R_+##.

It may be that the author put the norm signs in the numerator in order to exactly match what was used in the definition of 'differentiable'.

You should satisfy yourself that, if ##g:\mathbb R^n\to \mathbb R^m##, then:
$$\lim_{\mathbf x\to \mathbf a}g(\mathbf x) = \mathbf 0 \Rightarrow
\lim_{\mathbf x\to \mathbf a}\|g(\mathbf x)\| = 0
$$
You can use the theorem for the limit of the composition of two functions, each of which have certain limits.

Hi Andrew ... thanks for the help ...

But, your post has really got me thinking ...

I think I need some more help, though ...

You write:

" ... ...
You should satisfy yourself that, if ##g:\mathbb R^n\to \mathbb R^m##, then:
$$\lim_{\mathbf x\to \mathbf a}g(\mathbf x) = \mathbf 0 \Rightarrow
\lim_{\mathbf x\to \mathbf a}\|g(\mathbf x)\| = 0
$$
You can use the theorem for the limit of the composition of two functions, each of which have certain limits. ... ... "Can you explain how the theorem for the limit of the composition of two functions, each of which have certain limits. ... ... could be used to prove the above ..

Peter

 

[member 587159]

Hi Andrew ... thanks for the help ...

But, your post has really got me thinking ...

I think I need some more help, though ...

You write:

" ... ...
You should satisfy yourself that, if ##g:\mathbb R^n\to \mathbb R^m##, then:
$$\lim_{\mathbf x\to \mathbf a}g(\mathbf x) = \mathbf 0 \Rightarrow
\lim_{\mathbf x\to \mathbf a}\|g(\mathbf x)\| = 0
$$
You can use the theorem for the limit of the composition of two functions, each of which have certain limits. ... ... "Can you explain how the theorem for the limit of the composition of two functions, each of which have certain limits. ... ... could be used to prove the above ..

Peter

The norm is continuous. Therefore, you can swap limit and norm. The statement becomes trivial then. It is also not difficult to prove this implication starting from the epsilon-delta definition, using the reverse triangle inequality

 

[Math Amateur]

The norm signs are not needed in the numerator. The line that contains those is still true if they are removed. It's just that the 0 being referred to is the zero of the vector space ##\mathbb R^m## rather than the scalar 0 of ##\mathbb R_+##.

It may be that the author put the norm signs in the numerator in order to exactly match what was used in the definition of 'differentiable'.

You should satisfy yourself that, if ##g:\mathbb R^n\to \mathbb R^m##, then:
$$\lim_{\mathbf x\to \mathbf a}g(\mathbf x) = \mathbf 0 \Rightarrow
\lim_{\mathbf x\to \mathbf a}\|g(\mathbf x)\| = 0
$$
You can use the theorem for the limit of the composition of two functions, each of which have certain limits.

The norm is continuous. Therefore, you can swap limit and norm. The statement becomes trivial then. It is also not difficult to prove this implication starting from the epsilon-delta definition, using the reverse triangle inequality

Thanks Math_QED ...

You write:

" ... ... It is also not difficult to prove this implication starting from the epsilon-delta definition, using the reverse triangle inequality ... ... "

Cannot quite see how to do this ... but to start the process ... ...We want to show that if
##g:\mathbb R^n\to \mathbb R^m##, then:

##\lim_{\mathbf x\to \mathbf a} \mathbf g (\mathbf x) = \mathbf 0 \Rightarrow
\lim_{\mathbf x\to \mathbf a}\| \mathbf g (\mathbf x)\| = 0##

Now ...

##\lim_{\mathbf x\to \mathbf a} \mathbf g (\mathbf x) = \mathbf 0##

##\Longrightarrow## ... for every ##\epsilon \gt 0## there exists a ##\delta \gt 0## such that ...

## \| \mathbf{g} ( \mathbf{x} ) - \mathbf{0} \| = \| \mathbf{g} ( \mathbf{x} ) \| \lt \epsilon ##

where ##0 \lt \| \mathbf{x} - \mathbf{a} \| \lt \delta ##

... ... ... But ... where do we go from here ... how do we get a situation where we can use the reverse triangle inequality ...?

Can you help ...?

Peter

 

[member 587159]

Thanks Math_QED ...

You write:

" ... ... It is also not difficult to prove this implication starting from the epsilon-delta definition, using the reverse triangle inequality ... ... "

Cannot quite see how to do this ... but to start the process ... ...We want to show that if
##g:\mathbb R^n\to \mathbb R^m##, then:

##\lim_{\mathbf x\to \mathbf a} \mathbf g (\mathbf x) = \mathbf 0 \Rightarrow
\lim_{\mathbf x\to \mathbf a}\| \mathbf g (\mathbf x)\| = 0##

Now ...

##\lim_{\mathbf x\to \mathbf a} \mathbf g (\mathbf x) = \mathbf 0##

##\Longrightarrow## ... for every ##\epsilon \gt 0## there exists a ##\delta \gt 0## such that ...

## \| \mathbf{g} ( \mathbf{x} ) - \mathbf{0} \| = \| \mathbf{g} ( \mathbf{x} ) \| \lt \epsilon ##

where ##0 \lt \| \mathbf{x} - \mathbf{a} \| \lt \delta ##

... ... ...But ... where do we go from here ... how do we get a situation where we can use the reverse triangle inequality ...?

Can you help ...?

Peter

We prove a more general statement: Let ##a## be a limit point of ##A \subseteq \mathbb{R}^n## (your case is ##A = \mathbb{R}^n## with ##0 = a \in A)##

Let ##f: A \subseteq \mathbb{R}^n \to \mathbb{R}^m## be a function. If ##\lim_{x \to a} f(x) = b##, then ##\lim_{x \to a} \Vert f(x) \Vert = \Vert b \Vert##

Proof: Let ##\epsilon > 0##. Choose ##\delta>0## such that for all ##x \in A## satisfying ##0 < \Vert x-a \Vert < \delta##, we have that ##\Vert f(x) - b \Vert < \epsilon##. Then, whenever ##x \in A## satisfies ##0 <\Vert x - a \Vert < \delta##, we have:

##|\Vert f(x) \Vert - \Vert b \Vert | \leq \Vert f(x) - b \Vert < \epsilon##

Hence, we have proven:

##\forall \epsilon > 0: \exists \delta > 0: \forall x \in A: 0 < \Vert x - a \Vert < \delta \implies |\Vert f(x) \Vert - \Vert b \Vert | < \epsilon##, which is what we wanted to show.

 

[Math Amateur]

We prove a more general statement: Let ##a## be a limit point of ##A \subseteq \mathbb{R}^n## (your case is ##A = \mathbb{R}^n## with ##0 = a \in A)##

Let ##f: A \subseteq \mathbb{R}^n \to \mathbb{R}^m## be a function. If ##\lim_{x \to a} f(x) = b##, then ##\lim_{x \to a} \Vert f(x) \Vert = \Vert b \Vert##

Proof: Let ##\epsilon > 0##. Choose ##\delta>0## such that for all ##x \in A## satisfying ##0 < \Vert x-a \Vert < \delta##, we have that ##\Vert f(x) - b \Vert < \epsilon##. Then, whenever ##x \in A## satisfies ##0 <\Vert x - a \Vert < \delta##, we have:

##|\Vert f(x) \Vert - \Vert b \Vert | \leq \Vert f(x) - b \Vert < \epsilon##

Hence, we have proven:

##\forall \epsilon > 0: \exists \delta > 0: \forall x \in A: 0 < \Vert x - a \Vert < \delta \implies |\Vert f(x) \Vert - \Vert b \Vert | < \epsilon##, which is what we wanted to show.

Thanks Math_QED ... appreciate your help...

Peter