Metric Spaces .... the Uniform Metric .... Garling, Proposition 11.1.11

[Math Amateur]

TL;DR Summary

This thread involves a proof that the uniform metric is indeed a metric ...

I am reading D. J. H. Garling's book: "A Course in Mathematical Analysis: Volume II: Metric and Topological Spaces, Functions of a Vector Variable" ... ...

I am focused on Chapter 11: Metric Spaces and Normed Spaces ... ...

I need some help with an aspect of the proof of Theorem 11.1.11 ...

Garling's statement and proof of Theorem 11.1.11 reads as follows:

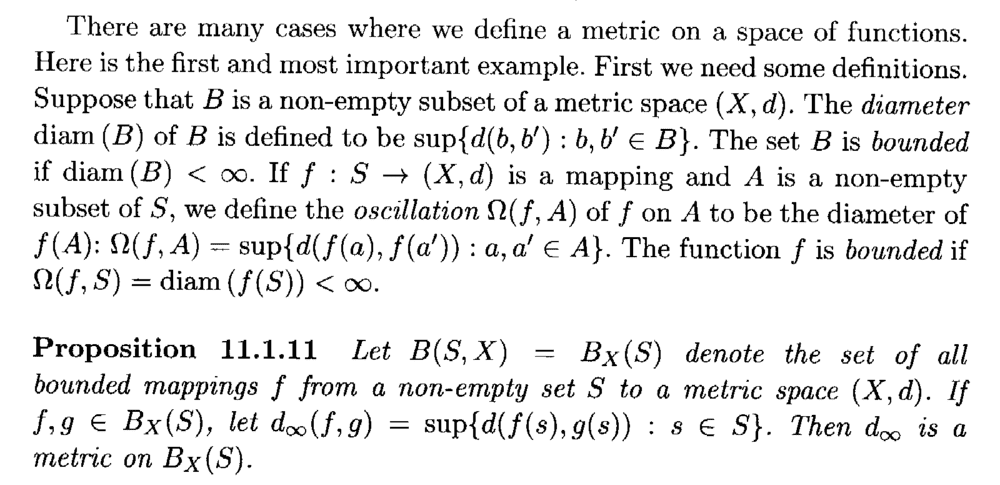
Near the end of Garling's proof above we read the following:

" ... ... Suppose that ##f,g,h \in B_X(S)## and that ##s \in S##. Then

##d(f(s), h(s)) \le d(f(s), g(s)) + d(g(s), h(s)) \le d_\infty (f, g) + d_\infty (g, h)## ... ... ... (1)

Taking the supremum, ##d_\infty (f, h) \le d_\infty (f, g) + d_\infty (g, h)##

... ... ... "Now (1) is true for arbitrary s and so it is true for all ##s## including the point for which ##d(f(s), h(s))## is a maximum ... if a maximum exists ...

But in the case where a maximum does not exist ... how do we know that taking the supremum preserves inequality (1) ...Hope someone can help ...

Peter

 

[pasmith]

Line (1) asserts that [itex]d_\infty(f,g) + d_\infty(g,h)[/itex] is an upper bound for [itex]\{d(f(s),g(s)) : s \in S\}[/itex]. By definition of "least upper bound" it follows that [itex]\sup\{d(f(s),g(s)) : s \in S\} \leq d_\infty(f,g) + d_\infty(g,h)[/itex].

 

[Math Amateur]

Thanks for the help, pasmith ...

Peter