- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Hugo D. Junghenn's book: "A Course in Real Analysis" ...
I am currently focused on Chapter 9: "Differentiation on ##\mathbb{R}^n##" ... ...
I need some help with another aspect of Definition 9.1.3 ...
Definition 9.1.3 and the relevant accompanying text read as follows:
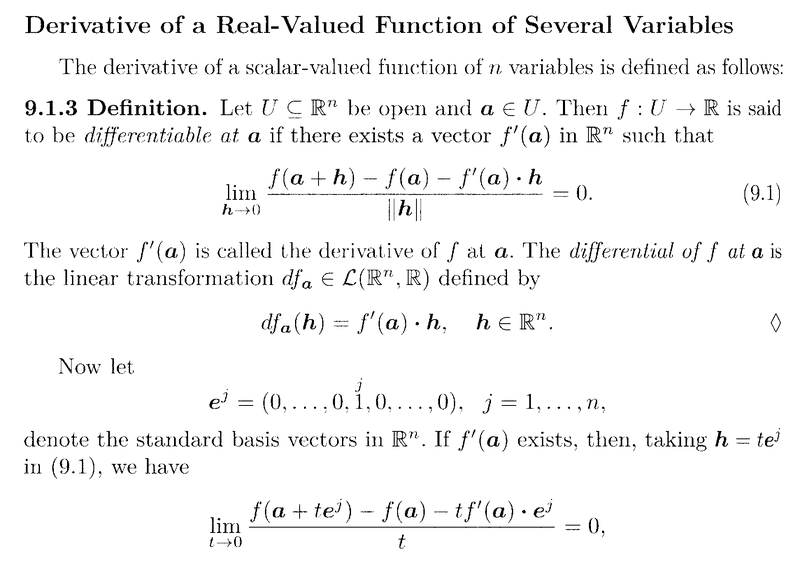
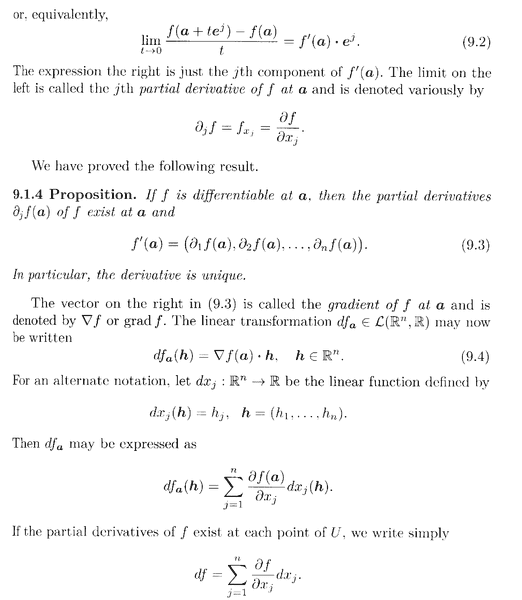
In the above text from Junghenn we read the following:
" ... ... The vector ##f'(a)## is called the derivative of ##f## at ##a##. The differential of ##f## at ##a## is the linear transformation ## df_a \in \mathscr{L} ( \mathbb{R}^n, \mathbb{R} )## defined by
##df_a(h) = f'(a) \cdot h, \ \ \ \ \ \ (h \in \mathbb{R}^n )## ... ... ... "My question is as follows:Is the derivative essentially equivalent to the differential ... can we write ##df_a = f'(a)## ... if if we can't ... then why not?
... ... indeed, what is the exact difference between the derivative and the differential ...(I know I have asked a general question like this before ... but this is now in the specific context of Junghenn ...)
Hope someone can help to clarify the above ...
Peter=============================================================================
In another post it was pointed out to me that the terms total derivative and differential are sometimes used for the same concept ... but this author seems to employ both the term derivative (and Junghenn seems to be defining a total derivative for a scalar function) and differential ...
It may also be that the derivative is ##f'(a)## and the differential is ##df_a(h) = f'(a) \cdot h## ... but then Junghenn states that the differential is ##df_a## ... and hence not ##df_a(h)## ...
Maybe I am making too much of the difference between ##df_a## and ##df_a(h)## ... ...
But my apologies to mathwonk and others if I have misunderstood their posts ...
Peter
I am currently focused on Chapter 9: "Differentiation on ##\mathbb{R}^n##" ... ...
I need some help with another aspect of Definition 9.1.3 ...
Definition 9.1.3 and the relevant accompanying text read as follows:
In the above text from Junghenn we read the following:
" ... ... The vector ##f'(a)## is called the derivative of ##f## at ##a##. The differential of ##f## at ##a## is the linear transformation ## df_a \in \mathscr{L} ( \mathbb{R}^n, \mathbb{R} )## defined by
##df_a(h) = f'(a) \cdot h, \ \ \ \ \ \ (h \in \mathbb{R}^n )## ... ... ... "My question is as follows:Is the derivative essentially equivalent to the differential ... can we write ##df_a = f'(a)## ... if if we can't ... then why not?
... ... indeed, what is the exact difference between the derivative and the differential ...(I know I have asked a general question like this before ... but this is now in the specific context of Junghenn ...)
Hope someone can help to clarify the above ...
Peter=============================================================================
In another post it was pointed out to me that the terms total derivative and differential are sometimes used for the same concept ... but this author seems to employ both the term derivative (and Junghenn seems to be defining a total derivative for a scalar function) and differential ...
It may also be that the derivative is ##f'(a)## and the differential is ##df_a(h) = f'(a) \cdot h## ... but then Junghenn states that the differential is ##df_a## ... and hence not ##df_a(h)## ...
Maybe I am making too much of the difference between ##df_a## and ##df_a(h)## ... ...
But my apologies to mathwonk and others if I have misunderstood their posts ...
Peter
Attachments
Last edited:

