- #1
Math Amateur
Gold Member
MHB
- 3,995
- 48
I am reading Houshang H. Sohrab's book: "Basic Real Analysis" (Second Edition).
I am focused on Chapter 2: Sequences and Series of Real Numbers ... ...
I need help with Theorem 2.1.45 concerning the Supremum Property (AoC), the Archimedean Property, and the Nested Intervals Theorem ... ...
Theorem 2.1.45 reads as follows:
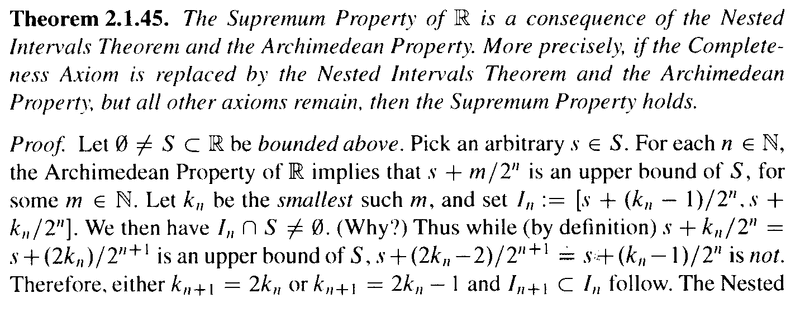
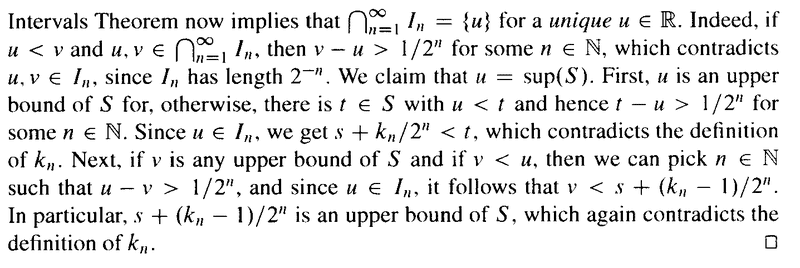 My questions regarding the above text from Sohrab are as follows:Question 1
My questions regarding the above text from Sohrab are as follows:Question 1
In the above text we read the following:
" ... ... ##s + \frac{m}{ 2^n}## is an upper bound of ##S##, for some ##m \in \mathbb{N}##. Let ##k_n## be the smallest such ##m## ... ... "Can we argue, based on the above text, that ##s + \frac{m}{ 2^n} = \text{Sup}(S)## ... ... ?
Question 2
In the above text we read the following:
" ... ... We then have ##I_n \cap S \ne \emptyset##. (Why?) ... ... "Is ## I_n \cap S \ne \emptyset## because elements such as ##s + \frac{ k_n - x }{ 2^n} , \ 0 \lt x \lt 1## belong to ##I_n \cap S## ... for example, the element ##s + \frac{ k_n - 0.5 }{ 2^n} \in I_n \cap S##?
Is that correct ... if not, then why exactly is ##I_n \cap S \ne \emptyset##?Hope someone can help ...
Peter==============================================================================
The above theorem concerns the Supremum Property, the Archimedean Property and the Nested Intervals Theorem ... so to give readers the context and notation regarding the above post I am posting the basic information on these properties/theorems ...
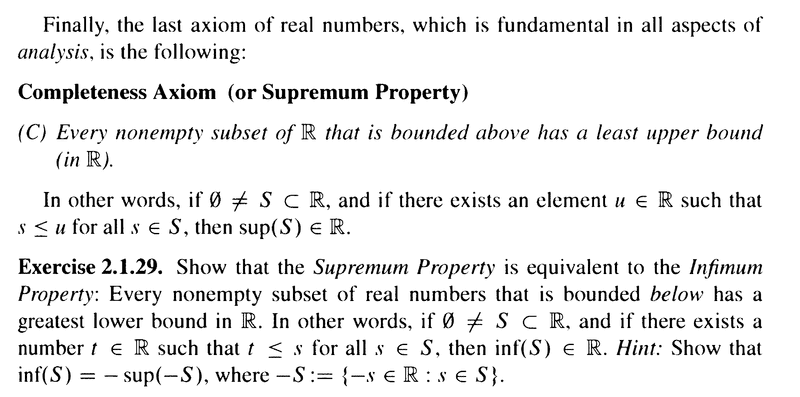

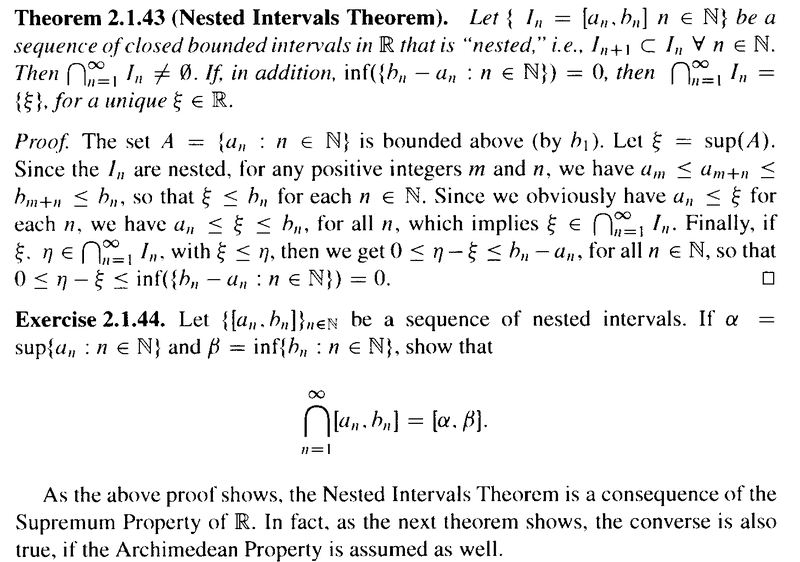
I am focused on Chapter 2: Sequences and Series of Real Numbers ... ...
I need help with Theorem 2.1.45 concerning the Supremum Property (AoC), the Archimedean Property, and the Nested Intervals Theorem ... ...
Theorem 2.1.45 reads as follows:
In the above text we read the following:
" ... ... ##s + \frac{m}{ 2^n}## is an upper bound of ##S##, for some ##m \in \mathbb{N}##. Let ##k_n## be the smallest such ##m## ... ... "Can we argue, based on the above text, that ##s + \frac{m}{ 2^n} = \text{Sup}(S)## ... ... ?
Question 2
In the above text we read the following:
" ... ... We then have ##I_n \cap S \ne \emptyset##. (Why?) ... ... "Is ## I_n \cap S \ne \emptyset## because elements such as ##s + \frac{ k_n - x }{ 2^n} , \ 0 \lt x \lt 1## belong to ##I_n \cap S## ... for example, the element ##s + \frac{ k_n - 0.5 }{ 2^n} \in I_n \cap S##?
Is that correct ... if not, then why exactly is ##I_n \cap S \ne \emptyset##?Hope someone can help ...
Peter==============================================================================
The above theorem concerns the Supremum Property, the Archimedean Property and the Nested Intervals Theorem ... so to give readers the context and notation regarding the above post I am posting the basic information on these properties/theorems ...
Attachments
-
 Sohrab - 1 - Theorem 2.1.45 ... - PART 1 ... ....png37.9 KB · Views: 818
Sohrab - 1 - Theorem 2.1.45 ... - PART 1 ... ....png37.9 KB · Views: 818 -
 Sohrab - 2 - Theorem 2.1.45 ... - PART 2 ... ....png29.9 KB · Views: 878
Sohrab - 2 - Theorem 2.1.45 ... - PART 2 ... ....png29.9 KB · Views: 878 -
 Sohrab - Axiom of Completeness ... Supremum Property ....png31.8 KB · Views: 606
Sohrab - Axiom of Completeness ... Supremum Property ....png31.8 KB · Views: 606 -
 Sohrab - Theorem 2.1.31 - Archimedean Property ... ....png28.3 KB · Views: 2,530
Sohrab - Theorem 2.1.31 - Archimedean Property ... ....png28.3 KB · Views: 2,530 -
 Sohrab - Theorem 2.1.43 ... Nested Intervals Theorem ....png48 KB · Views: 620
Sohrab - Theorem 2.1.43 ... Nested Intervals Theorem ....png48 KB · Views: 620