Rod on a Plane: Calculating Angular and Linear Motion After Impact"

[Nexus99]

Homework Statement

posted in solution

Relevant Equations

Conservation of angular momentum, kinetic energy etc.

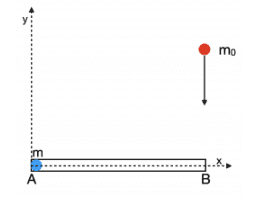
A hollow rod closed at the ends A and B, has mass M and length R. The rod is free
to rotate on a horizontal frictionless plane around the z axis passing through A
and coming out of the sheet. A body can slide without friction inside the cavity
point mass m.
Initially the rod is stationary and the mass m is placed, also stationary, at a distance
negligible but finished by A. A ball of mass m0, with velocity ##\vec{v_0}## = (0,-v0, 0), elastically hits it in B. Calculate:
1. the speed of the ball ##\vec{v_1}## and the angular speed ##\vec{\omega_i}## I of the rod immediately after the impact;
2. the impulse of the constraint reaction ##\vec{J}##

After the impact, the mass m starts up and reaches the end B. Calculate:
3. the angular speed of the rod ##\vec{\omega_{f}}## and the module of the speed ##v_f## of the mass m
with respect to the rod when m reaches B;
4. assuming that the mass m bounces elastically in B, calculate the speed
angle ##\vec{\omega_{A}}## of the rod when m returns to A;
In the event that the rod is not constrained to A and the impact is completely inelastic determine:
5. the equation of motion of the overall center of mass of the ##\vec{r_{CM}}## system;
6. the angular velocity ##\vec{\omega_{CM}}## with respect to an axis passing through the center of mass.

1) angular momentum and kinetic energy is conserved:

## -m_0 v_0 R = m_0 v_1 R + I \omega_1 ##
## \frac{1}{2} m_0 v_0^2 = \frac{1}{2} I \omega_{i}^2 + \frac{1}{2} m_0 v_1^2 ##
Solving the system i got:
## v_1 = \frac{(I-R^2 m_0) v_0}{I + R^2 m_0}##
## \omega_i = -\frac{2 R m_0 v_0}{I + R^2 m_0}##

where ## I = \frac{M R^2}{3} ##

## \vec{v_1} = (0,v_1,0)## and ##\vec{\omega_i} = (0,0,\omega_{i}) ##

2) Impulse of the costraint reaction = - Impulse generated by the ball
## J = -(m_0 v_1 - m_0 v_0) = \frac{2R^2 m_0^2 v_0}{I + R^2 m_0} ##
##\vec{J} = (0,J,0) ##

3) Angular momentum and kinetic energy is conserved
## I \omega_{i} = I \omega_{f} + m v_{f} R##
## \frac{1}{2} I \omega_i^2 = \frac{1}{2} I \omega_f^2 + \frac{1}{2} m v_f^2 ##

and i got
##v_f = \frac{2 I R \omega_i}{I + R^2m_0} ##
##\omega_f = \frac{\omega_{i}(I - R^2 m_0 }{I + R^2m_0} ##

##\vec{\omega_{f}} = (0,0, \omega_{f}) ##

4) momentum and kinetic energy is conserved
## - m v_f = m v_A ##
## \frac{1}{2} I \omega_f^2 + \frac{1}{2} m v_f^2 = \frac{1}{2} m v_A^2 + \frac{1}{2} I \omega_A^2##
and i got
##\omega_f = \omega_A##
##\vec{\omega_A} = (0,0, - \omega_f )##

5) i don't know, i think that ##x_{CM}## is constant since total ##p_{x}## is constant and equal to 0
So i would say ##x_{CM} = \frac{R(2m_0 + M)}{2(m + M + m_0)}##
Neither the momentum along the y-axis nor the kinetic energy is conserved, so i think that the CM along y-axis is moving uniform linear motion but i don't know how to find ##v_{y_{CM}}##
Can you help me?

 

[Nexus99]

I wrote that I would post it in moments, now is on

 

[Delta2]

For 2) i think you calculate the impulse of the reaction force at point B, while problem asks (i think) for the impulse of the reaction force at point A
For 3) i think ##v_f## will have a radial component ##v_{fr}## and a tangential component ##v_{ft}##. The way you wrote the equations you took ##v_f## to be only the tangential component. You should rework the equations taking ##v_f^2=v_{fr}^2+v_{ft}^2## and ##v_{ft}=\omega_fR##. In the angular momentum equation only the ##v_{ft}## will contribute(the contribution of ##v_{fr}## will be zero cause its along the radial direction).

 

[haruspex]

In 1, you have an L that should be R in the expression for omega.
In 2, you have forgotten about the linear momentum the rod acquires.
For 3, I agree with @Delta2 except that the question defines ##v_f## as the radial component. You will need another variable for the tangential component.

 

[Nexus99]

2)
Ok, so J is:
## J = -((m_0 v_1 + I \omega_{i}) - m_0 v_0) ## ?
3) How can i find the tangential component?

 

[Delta2]

2)
Ok, so J is:
## J = -((m_0 v_1 + I \omega_{i}) - m_0 v_0) ## ?
3) How can i find the tangential component?

2) ##I\omega_i## is the angular momentum of the rod. You should add the linear momentum of the rod, not the angular momentum of the rod...Impulse is all about linear momentum (and Torques all about angular momentum).
3) The tangential component is ##\omega_fR##. Think about it the particle does a composite motion, one motion along a circle (of increasing radius) with angular velocity ##\omega_f## and one motion along the radius of the circle.

 

[Nexus99]

Ok i understood
2)
## J = (m_0v_1 + M\omega_{i} R - m_0 v_0) = -\frac{2 R^2 m_0 v_0 (m_0 + M)} {I + R^2 m_0}##
##\vec{J} = (0,-J,0)##

3) ##v_{f} = \omega_{f} R##
## \frac{1}{2} I \omega_{i}^2 = \frac{1}{2} \omega_f^2 + \frac{1}{2}m (\omega_{f} R)^2 ##
i got:
## \omega_{f} = - \frac{\sqrt{I} \omega_{i}}{\sqrt{I + L^2 m}} ##
## v_{f} = - \frac{\sqrt{I} \omega_{i}}{\sqrt{I + L^2 m}} R ##

##\vec{ \omega_{f} } = (0,0, \omega_{f} ) ##

is number 4 good?

 

[Delta2]

Ok i understood
2)
## J = (m_0v_1 + M\omega_{i} R - m_0 v_0) = -\frac{2 R^2 m_0 v_0 (m_0 + M)} {I + R^2 m_0}##
##\vec{J} = (0,-J,0)##

NO ##M\omega_iR## is not exactly the linear momentum of the rod. The linear momentum of the rod equals its mass M times the velocity of its center of the mass.

3) ##v_{f} = \omega_{f} R##
## \frac{1}{2} I \omega_{i}^2 = \frac{1}{2} \omega_f^2 + \frac{1}{2}m (\omega_{f} R)^2 ##
i got:
## \omega_{f} = - \frac{\sqrt{I} \omega_{i}}{\sqrt{I + L^2 m}} ##
## v_{f} = - \frac{\sqrt{I} \omega_{i}}{\sqrt{I + L^2 m}} R ##

##\vec{ \omega_{f} } = (0,0, \omega_{f} ) ##

is number 4 good?

As @haruspex noted the problem defines as ##v_f## the radial component of the velocity ( i didnt notice that myself either). The total velocity of the particle is then ##\vec{v}=\vec{v_f}+\vec{v_t}## where ##v_t## its tangential velocity which equals ##v_t=\omega_fR##. Write the equation of conservation of angular momentum and conservation of kinetic energy for the total velocity ##\vec{v}##. It will be ##v^2=v_f^2+v_t^2## because ##v_f,v_t## are perpendicular. Also in the angular momentum equation only the ##v_t## will contribute, cause ##v_f## is along the radial direction and hence the angular momentum due to ##v_f## is zero.

And no i think number 4 is not correct, i believe the correct answer for 4 is ##\omega_A=\omega_i##, that is, it is like we turn back time and hence the angular velocity will be equal to that, that it has right after the impact with the mass ##m_0##.

 

[Delta2]

From which book is this problem btw, it seems quite complex, though it is based on conservation of angular momentum, momentum and kinetic energy.

 

[Nexus99]

3) So equations should be:
## \frac{1}{2} I \omega_{i}^2 = \frac{1}{2} I \omega_{f}^2 + \frac{1}{2}m( \omega_{f} + v_{f})^2 ##
## I \omega_{i} = I \omega_{f} + m \omega_{f} R^2 ##
i got
## v_f = - \frac{\sqrt{I R^2 \omega_{i}^2 (2 I + mR^2)} + I R \omega_{i}}{I + mR^2} ##
## \omega_{f} = \frac{I \omega_{i}}{I + mR^2} ##

## v = \sqrt{v_f^2 + \omega_{i}^2R^2} ##
## \vec{ \omega_{f} } = (0,0,\omega_{f}) ##

2) I'm not understanding why my first solution is wrong, i thought that the impulse given by the constraint reaction was the opposite of the impulse done by the ball, why is it wrong?

Anyway the problem is not from a book, this is an old exam done in course

 

[haruspex]

3) So equations should be:
## \frac{1}{2} I \omega_{i}^2 = \frac{1}{2} I \omega_{f}^2 + \frac{1}{2}m( \omega_{f} + v_{f})^2 ##

You cannot add ##\omega_{f}+ v_{f} ##. They’re dimensionally different, and if you correct that to make them both velocities you will need to add them as vectors.

I'm not understanding why my first solution is wrong, i thought that the impulse given by the constraint reaction was the opposite of the impulse done by the ball

If that were true the rod could gain no linear momentum, i.e. its mass centre would not move.

 

[Delta2]

3) The equation for conservation of angular momentum looks good but the equation for conservation of kinetic energy does not. The total kinetic energy of the particle of mass m is ##\frac{1}{2}m((\omega_fR)^2+v_f^2)## NOT ##\frac{1}{2}m(\omega_f+v_f)^2##.
2) What you say would be true if the rod possessed no linear momentum after the impact. But it has linear momentum (though it is rotating, it still has linear momentum equal to M times the velocity of the CM)). Part of the impulse from the constraint A goes into changing the linear momentum of the rod, and part into changing the linear momentum of the ball ##m_0##.

 

[Nexus99]

3)
## \frac{1}{2} I \omega_{i}^2 = \frac{1}{2} I \omega_{f}^2 + \frac{1}{2}m( \omega_{f}^2 R^2 + v_{f}^2) ##
## I \omega_{i} = I \omega_{f} + m \omega_{f} R^2 ##

i got:
## v_{f} = -\frac{ \sqrt{I} L \omega_{i}}{I^2 + R^2 m} ##
## \omega_{f} = \frac{ I \omega_{i}}{I^2 + R^2 m} ##

## v = \sqrt{v_f^2 + \omega_{i}^2R^2} ##
## \vec{ \omega_{f} } = (0,0,\omega_{f}) ##

3)
## J = \Delta p = m_0v_1 + M v_{CM} - m_0 v_0 ##
where ## v_{CM} = \frac{\omega_{i} R M + v_{1} m_0}{M + m_0} ##
and ## \vec{J} = (0,J,0) ##

4) For the conservation of kinetic energy:
## \omega_{A} = \omega_{i} ##
and:
## \omega_{A} = (0,0,-\omega_{i})## (since the rotation direction is counter-clockwise

 

[haruspex]

i got:
## v_{f} = -\frac{ \sqrt{I} L \omega_{i}}{I^2 + R^2 m} ##
## \omega_{f} = \frac{ I \omega_{i}}{I^2 + R^2 m} ##

Some errors in there, but maybe just typos. Check for dimensional consistency. (Always worth doing.)

 

[Delta2]

Looks to me like an evil mastermind is behind this problem e hehe.

Seriously , though the problem solution makes use of simple conservation principles, the various concepts (linear momentum, angular momentum, radial and tangential velocity, rotational kinetic energy and linear kinetic energy) are perplexed in a unique way that can make you fall into traps and make the wrong conclusions.

The professor that thought of this problem for an exam wanted to make really hard the life of his students.

 

[Nexus99]

Sorry real soultions are:

## v_{f} = -\frac{ \sqrt{I} L \omega_{i}}{ \sqrt{I^2 + R^2 m} } ##
## \omega_{f} = \frac{ I \omega_{i}}{I + R^2 m} ##

5)
As i wrote in the beginning, ##x_{CM}## is constantly equal to :
## x_{CM} = \frac{R(2m0 + M)}{2(m+M+m0)} ##
while along y-axis the center of mass is moving in a uniform linear motion, and:
##V_{y_{CM}}## can be calculated by conservation of angular momentum:
## - m_0 v_0 = I_{TOT} (R - x_{CM}) V_{CM} ##
## V_{CM} = \frac{-m_0v_0}{(R - x_{CM}) I_{TOT}} ##
where ##I_{TOT} = \frac{MR^2}{12} + \frac{m_0R^2}{4} ##
So:
##\vec{r_{CM}(t)} = (\frac{R(2m0 + M)}{2(m+M+m0)}, \frac{-m_0v_0}{(R - x_{CM}) I_{TOT}}t,0) ##
and ##\vec{\omega_{CM}} = (0,0, \frac{-m_0v_0}{ I_{TOT}}) ##

 

[Delta2]

I haven't check the algebra for ##v_f## but looks ok (why the minus sign btw and what is the L there ?)

Now for 4) what do you think, how you should apply the conservation laws?

 

[Nexus99]

I think you have to do a system where you combine the conservation of momentum and kinetik energy between the time after the collision and that in A

 

[Delta2]

Why conservation of momentum?? Momentum is not conserved for the system of the particle+rod (why not?)

I think we need to consider conservation of angular momentum. 4) is actually the reverse of 3) isn't it? what do you think?

 

[Delta2]

for 5) you are right about ##x_{cm}## but i think in order to calculate ##v_{CM,y}## you need to apply conservation of linear momentum. As i 've told before the total mass times the velocity of the CM corresponds to a linear momentum.
for 6) apply conservation of angular momentum where the axis of rotation is through the CM of the system.

 

[Delta2]

I now saw you edited post #13, for the impulse there you got it right except that i don't know how you got that complex expression for ##v_{CM}##, it is simply $$v_{CM}=\omega_i\frac{R}{2}$$

 

[Nexus99]

4)
I think is something like that
## I \omega_{B} = I \omega_{A} ##
because m has no angular momentum in A

5)i don't think linear momentum is conserved since the collision is inelastic
So applying conservation of angular mometum:
## -m_0 v_0 R = I_{TOT} V_{CM} (R- x_{CM}) ##

## v_{CM} = \frac{-m_0 v_0 R}{I_{TOT}} (R - x_{CM}) ##

##\vec{r_{CM}(t)} = (x_{CM}, V_{CM} t, 0) ##

## \omega_{CM} = \frac{-m_0v_0 R}{I_{TOT}} (R - x_{CM})^2 ##

where
## I_{TOT} = \frac{MR^2}{12} + M (\frac{R}{2} - x_{CM})^2 + mx_{CM}^2 + m_0(R- x_{CM})^2 ##

 

[haruspex]

5)i don't think linear momentum is conserved since the collision is inelastic
So applying conservation of angular mometum:

Both are conserved here.
The inelasticity only affects conservation of work.
Linear momentum is conserved provided there are no external forces acting (there aren't)
Angular momentum about a given axis is conserved as long as there are no external torques about that axis.

The equation you obtained is incorrect anyway. You omitted the rotation of the rod. which is now independent of the linear motion.

 

[haruspex]

4)
I think is something like that
## I \omega_{B} = I \omega_{A} ##
because m has no angular momentum in A

Yes, but it bothers me that the question setter says to assumes the bounce at B is elastic. I do not see the relevance.

 

[Delta2]

4)
I think is something like that
## I \omega_{B} = I \omega_{A} ##
because m has no angular momentum in A

You forgot something, the angular momentum that mass m has in position B. If you plug that into the equation you ll see that you have essentially the second equation in post #13.

5)i don't think linear momentum is conserved since the collision is inelastic
So applying conservation of angular mometum:
## -m_0 v_0 R = I_{TOT} V_{CM} (R- x_{CM}) ##

## v_{CM} = \frac{-m_0 v_0 R}{I_{TOT}} (R - x_{CM}) ##

##\vec{r_{CM}(t)} = (x_{CM}, V_{CM} t, 0) ##

## \omega_{CM} = \frac{-m_0v_0 R}{I_{TOT}} (R - x_{CM})^2 ##

where
## I_{TOT} = \frac{MR^2}{12} + M (\frac{R}{2} - x_{CM})^2 + mx_{CM}^2 + m_0(R- x_{CM})^2 ##

i am not sure i understand how you apply conservation of angular momentum to calculate ##V_{CM}## and ##\omega_{CM}## BUT:
I think we first have to agree on what kind of motion the system will do after the impact. I think it will do a composite motion: The (new) center of mass will move along a straight line down the y-axis with constant velocity, while the rest of the system is rotating around the center of mass. Do you agree with this view?

 

[Delta2]

Yes, but it bothers me that the question setter says to assumes the bounce at B is elastic. I do not see the relevance.

If we don't make this assumption, then a mini paradox will appear if we do the balance of the kinetic energy when the particle returns to A. Somehow the total kinetic energy will be increased (in point A , with regards to point B after the inelastic collision), and we won't be able to infer where this surplus "ghost" energy has come from.

 

[Nexus99]

If we don't make this assumption, then a mini paradox will appear if we do the balance of the kinetic energy when the particle returns to A. Somehow the total kinetic energy will be increased (in point A , with regards to point B after the inelastic collision), and we won't be able to infer where this surplus "ghost" energy has come from.

I agree with your idea.

5)
applying the conservation of linear momentum
## -m_0 v_0 = (M + m + m_0) V_{CM_y} ##
## V_{CM_y} = \frac{ -m_0 v_0 }{ M + m + m_0 } ##

6) i have to apply the conservation of angular momentum, I'm not sure, but i think the equation is something like that:
## -m_0 v_0 R = I_{TOT} V_{CM_y} (R - x_{CM}) + I_{rod} \omega_{CM} ##

 

[Delta2]

I agree with your idea, but now i have no idea to solve the problem

Just use conservation of angular momentum between point B(after the elastic collision) and point A.

 

[Nexus99]

Just use conservation of angular momentum between point B(after the elastic collision) and point A.

Yes, i modified my previous post, sorry

 

[Delta2]

I agree with your idea.

5)
applying the conservation of linear momentum
## -m_0 v_0 = (M + m + m_0) V_{CM_y} ##
## V_{CM_y} = \frac{ -m_0 v_0 }{ M + m + m_0 } ##

This looks good

6) i have to apply the conservation of angular momentum, I'm not sure, but i think the equation is something like that:
## -m_0 v_0 R = I_{TOT} V_{CM_y} (R - x_{CM}) + I_{rod} \omega_{CM} ##

You have to take the angular momentum around the CM of the system. And also the total moment of inertia around the CM of the system. I can't understand what the term ##I_{TOT}V_{CM_y}(R-x_{CM})## is about?

 

[Nexus99]

This looks good

You have to take the angular momentum around the CM of the system. And also the total moment of inertia around the CM of the system. I can't understand what the term ##I_{TOT}V_{CM_y}(R-x_{CM})## is about?

The terms is an error, i tought that ## R - x_{CM} ## was the radius of the circumference along which the system rotates.
I'm not understanding perfectly what you mean (probably because I'm a little dumb) but if i fix that term i got:
## -m_0 v_0 R = I_{TOT} V_{CM_y} (x_{CM}) + I_{TOT} \omega_{CM} ##
is it right?

 

[Delta2]

To be honest i am not sure myself either at this point, i think the correct equation is $$-m_0v_0(R-x_{CM})=I_{TOT}\omega_{CM}$$, where ##I_{TOT}## the moment of inertia of the system around the CM.

 

[Nexus99]

To be honest i am not sure myself either at this point, i think the correct equation is $$-m_0v_0(R-x_{CM})=I_{TOT}\omega_{CM}$$, where ##I_{TOT}## the moment of inertia of the system around the CM.

Ummm ok.
Is the total moment of inertia that I found in post 22 correct?

 

[Delta2]

Yes it is correct.

 

[Nexus99]

Yes it is correct.

Ok thanks for your patience, but this problem was difficult as f*ck.
I hope this year the exam will be a little easier