- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Joseph J. Rotman's book: Advanced Modern Algebra and I am currently focused on Section 6.1 Modules ...
I need some help with the proof of Corollary 6.25 ... Corollary to Theorem 6.22 (Correspondence Theorem) ... ...
Corollary 6.25 and its proof read as follows:
 Can someone explain to me exactly how Corollary 6.25 follows from the Correspondence Theorem for Modules ...?
Can someone explain to me exactly how Corollary 6.25 follows from the Correspondence Theorem for Modules ...?
Hope that someone can help ...
Peter=============================================
*** EDIT ***
The above post refers to the Correspondence Theorem for Modules (Theorem 6.22 in Rotman's Advanced Modern Algebra) ... so I am proving the text of the Theorem from Rotman's Advanced Modern Algebra as follows:
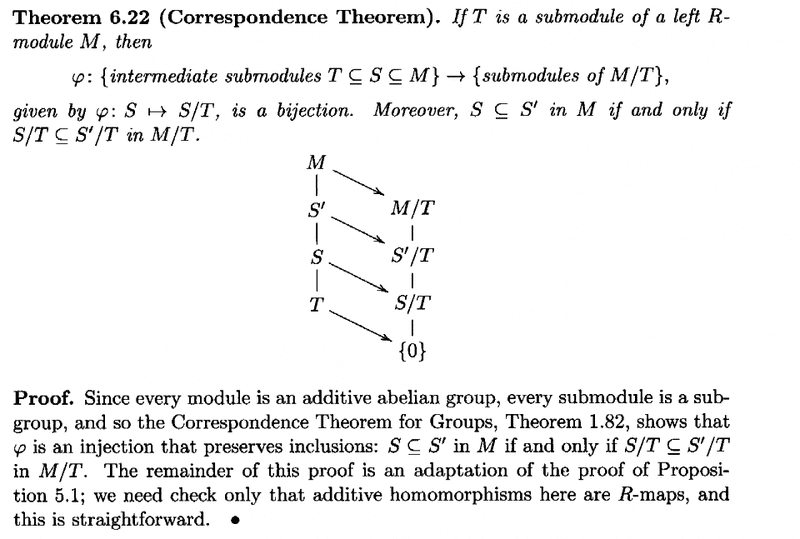
I need some help with the proof of Corollary 6.25 ... Corollary to Theorem 6.22 (Correspondence Theorem) ... ...
Corollary 6.25 and its proof read as follows:
Hope that someone can help ...
Peter=============================================
*** EDIT ***
The above post refers to the Correspondence Theorem for Modules (Theorem 6.22 in Rotman's Advanced Modern Algebra) ... so I am proving the text of the Theorem from Rotman's Advanced Modern Algebra as follows:
