Correspondence Theorem for Groups .... Rotman, Propn 1.82 ....

[Math Amateur]

I am reading the book: "Advanced Modern Algebra" (Second Edition) by Joseph J. Rotman ...

I am currently focused on Chapter 1: Groups I ...

I need help with an aspect of the proof of Proposition 1.82 (Correspondence Theorem) ...

Proposition 1.82 reads as follows:

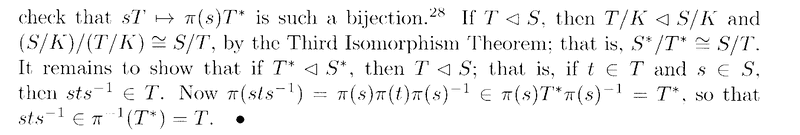
In the above proof by Rotman we read the following:

" ... ... For the reverse inclusion let ##a \in \pi^{-1} \pi (S)##, so that ##\pi (a) = \pi (s)## for some ##s \in S##. It follows that ##as^{-1} \in \text{ ker } \pi = K## ... ... "Can someone please explain exactly how/why ##\pi (a) = \pi (s)## implies that ##as^{-1} \in \text{ ker } \pi = K## ... ...?Peter

===========================================================================================***EDIT***

Just had some thoughts ... BUT ... unfortunately my logic does not seem to line up with Rotman's logic ...

My thoughts are as follows:

##\pi (a) = \pi (s)##

##\Longrightarrow aK = sK##

##\Longrightarrow a = sk## for some ##k \in K## since ##a## must belong to ##sK## ... ... (is this a legitimate step ...)

##\Longrightarrow s^{-1} a = k##

##\Longrightarrow as^{-1} = k##

##\Longrightarrow as^{-1} \in \text{ker } \pi = K##Is that correct?

BUT note ... logic is different from Rotman's set of steps ... ... what is Rotman's logic ...?Peter

 

[fresh_42]

##\operatorname{ker}\pi = \{a \in G\,\vert \,\pi(a)=[e] \in G/K\}## and ##[e] \in G/K## is the coset ##e\cdot K##. Important is that ##\pi## is a group homomorphism:
$$
\pi(a)=\pi(s) \Longrightarrow \pi(a)\cdot \pi(s)^{-1}=\pi(as^{-1})=[e] \in G/K \Longrightarrow as^{-1}\in \operatorname{ker}\pi
$$
by definition of the kernel. Now which elements of ##G## map to ##G/K \ni [e]= e\cdot K\,##?

 

[Math Amateur]

Hi fresh_42 ... thanks for the help ...

Which elements map to ##e_{G/K} = e_{G} K## ... ?

I think it is all the elements of the normal group ##K## ... Is that correct?

Peter

 

[fresh_42]

Hi fresh_42 ... thanks for the help ...

Which elements map to ##e_{G/K} = e_{G} K## ... ?

I think it is all the elements of the normal group ##K## ... Is that correct?

Peter

Yes.

 

[member 587159]

The canonical map ##\pi## is a homomorphism (easy exercise). Hence, ##\pi(a) = \pi(s) \implies \pi(as^{-1}) = \pi(a)\pi(s)^{-1} = e \implies as^{-1} \in \ker \pi##

 

[Math Amateur]

Thanks Math_QED ... most helpful and clear ..

Peter