- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Anthony W. Knapp's book: Basic Algebra in order to understand tensor products ... ...
I need some help with an aspect of Theorem 6.10 in Section 6 of Chapter VI: Multilinear Algebra ...
The text of Theorem 6.10 reads as follows:
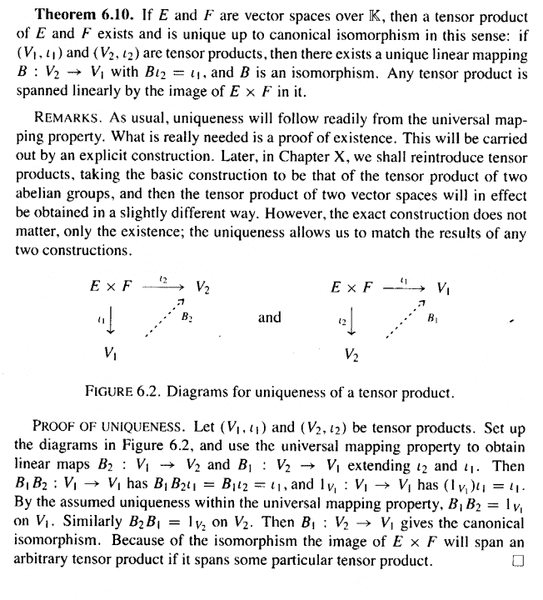
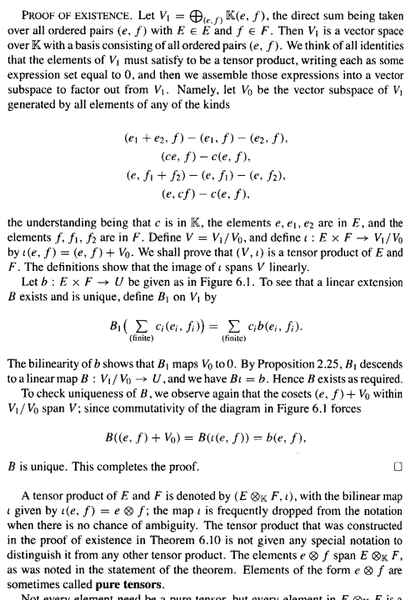
About midway in the above text, just at the start of "PROOF OF EXISTENCE", Knapp writes the following:
" ... ... Let [itex]V_1 = \bigoplus_{ (e,f) } \mathbb{K} (e, f)[/itex], the direct sum being taken over all ordered pairs [itex](e,f)[/itex] with [itex]e \in E[/itex] and [itex]f \in F[/itex]. ... ... "I do not understand Knapp's notation for the exact sum ... what exactly does he mean by [itex]\bigoplus_{ (e,f) } \mathbb{K} (e, f)[/itex] ... ... ? What does he mean by the [itex]\mathbb{K} (e, f)[/itex] after the [itex]\bigoplus_{ (e,f) }[/itex] sign ... ?If others also find his notation perplexing then maybe those readers who have a good understanding of tensor products can interpret what he means from the flow of the proof ...Note that in his section on direct products Knapp uses standard notation and their is nothing in his earlier sections that I know of that gives a clue to the notation I am querying here ... if any readers request me to provide some of Knapp's text on the definition of direct products I will provide it ...Hope someone can help ...
Peter*** NOTE ***
To give readers an idea of Knapp's approach and notation regarding tensor products I am proving Knapp's introduction to Chapter VI, Section 6: Tensor Product of Two Vector Spaces ... ... ... as follows ... ... ... :

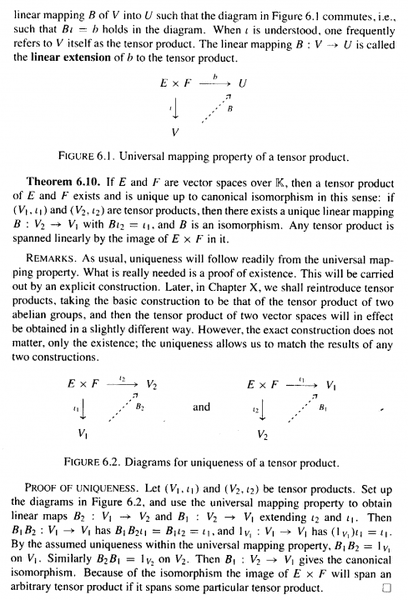
I need some help with an aspect of Theorem 6.10 in Section 6 of Chapter VI: Multilinear Algebra ...
The text of Theorem 6.10 reads as follows:
About midway in the above text, just at the start of "PROOF OF EXISTENCE", Knapp writes the following:
" ... ... Let [itex]V_1 = \bigoplus_{ (e,f) } \mathbb{K} (e, f)[/itex], the direct sum being taken over all ordered pairs [itex](e,f)[/itex] with [itex]e \in E[/itex] and [itex]f \in F[/itex]. ... ... "I do not understand Knapp's notation for the exact sum ... what exactly does he mean by [itex]\bigoplus_{ (e,f) } \mathbb{K} (e, f)[/itex] ... ... ? What does he mean by the [itex]\mathbb{K} (e, f)[/itex] after the [itex]\bigoplus_{ (e,f) }[/itex] sign ... ?If others also find his notation perplexing then maybe those readers who have a good understanding of tensor products can interpret what he means from the flow of the proof ...Note that in his section on direct products Knapp uses standard notation and their is nothing in his earlier sections that I know of that gives a clue to the notation I am querying here ... if any readers request me to provide some of Knapp's text on the definition of direct products I will provide it ...Hope someone can help ...
Peter*** NOTE ***
To give readers an idea of Knapp's approach and notation regarding tensor products I am proving Knapp's introduction to Chapter VI, Section 6: Tensor Product of Two Vector Spaces ... ... ... as follows ... ... ... :



