- #1
chwala
Gold Member
- 2,658
- 351
- Homework Statement
- See attached
- Relevant Equations
- separation of variables
My interest is only on the highlighted part, i can clearly see that they made use of chain rule i.e
by letting ##u=1+x^2## we shall have ##du=2x dx## from there the integration bit and working to solution is straightforward. I always look at such questions as being 'convenient' questions.
Now to my question, supposing we had ##x(1+x^2)^{-\frac{1}{5}} dx## in the place of ##x(1+x^2)^{-\frac{1}{2}} dx##
How would we handle this?
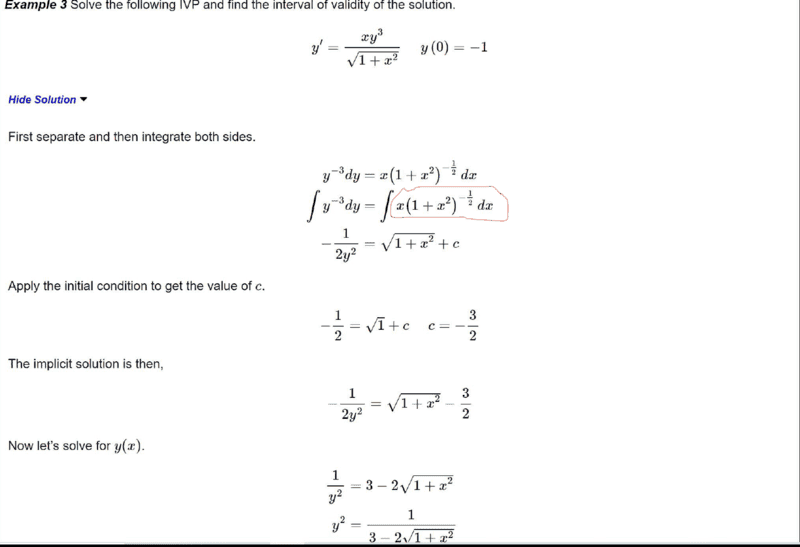
by letting ##u=1+x^2## we shall have ##du=2x dx## from there the integration bit and working to solution is straightforward. I always look at such questions as being 'convenient' questions.
Now to my question, supposing we had ##x(1+x^2)^{-\frac{1}{5}} dx## in the place of ##x(1+x^2)^{-\frac{1}{2}} dx##
How would we handle this?