Problem with the region of an integral

[Amaelle]

Homework Statement

look at the image

Relevant Equations

Stocke theorem!

Greetings!

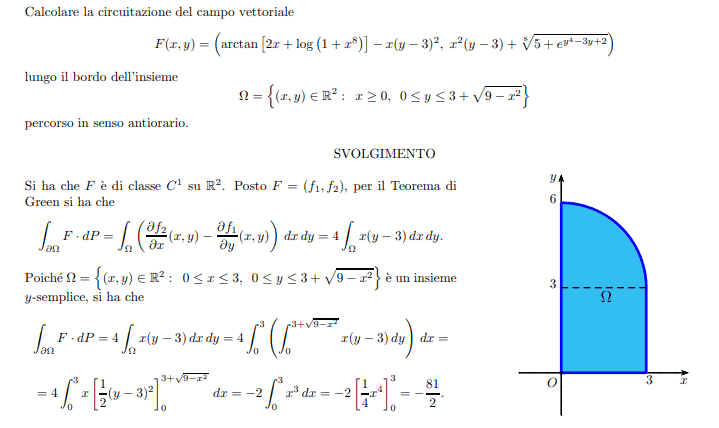
The exercice ask to calculate the circuitation of the the vector field F on the border of the set omega
I do understand the solution very well
my problem is the region!
I m used to work with a region delimitated clearly by two intersecting function here the upper one stop a y=3 and doesn´t intersect with y=0.

thank you!

 

[Mark44]

I m used to work with a region delimitated clearly by two intersecting function here the upper one stop a y=3 and doesn´t intersect with y=0.

No, the upper function doesn't stop at y = 3. The quarter circle does, but the function is given by ##y = 3 + \sqrt{9 - x^2}##.
The region ##\Omega## is (obviously) the portion in blue. The inequality ##0 \le y \le 3 + \sqrt{9 - x^2}## includes all those y-values for which ##x \ge 0##, that run from the x-axis up to the quarter circle. For example, if x = 0, y ranges from 0 to 6; if x = 3, y ranges from 0 to 3.

 

[Amaelle]

No, the upper function doesn't stop at y = 3. The quarter circle does, but the function is given by ##y = 3 + \sqrt{9 - x^2}##.
The region ##\Omega## is (obviously) the portion in blue. The inequality ##0 \le y \le 3 + \sqrt{9 - x^2}## includes all those y-values for which ##x \ge 0##, that run from the x-axis up to the quarter circle. For example, if x = 0, y ranges from 0 to 6; if x = 3, y ranges from 0 to 3.

Thanks a million!