Problem about a ball rolling on a rotating hoop

[Tony Hau]

[No template as this thread was moved to the homework forums after it had attracted several replies]
Here I have a tutorial problem as follows:

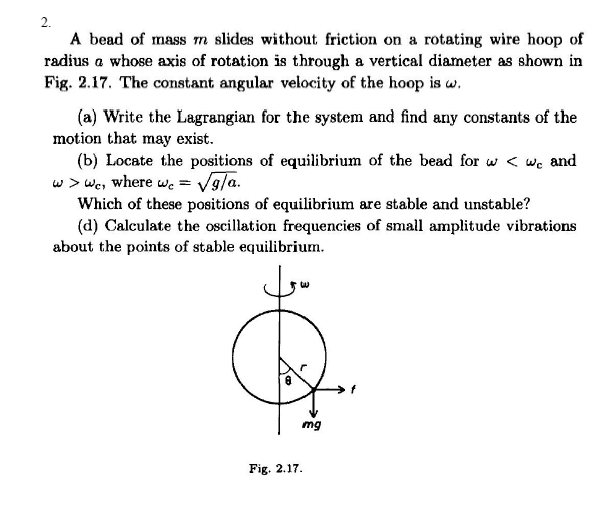
The problem I have is about part a, whose answer is as follows:

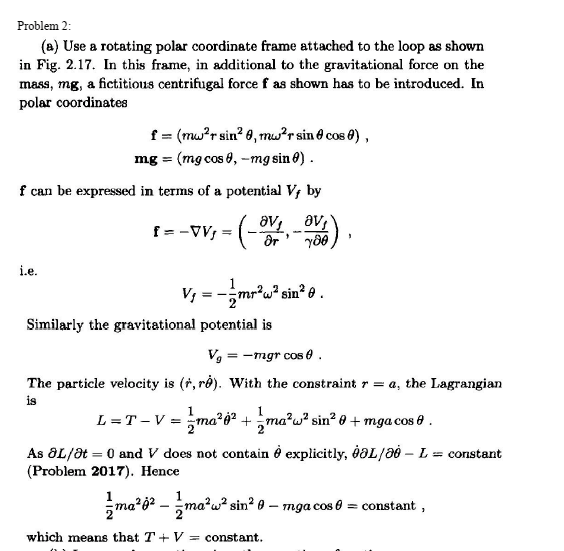
When I solve the partial derivative on Vf w.r.t. r, I get Vf = mω^2rsin^2(θ)/2 +g(θ), where g(θ) is a function of θ.
However, when I take the partial derivate on Vf w.r.t. θ, I get mω^2r^2sin(θ)cos(θ)dθ/dt + dg(θ)/dθ. This is different from the centrifugal force in θ dimension and I am confused.

 

[BvU]

There is a typo in the solution: the ##\gamma## in the denominator should be an ##r##.

I get Vf = mω^2rsin^2(θ)/2 +g(θ)

When I do ##\bigl (\nabla V_f\bigr )_r = {\partial V_{f,r}\over \partial r}\ , \ ## I get ##\ m\omega^2 r\ sin^2\theta##

Probably because I have a different idea what ##\nabla## is in polar coordinates.Oops, too long typesetting .. bravo kuru !

[edit] but there is an ##r## too many in ##-m\omega^2r^2\sin\theta\cos\theta## ...​

 

[kuruman]

When I solve the partial derivative on Vf w.r.t. r, I get Vf = mω^2rsin^2(θ)/2 +g(θ), where g(θ) is a function of θ.

How would you proceed to find ##g(\theta)##? You will have to solve $$\frac{1}{r}\frac{\partial }{\partial \theta}\left[\frac{1}{2}m\omega^2 r\sin^2\theta+g(\theta)\right]=m\omega^2r^2\sin\theta\cos\theta.$$What does that give you for ##g(\theta)##?

 

[kuruman]

There is a typo in the solution: the ##\gamma## in the denominator should be an ##r##.When I do ##\bigl (\nabla V_f\bigr )_r = {\partial V_{f,r}\over \partial r}\ , \ ## I get ##\ m\omega^2 r\ sin^2\theta##

Probably because I have a different idea what ##\nabla## is in polar coordinates.Oops, too long typesetting .. bravo kuru !

[edit] but there is an ##r## too many in ##-m\omega^2r^2\sin\theta\cos\theta## ...​

I posted too soon and had to delete my original message in favor of what I think actually addresses OP's other issue, namely what to do with ##g(\theta)##. It wasn't clear to me that the typo was to blame for that.

 

[wrobel]

There is no need to consider centrifugal force. Write the kinetic energy T relative the lab frame and the potential V of the force mg; L=T-V.

 

[wrobel]

$$T=\frac{1}{2}m((\dot \theta r)^2+(r\omega\sin\theta )^2),\quad V=-mgr\cos\theta$$

 

[etotheipi]

$$T=\frac{1}{2}m((\dot \theta r)^2+(r\omega\sin\theta )^2),\quad V=-mgr\cos\theta$$

Yes I was also confused as to why they use a rotating frame. To make it more difficult? It's a good exercise in any case though

 

[wrobel]

And also derivatives in r are meaningless ; ##\theta## is only generalized coordinate

 

[Tony Hau]

Thanks for all your answers, though I don't have the time to really study them at the moment because of a quiz few days later.

 

[Tony Hau]

$$T=\frac{1}{2}m((\dot \theta r)^2+(r\omega\sin\theta )^2),\quad V=-mgr\cos\theta$$

This is similar to my initial approach. However I thought I was wrong after I had read the solution. Thanks anyway.

 

[Tony Hau]

I have one more extra question about the question. I don't quite understand the last part of the solution. Particularly, when is the energy conserved in Lagrangian?

 

[wrobel]

the energy
$$H=\sum_{i=1}^n\dot q_i\frac{\partial L}{\partial \dot q_i}-L$$
is conserved iff ##L## does not depend on ##t##

 

[etotheipi]

the energy
$$H=\sum_{i=1}^n\dot q_i\frac{\partial L}{\partial \dot q_i}-L$$
is conserved iff ##L## does not depend on ##t##

So if you throw a ball up in the air, ##L = \frac{1}{2}m\dot{y}^2 - mgy \implies \frac{\partial L}{\partial t} = 0 \implies \frac{dE}{dt} = 0##. Although if the total energy is ##E## then just after it is thrown it has Lagrangian ##L = E## and at the top it has Lagrangian ##L = -E##. So the Lagrangian might vary in time, but so long as there is no explicit time dependence we have ##H = \text{constant}##?

 

[Tony Hau]

So if you throw a ball up in the air, ##L = \frac{1}{2}m\dot{y}^2 - mgy \implies \frac{\partial L}{\partial t} = 0 \implies \frac{dE}{dt} = 0##. Although if the total energy is ##E## then just after it is thrown it has Lagrangian ##L = E## and at the top it has Lagrangian ##L = -E##. So the Lagrangian might vary in time, but so long as there is no explicit time dependence we have ##H = \text{constant}##?

For H to be equal to the sum of kinetic and potential energy, two conditions have to be satisfied according to my textbook. Firstly, the potential has to be independent of velocity. Secondly, the generalized coordinates have to be scleronomic, which means that the coordinates have no explicit time dependence. I am wondering if what wrobel has mentioned is what the second condition is saying.

 

[etotheipi]

I think what @wrobel quoted was a Legendre transform. If ##L## has no explicit time dependence then we may show that ##\frac{dH}{dt}=0##. Then as you say ##H## is generally, but not necessarily, ##E##. This requires an additional set of conditions which you quoted, i.e. there be no generalised potential and, also again, no time dependence in the Lagrangian.

 

[wrobel]

Lagrangian might vary in time, but so long as there is no explicit time dependence we have H=constantH = \text{constant}?

Lagrangian does not depend on time means that it does not depend on time as a function $$L=L(t,q,\dot q),\quad \frac{\partial L}{\partial t}=0$$

 

[wrobel]

I think what @wrobel quoted was a Legendre transform.

no the Legendre transform is about p, I did not propose to go to impulses

there be no generalised potential

no problems with generalized potential if only L does not depend on t

 

[etotheipi]

no the Legendre transform is about p, I did not propose to go to impulses

I thought the Legendre transformation relates the hamiltonian ##H(q,p)## to the lagrangian ##L(q, \dot{q})## like
$$H = \frac{\partial L}{\partial \dot{q}}\dot{q} - L$$

 

[wrobel]

I did not express ##H## in terms of ##p## In my formulas ##H=H(q,\dot q)##
This is not the Hamiltonian and this is not the Legendre transform