How does one-to-oneness follow from this?

[pellman]

Given a symmetric operator A on a Hilbert space with inner product

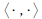and a complex number λ = a + ib, we know that

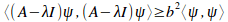for all

.

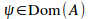The author I am reading then says: this shows that A - λI is injective ( one to one). I don't see how this follows. Can someone explain?

 

[Haborix]

Hello. Recall that an operator ##T## is injective iff ##ker(T)=\{ \textbf{0}\} ## (the set containing only the zero vector). By contradiction, if there were a ##\psi \neq \textbf{0}## such that ##(A-\lambda I)\psi=0##, then, recalling that ##\langle \psi, \psi \rangle >0##, we would have ##\langle (A-\lambda I)\psi , (A-\lambda I)\psi \rangle=0< b^2\langle \psi, \psi \rangle##. This establishes the contradiction, so no such non-zero vector exists in the kernel. Now that we know ##ker(A-\lambda I)=\{\textbf{0} \}## we can use the theorem relating kernel and injectivity of an operator to get that ##A-\lambda I## is injective.

 

[pellman]

Thank you very much.