##GL(n,\mathbb{R})## Spin Reps

[bolbteppa]

Motivated by a statement in GSW (discussed here and here), in this closed thread

https://www.physicsforums.com/threads/why-there-are-no-spinors-for-gl-n.240240/

a discussion asking 'Why there are no spinors for GL(n)' is had.

In trying to follow the proof in one of the references they mention in the pf thread, from https://archive.org/stream/TheTheoryOfSpinors/Cartan-TheTheoryOfSpinors#page/n159/mode/2up, sec. 177, we want the prove the theorem at the bottom of the page:

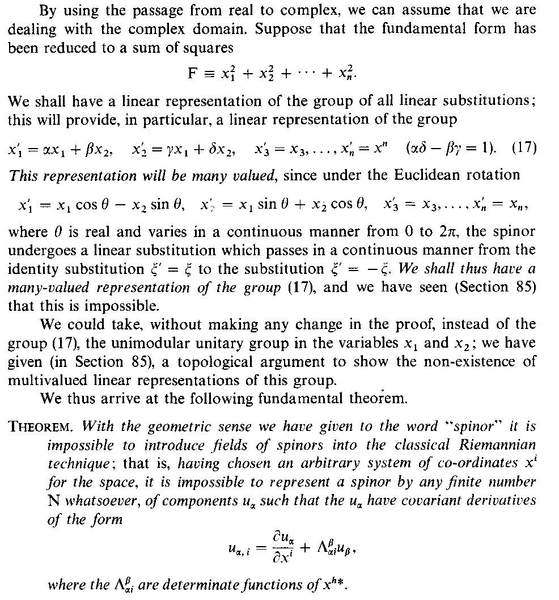
but exclusively for ##x_i## in ##\mathbb{R}##, as is needed in GSW (the proof above does it over ##\mathbb{C}##). Most of the proof, mimicking the above as much as possible, seems to go as follows, for ##D > 2##:

If ##A^{\mu} \mapsto A'^{\mu} = \frac{\partial x'^{\mu}}{\partial x^{\nu}} A^{\nu}## where the matrix ##\frac{\partial x'^{\mu}}{\partial x^{\nu}}## is an element of ##GL(D,\mathbb{R})## admits tensor representations, we want to ask if the spin (projective) representations of ##SO(D)## come out of restrictions from ##GL(D,\mathbb{R})##. Were we able to obtain operators in the spin representation by elements ##\frac{\partial x'^{\mu}}{\partial x^{\nu}} \in GL(D,\mathbb{R})##, it would mean we could restrict the element corresponding to ##x_1' = x_1 \cos(\theta) - x_1 \sin(\theta)##, ##x_2' = x_1 \sin(\theta) + x_2 \cos(\theta)## to an element of the spin representation. But we can see this restriction is double-valued from

$$\begin{aligned}
\zeta_0 &= \pm \sqrt{\frac{x_1-ix_2}{2}} \\
\zeta_1 &= \pm \sqrt{ \frac{-x_1-ix_2}{2} }
\end{aligned},$$
where the components change sign after a rotation by ##2 \pi##
$$\begin{aligned}
\zeta_0' &= \pm \sqrt{\frac{x_1'-ix_2'}{2}} = \pm \sqrt{\frac{e^{i 2 \pi}(x_1-ix_2)}{2}} = \mp \sqrt{\frac{x_1-ix_2}{2}} \\
\zeta_1' &= \pm \sqrt{ \frac{-x'_1-ix'_2}{2} } = \mp \sqrt{ \frac{-x_1-iyx_2}{2} }
\end{aligned}$$
showing that the ##\frac{\partial x'^{\mu}}{\partial x^{\nu}} \in GL(D,\mathbb{R})## must restrict to both ##(\zeta_0,\zeta_1)## and ## (-\zeta_0,-\zeta_1)##, that is, the group of transformations ##x_1' = x_1 \cos(\theta) - x_1 \sin(\theta)##, ##x_2' = x_1 \sin(\theta) + x_2 \cos(\theta)## would have a double-valued spin representation.

Now comes the iffy part.

(If we follow the proof over ##\mathbb{C}##, at this stage we finish the proof with a topological argument - we have found a multivalued representation of the matrix
$$ \begin{bmatrix} a && b \\ c && d \end{bmatrix} = \begin{bmatrix} \cos(\theta) && -\sin(\theta) \\ \sin(\theta) && \cos(\theta) \end{bmatrix}$$
by the spinors ##(zeta_1,zeta_2)## and ##(-\zeta_1,-\zeta_2)##, but multi-valued representations of complex matrices of this form with non-zero determinant are impossible, as the group of matrices is simply connected, so that following a closed path so that the final point is represented by a different spinor to the initial one, and then we shrink the path down to a point at the origin without modifying the final spinor, we obtain a contradiction.)

We need to modify this over ##\mathbb{R}## as the group is no longer simply connected. The jist of the proof seems to be in showing we cannot set up a faithful representation of the group of matrices, by using real projective transformations
$$z' = \frac{az+b}{cz+d},$$
setting ##z = \tan(x), z' = \tan(x')## and then modifying ##a,b,c,d## so that we end up with ##z' \pm \pi##, but I am not clear on this part of the proof and how it actually finishes the proof.

Any help on finishing this proof clearly, really appreciate it!? The proof is given here, sec. 86:

 

[eljose79]

https://archive.org/stream/TheTheoryOfSpinors/Cartan-TheTheoryOfSpinors#page/n159/mode/2up

it is important to carefully examine and understand the proof presented in the reference material. In this case, the proof is attempting to show that there can be no spinors for GL(n) over the real numbers. The key point in the proof is the use of real projective transformations, which are transformations of the form z' = (az+b)/(cz+d) where a,b,c,d are real numbers. The proof then uses the fact that these transformations cannot be represented by spinors, as they are double-valued, leading to a contradiction.

The proof also mentions the use of complex numbers, but it is important to note that this is only to provide a comparison to the proof over the real numbers. In the case of complex numbers, the group of matrices is simply connected, meaning that there is only one path that connects any two points. This is not the case for the real numbers, where there are multiple paths that can connect two points. This is why the proof needs to be modified for the real numbers.

In order to show that the group of matrices cannot be faithfully represented by spinors, the proof uses the real projective transformations to show that there are points that cannot be reached by a single-valued representation. This is done by showing that there are points that are related by a real projective transformation, but cannot be represented by a single spinor. This contradiction proves that there can be no spinors for GL(n) over the real numbers.

Overall, the key points to take away from the proof are the use of real projective transformations and the fact that the group of matrices is not simply connected over the real numbers. These are the key elements that lead to the contradiction and ultimately prove that there can be no spinors for GL(n) over the real numbers.