- #1
alexmahone
- 304
- 0
Find an $\displaystyle f(x)$ such that $\displaystyle \frac{1}{f(x)}$ is defined for all $\displaystyle x$ and is bounded, but $\displaystyle f(x)$ is decreasing.
Last edited:
Find a Bounded, Decreasing $\displaystyle f(x)$
- MHB
- Thread starter alexmahone
- Start date
-
- Tags
- Bounded decreasing
In summary, in order to find a function $\displaystyle f(x)$ such that $\displaystyle \frac{1}{f(x)}$ is defined for all $\displaystyle x$ and is bounded, but $\displaystyle f(x)$ is decreasing, we must have $\displaystyle f(x) \neq 0$ and $\displaystyle f(x)$ must be separated from 0 by a certain value $\epsilon$. One possible function that satisfies this condition is $f(x) = 1 + e^{-x}$. The behavior of $\displaystyle f(x)$ can vary, but it must approach a limit as $\displaystyle x$ approaches infinity.
- #2
Evgeny.Makarov
Gold Member
MHB
- 2,436
- 4
This is not hard. Obviously, we must have f(x) ≠ 0 and moreover f(x) must be separated from 0, i.e., for some ε we must have |f(x)| > ε for all x.
- #3
alexmahone
- 304
- 0
Evgeny.Makarov said:
I'm still not able to find such a function. :(
- #4
Amer
- 259
- 0
Alexmahone said:
what is the domain of the function ? all real numbers ?
- #5
alexmahone
- 304
- 0
Amer said:
Yes.
- #6
Evgeny.Makarov
Gold Member
MHB
- 2,436
- 4
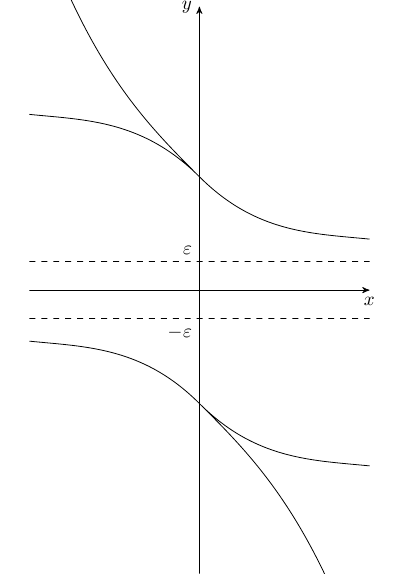
You can't find a decreasing function whose graph lies outside the band $\{(x,y):|y|\le\varepsilon\}$? If you don't know a precise formula, can you at least describe how such function behaves?Alexmahone said:
- #7
alexmahone
- 304
- 0
Evgeny.Makarov said:
Since $\displaystyle f(x)$ is decreasing, $\displaystyle \frac{1}{f(x)}$ is increasing. But $\displaystyle \frac{1}{f(x)}$ is also bounded. So, it must approach a certain limit as $\displaystyle {x\to\infty}$.
- #8
Mathwebster
- 185
- 0
How about $f = 1 + e^{-x}$?
- #9
Evgeny.Makarov
Gold Member
MHB
- 2,436
- 4
Yes, but I was asking really about f(x). Here are the possible behaviors of f(x).Alexmahone said:
Related to Find a Bounded, Decreasing $\displaystyle f(x)$
1. What does it mean for a function to be bounded and decreasing?
A bounded, decreasing function is one that has a finite range and is always decreasing as its input values increase. This means that the function has both an upper and lower bound, and the output values always decrease as the input values increase.
2. How can I tell if a function is bounded and decreasing?
To determine if a function is bounded and decreasing, you can plot the function on a graph and see if it has a finite range and a downward trend. Alternatively, you can also check the function's derivative, which should always be negative for a bounded, decreasing function.
3. Why is it important to find a bounded, decreasing function?
Knowing if a function is bounded and decreasing is important because it can tell us about the behavior and limits of the function. It can also be useful for optimization problems, as bounded, decreasing functions tend to have a global minimum.
4. Can a function be bounded and decreasing on an infinite interval?
Yes, a function can be bounded and decreasing on an infinite interval. For example, the function $\displaystyle f(x) = \frac{1}{x}$ is bounded and decreasing on the interval $\displaystyle x > 0$.
5. What are some common examples of bounded, decreasing functions?
Some common examples of bounded, decreasing functions include exponential functions such as $\displaystyle f(x) = e^{-x}$, logarithmic functions such as $\displaystyle f(x) = \ln(x)$, and power functions such as $\displaystyle f(x) = x^{-2}$. These functions have a finite range and always decrease as the input values increase.
Similar threads
-
Calculus
-
Calculus
-
Calculus
-
Calculus