Example on Z-modules .... Dummit & Foote, Page 339 ....

[Math Amateur]

I am reading Dummit and Foote's book: "Abstract Algebra" (Third Edition) ...

I am currently studying Chapter 10: Introduction to Module Theory ... ...

I need some help with an aspect of Dummit and Foote's example on Z-modules in Section 10.1 Basic Definitions and Examples ... ...Dummit and Foote's example on Z-modules reads as follows:

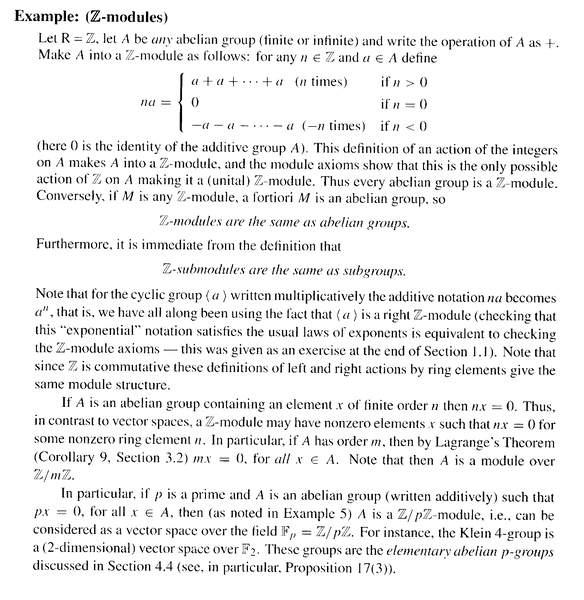
In the above example we read the following:

" ... ... This definition of an action on the integers on ##A## makes ##A## into a ##\mathbb{Z}##-module, and the module axioms show that this is the only possible action of ##\mathbb{Z}## on ##A## making it a (unital) ##\mathbb{Z}##-module ... ... "Can someone please explain how/why the module axioms demonstrate that this is the only possible action of ##\mathbb{Z}## on ##A## making it a (unital) ##\mathbb{Z}##-module ... ... ? How do we know it is the only possible such action ... ... ?Hope someone can help ...

Peter

 

[member 587159]

Hey peter.

Take any action ##\mathbb{Z} \times A \to A: (n,a) \mapsto n.a## satisfying the module axioms.

It is easy to see that

##n.a = (1+1+ \dots +1).a = 1.a + \dots + 1.a = a + \dots + a## if n > 0.

The other cases can be treated in the same way. Since I assumed nothing but the action axioms, it follows that if there is an action, it must look like the action that is written is your book.

Hope this helps.

 

[fresh_42]

We have ##1.a=a## and ##0.a=0##. Then ##n.a=((n-1)+1).a=(n-1).a+1.a=(a+\ldots+a)_{n-1\text{ times }} \cdot a + a=n\cdot a## by induction. The same works for ##-a## if we use ##0=0.a## to show ##(-1).a=-a\,.##

Remark: Here ##n.a## stands for an operation of ##\mathbb{Z}## on ##A## and ##n\cdot a## for ##n## additions, resp. subtractions of ##a##, because at prior, ##n\cdot a## isn't defined, so it is merely a short hand notation of ## a+\ldots +a## (##n## times), which is defined. The assertion is basically that the two don't have to be distinguished, and we can speak of the operation, which makes Abelian groups (additively written) naturally into a ##\mathbb{Z}-##module.