- #1
ATY
- 34
- 1
Hey guys, so my professor told me to take a look at an equation, because he thinks that there is a mistake. We are basically talking about exercise 6.3 (on last image). The pictures will show you the text, so that you have all the information, that I have
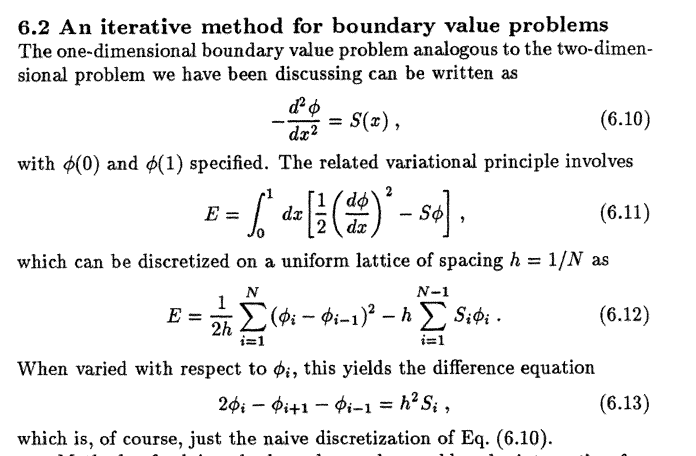
http://puu.sh/mrNDl/ec19cdff63.png
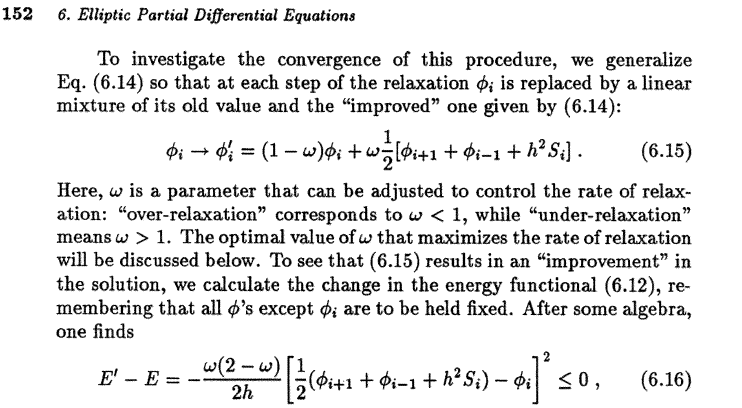
http://puu.sh/mrNF3/7461f97ad4.png
So... I should "just use" 6.12 and 6.15 to get 6.16.
The point is that I have no clue how to do this.
my attemp was
http://puu.sh/mJ1HR/08899e8813.png
but, this gets super ugly when I start to insert the phi' from 6.15 and calculate everything. Is this even the right idea ?
http://puu.sh/mrNDl/ec19cdff63.png
http://puu.sh/mrNF3/7461f97ad4.png
So... I should "just use" 6.12 and 6.15 to get 6.16.
The point is that I have no clue how to do this.
my attemp was
http://puu.sh/mJ1HR/08899e8813.png
but, this gets super ugly when I start to insert the phi' from 6.15 and calculate everything. Is this even the right idea ?
Last edited by a moderator: