BRS: Random Comments on Some Recent PF Threads

[Chris Hillman]

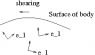
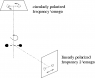
Citations for Parallel Transport

Thanks, George, this is just what we need! I'll try to see if I can find Fecko's book in the library. When I get a chance, I'll try to write a BRS post on parallel transport. The daunting thing is that I should first write posts on Lie groups and Lie algebras, vector fields, connection the Cartan way, Maurer-Cartan form... I know this isn't as hard as I am making it sound, which is making it frustrating. Clearly working some well chosen explicit computations the Cartan way would be an essential goal of the projected BRS thread!

 

[Dale]

The Fecko book is also available on Google books:
http://books.google.com/books?id=vQ...C0Q6AEwAA&safe=active#v=snippet&q=385&f=false

But of course, that is always somewhat hit-or-miss.

 

[Chris Hillman]

I never use Google, for the obvious reasons.

Re what George said about "history of fringe claims", I have to revise my judgement: IMO Anamitra does appear to be behaving like a genuine pseudomath crank, not a troll. The list of bizarre mistakes he has made in the cited thread along include:

• appears to claim spheres can't exist (he's confused by the coordinate singularity at North pole in the standard trig chart)
• appears to claim parallel transport is path in-dependent (oddly enough, he appears to be trying to use frame fields rather than coordinate bases, which would be good, but he's apparently confused by one example where the frame fields he is using happen to parallel transported around the curve he happens to be considering, and he also assumes that "broken line" curves are "improper")

Random selection of weirdities just from the first few pages of just the one thread:

You are getting these results because you are considering a spherical space-time surface [actually, an ordinary round Riemannian sphere] which should not exist in practice.
...
Near the Earth's surface the "geodesics" are great circles possibly due to the impenetrability of the Earth and not due the"strong" curvature of space-time.
...
If we have two geodesics connecting a pair of points A and B , the particle at the point of intersection would be in a state of indecision (as to which spacetime curve it should follow).
...
So if we parallel transport a vector along different paths starting from the same point the components do not change, when referred to the local inertial frames.
...
So if we parallel transport a vector along different paths starting from the same point the components do not change,when referred to the local inertial frames.
...
This "demonstrably false" notion [Anamitra's claim that parallel transport is path independent] arises out of the fact that the singularities north and south poles have been chosen simultaneously.
...
But if one is interested in the parallel transporting a vector at a stretch along a curve it should not be one with sharp bends. In such an instance it cannot be called parallel transport in the totality of the operation.

He's also been very slow to recognize that he is using the word "thread" improperly, and very slow to use the quotation feature of VB and the LaTex features of PF. And he insists on using brackets when others would use parentheses. He refers repeatedly to "physical distance" (in the large), he repeatedly confuses coordinate singularities with geometrical singularities...

All in all, he seems to take the typical crank attitude that "it must be a good idea" [sic] to insist on doing the same incorrect thing over and over again... not to mention his insistence that the world adopt his private terminology, rather than adapting his writings to use standard technical terms correctly.

 

[Chris Hillman]

"Geodesic doubts", plus: local vs ultralocal

Re User:TrickyDicky's thread "Geodesic doubts"

www.physicsforums.com/showthread.php?t=424278

Let's imagine a test particle in outer space not being subjected to any significant force, gravitational(far enough from any massive object) or any other. Its path would be describing a geodesic that follows the universe curvature, right? Would that be an euclidean straight path, or would it follow a curved path, like an ellipse or a hyperbola?

The question as stated is confused almost beyond repair. Ambiguities include:

• gravitational force, in gtr?
• if TrickyDicky meant a test particle in a cosmological model such as FRW model, then dust models have the property that the world lines of the dust particles are timelike geodesics, and it makes sense to consider other timelike geodesics (world line of a particle in inertial motion which is not stationary wrt nearby dust particles),
• as bcrowell noted, in a curved manifold, geodesics are "straight" by definition,
• if TrickyDicky meant to ask about the coordinate equation of a world line, that will only be valid wrt a particular chart and will probably not have a simple geometrical/physical interpretation.

TrickyDicky then restated his question as

I guess what I really meant was what kind of curvature does our spacetime have.

One wonders if he has any idea how frustrated that would make some feel if they tried to answer his first (completely different) question!

And unfortunately, his new question is still ambiguous:

• By "our spacetime", does he mean "best fit FRW model to current observations of our own universe?"
• By "curvature", does he mean the Riemann tensor of the spacetime?
• Or does he mean the Riemann tensor of a spatial hyperslice? If so, there are infinitely many foliations of any spacetime into a family of spatial hyperslices. Does he mean the family orthogonal to the world lines of the dust particles? If so, such a family exists and is unique only if the congruence of world lines of dust particle is irrotational (vorticity tensor vanishes).

Needless to say, I recognize that one needs to try to have patience with people who, due to inadequate understanding of topic T, are unable to clearly express an unambiguous question about some issue regarding topic T.

when I've read descriptions of the Einsten model of the universe, IIRC they talk about a hypersphere embedded in Euclidean ambient space.

There is an Einstein cosmological model, the static lambdadust isometric to [itex]R \times S^3[/itex], with the obvious -+++ signature, which is not consistent with observations and would in any case be unstable against small perturbations. Most likely he is misremembering someone describing a figure in MTW, Gravitation which represents H^3 as a hyperboloid in E^{1,3}; some FRW models feature H^3 hyperslices orthogonal to the world lines of the dust or fluid particles.

In a nonexpanding spacetime I guess the curvature would be that of the spatial part of the line element, right?

In a typical cosmological model in gtr, i.e. a four-dimensional Lorentzian manifold with a stress-energy tensor describing matter, which satisfies the EFE, there is a gravitational field which is due to the presense of matter, which is often modeled as a perfect fluid or dust (pressure-free perfect fluid). In such models, it makes sense physically and geometrically to compute/describe the expansion scalar, shear tensor, and vorticity vector of the congruence of world lines of dust/fluid particles. As George Jones noted, for an expanding phase, the expansion scalar will be positive. If the vorticity vector vanishes, it makes sense to compute/describe the Riemannian geometry of the (unique) family of three dimensional spatial hyperslices which are everywhere orthogonal to the congruence of world lines of dust/fluid particles. This three dimensional curvature tensor is not the same as the "spatial components" of the Riemann tensor of the spacetime itself, however.

Ich's suggestion of constructing a Riemann normal chart is interesting.. and for the FRW dust with E^3 hyperslices orthogonal to the world lines of the dust particles it should be easy enough.

I was asking for the case of a non-expanding spacetime manifold just to fix concepts before I go into the more geometrically complex FRW metric.

The FRW models are the simplest cosmological models in gtr which resemble our universe even approximately.

"Non-expanding spacetime manifold": is it possible that TrickyDicky is trying to ask about a stationary cosmological moodel? (Timelike Killing vector field, not neccessarily vorticity-free?) If so, the better known candidates include the Einstein lambdadust already mentioned, which is static, and the Goedel lambda-dust (homogeoneous but world lines of dust particles has nonzero vorticity).

Expansion of space is purely coordinate dependent. Or better, as George Jones puts it, "expansion" is a property of a congruence, like the one defined by the canonical observers in a FRW metric. It's not a property of spacetime.

I agree with what George Jones said, but the bolded statement is IMO potentially misleading. In fact I am not sure what technical statement Ich had in mind here. Ich? Or am I talking to myself?

But this seems to be at odds with General Covariance, according to which only those properties that are invariant under changes of coordinates are physically real, so if expansion vanishes just by a change of coordinates as youare claiming, then expansion is a coordinate artifact rather than a physical fact.

I see why he's confused here. I'd reassure him that the acceleration vector, expansion scalar, shear tensor, vorticity vector of a timelike congruence is a coordinate-free geometric description of the relative motion of the particles whose world lines comprise the timelike congruence. In the case of a family of particles in a state of inertial motion, the congruence of their world lines will be a timelike geodesic congruence, so the acceleration vector will vanish identically. Particles in a perfect fluid, or charged particles in an EM field, will generally have nonzero forces acting so will generally have nonzero acceleration (acceleration just means path curvature of a world line, which has units 1/length and is not to be confused with curvature of a surface, a hyperslice, or of spacetime, all of which have units 1/area).

However, if you choose some other congruence in the very same spacetime, you might find completely different acceleration vector, expansion scalar, shear tensor, vorticity vector!

So the freedom George Jones had in mind, I am pretty sure, is the freedom to choose a congruence. Sometimes a choice is "natural", e.g. in the FRW models, it makes sense to single out the congruence of world lines of material particles (dust or fluid, depending upon the model). Also, in some models, some congruences may be distinguished by being particulary symmetrical (in the FRW models, more or less by contruction, this is true for the congruence of world lines of material points).

From what I've been learning from this thread, we have grounds for selecting a 'canonical' coordinate system - i.e. a point of view where the universe appears homogenous and isotropic.

In an FRW model, that turns out to be the same thing as picking out the congruence of world lines of the particles whose mass-energy is collectively producing the gravitational field (curvature of spacetime). If we compute the Einstein tensor wrt the associated frame field, all but one component vanishes; the only nonzero component at p is positive and represents the matter density of the dust, as measured by the dust particle whose world line passes through event p in our spacetime (M,g). It is often convenient to think of tensor fields as smooth sections in an appropriate tensor bundle over M, incidentally. Then we are saying that for our special frame field, the same information is conveyed by the graph of a smooth function on M.

In "The speed of light?", when bcrowell wrote

"Local" is actually very difficult to define rigorously [Sotiriou 2007], but basically the idea is that if your apparatus is of size L, any discrepancy in its measurement of c will approach zero in the limit as L approaches zero.

I'd caution that there is a huge terminological ambiguity in the physics literature (but not the math literature) regarding "local", which has traditionally been tolerated on the grounds that, allegedly, "the intended meaning will be clear from context". However, from my reading of arXiv eprints by persons not experienced in gtr, it is quite clear that this assumption is invalid: real physicists are getting confused, all the time. Matt Visser has started using "ultralocal" to refer to the level of tangent spaces and jet spaces, and "local" to refer to the level of local neighborhoods (consistent with huge math literature on fiber bundles and suchlike, and with the huge literature produced by math-knowledgeable physicists like Witten).

Jet spaces are one of those concepts everyone should know, but few do, even though it would have been well known to any well-educated mathematician of the late 19th century*. The basic idea is very simple: in addition to some function, include its first and second order derivatives (or partial derivatives) as "additional variables". This is just what you need to get started in Lie's theory of the symmetry of a differential equation (ODE or PDE). To be the topic of another long-deferred BRS thread.

*Just noticed the bolded phrase could sound snooty :blush: Actually, I was trying to express wry despair at the fact that the explosive growth of mathematics in the 20th century inevitably meant that many valuable notions like jet spaces and Frobenius cocycles became undeservedly obscure. So that even well educated persons in the 21st century might not know really valuable concepts which would have been well known to a well educated person of the 19th century.

 

[Chris Hillman]

BRS: Roy Kerr at PF, plus Penrose diagrams generally

Re "Can one diagonalize the Kerr metric?"

www.physicsforums.com/showthread.php?t=247794

Is it possible to diagonalize the Kerr metric in the Boyer-Lindquist coordinates? If so then I think calculations with the metric will become easier. I forget under what condition a matrix can be diagonalized. Can anybody remind me?

I was about to answer this when I saw that User:tagsdad gave a correct answer, in fact better than what I would have said which would have been less specific and probably less concise. And appeared to sign "Roy Kerr", although I am guessing this user means he is posting the result of an email inquiry to Roy Kerr.

It might be worth adding that the Doran chart generalizes the Painleve chart for the Schwarzschild vacuum to the Kerr vacuum.

Re "Penrose diagrams in general"

www.physicsforums.com/showthread.php?t=422583&page=2

If Penrose-Carter diagram means:

• compactificated space-time diagram and
• null-like world lines are 45 degree lines

than, every spherically symmetric space-time has Carer-Penrose diagram? If Yes, can you cite a paper about proving the existence?

Else: on the 2D Carter-Penrose diagram only radial motion can be studied, what about 3D=2+1 Penrose-Carter diagrams? Can you draw it? The 2D diagram is simply rotated?

Those are all good questions, which I should have addressed in the BRS thread. I'll say more there, but briefly: "Penrose-Carter" diagram is a somewhat informal term, but in general the idea is to find a chart such that

• the line element is conformal to some easy to understand line element such as Einstein static model (used in the standard diagram for Minkowski spacetime),
• all coordinates have finite ranges, so that the entire spacetime is "mapped" in a finite region, in the sense of Mappa Mundi,
• asymptotically flat sheets should have a similar boundary to the usual diagram for Minkowski spacetime, and similarly sheets asymptotic to some familiar nonflat manifold should (perhaps) exhibit this relation in the chart,
• in particular, asymptotically flat sheets should have loci labeled scri^+, scri^-, i^+, i^0, i^-.

Thus, a Penrose-Carter chart for a four-dimensional Lorentzian manifold is a local coordinate chart, often with coordinates which can be identified as "angular coordinates" on an exterior sheet. Often one suppresses one or two angular coordinates, so that each "point" in the resulting "Penrose diagram" denotes a round sphere or a circle of some radius (larger near the boundary of any asymptotically flat sheet, smaller in the interior, generally).

In the case of boost-rotation symmetric vacuum solutions (see the BRS on Weyl vacuums), it is in fact convenient to draw three dimensional Penrose diagrams in which only one angular coordinate has been suppressed, so that each "point" in the diagram is really a circle.

 

[George Jones]

Re "Can one diagonalize the Kerr metric?"

www.physicsforums.com/showthread.php?t=247794

I was about to answer this when I saw that User:tagsdad gave a correct answer, in fact better than what I would have said which would have been less specific and probably less concise. And appeared to sign "Roy Kerr", although I am guessing this user means he is posting the result of an email inquiry to Roy Kerr.

Actually, there are other indications that tagsdad really is Roy Kerr!

 

[Chris Hillman]

In that case I nominate tagsdad for Science Advisor!

 

[Chris Hillman]

Bell spaceship "paradox" again, oh nooooo!

Here we go again: in "Quick question - has length contraction actually been experimentally confirmed?"

www.physicsforums.com/showthread.php?t=425566&page=2

There is the thought demonstation, first presented by Dewan and Beran, later retold by John Stewart Bell, usually referred to as 'Bell's spaceship paradox'. Two spaceships, connected by an unstretchable tether of length L, tether fully extended, are initially comoving. They synchronize their clocks. At an agreed point in time they commence acceleration, parallel to the tether, both accelerating at exactly the same G-count. For the tether to not break it would have to decrease the separation between the spaceships. However, since the spaceships meticulously maintain the same G-count the tether will snap.

There is only one physical factor that the breaking of the tether can be attributed to: length contraction.

The tether does break, but of course the "physical cause" is that the Bell congruence has nonzero expansion tensor (compare the Rindler congruence). IOW, the tether breaks because nearby Bell observers are moving away from each other. Notice that the expansion tensor approach neatly avoids the issue of "distance in the large": physically, the fact that nearby particles in the tether are moving away from each other means that at some place where the tether has a mechanical flaw, it will snap.

 

[Chris Hillman]

The Term "Locally Flat"

In the mis-titled thread "No globally flat geometry on S²" the OP is asking a question about the topology of the sphere. Unfortunately, User:lavinia just said in

www.physicsforums.com/showthread.php?p=2868002#post2868002

Globally flat means that the curvature tensor is identically zero.

No, a Lorentzian or Riemannian manifold (M,g) is said to be locally flat if the Riemann tensor vanishes. That implies that in a sufficiently small local neighborhood of any point, we can introduce a Cartesian chart and in our neighborhood, the geometry will mimic that of a flat space (or spacetime). But globally the topology could be nontrivial; consider the case of the cylinder [itex]R \times S^1[/itex]. Its Riemann tensor has only one algebraically independent component, which vanishes. Yet its topology is nontrivial.

 

[Chris Hillman]

BRS: Two questions concerning models constructed via "matching"

In "GR Vacuum solutions",

www.physicsforums.com/showthread.php?t=427333

TrickyDicky asks about vacuum solutions

when applying the solution to the Mercury precession problem or the bending of light by the sun problem, we are actually introducing the mass of the sun to solve them, and this to me seems a bit contradictory with the premise that there is no matter in the manifold under consideration.

Boundary conditions, just like solving any "field equation" for a source-free solution. No source term in the interior of the domain, but a source must be assumed on boundary to get a nontrivial solution.

Tom.Stoer replied to TrickyDicky by saying:

The Schwarzschild solution has two patches matching at the Schwarzschild radius. The outer solution is the familiar vacuum solution, whereas there is an inner solution which is NOT a vacuum metric and which therefore differs from the well-known inner vacuum solution used for a black hole. Instead a spherical symmetric, non-rotating, incompressible fluid is used which leads to a regular solution w/o singularity at r=0.

That is not quite correct.

Rather, the Schwarzschild stellar model is constructed by matching a perfect fluid interior (with constant density, so "incompressible" fluid, but nonconstant pressure falling to zero at some [itex]r0 > 2m[/itex], in fact [itex]r_0 > 9/8 \cdot 2m[/itex]--- see the discussion of Buchdahl's theorem in the textbook by Schutz) to a region of the exterior sheet of Schwarzschild vacuum, where the matching is across the world sheet of the round sphere at r=r0 (the zero pressure surface). The result is indeed a static spacetime with no curvature singularities anywhere, and with a true "center" in the fluid ball, at r=0 (where pressure is maximal).

However, it seems to me that Tom.Stoer was trying to address the issue raised by TrickyDicky, by pointing out that this suggests that the mass parameter in the exterior solution arises from imposing a physically appropriate boundary condition. Astrophysical black holes are formed by the collapse of ordinary matter, which we can idealize as an OS collapsing dust ball, constructing using a similar matching construction, in which again we have a regular center inside the dust ball, right up the moment when the dust ball collapses to a strong spacelike singularity inside the interior region of the newly formed black hole. See the BRS thread on "Conformal Compactifications" for how the causal structure of the OS model differs from the "eternal black hole".

In "GR Dust Cloud"

www.physicsforums.com/showthread.php?t=427311

Austin0 asks (murkily) about essentially the same construction; the answer is that in the exterior region of a model constructed by matching either a (dynamical) collapsing dust ball interior region or static spherically symmetric perfect fluid ball interior region to a static asymptotically flat Schwarzschild vacuum exterior region, the mass parameter in the exterior is the mass of the dynamical collapsing dust ball or static fluid ball respectively. The exterior region doesn't care which.

[EDIT: Heh, Dalespam already made this point while I was composing this post... ]

In general there is a problem with comparing the geometry of two locally nonisometric spacetimes both using a "radial coordinate" labeled r. Typically it is not so easy to compare "what happens at radius r=10m" between two such solutions, or even to compare the physical meaning of the parameter m. But that's probably too sophisticated for Austin0 right now.

 

[Dale]

But that's probably too sophisticated for Austin0 right now.

Definitely, but he is learning so he may get to that point sometime reasonably soon.

 

[Chris Hillman]

BRS: Survey of Conservation Laws in GTR?

In

www.physicsforums.com/showthread.php?t=426479

Ben Crowell asks for a review on the status of conservation laws in gtr, specifically:

What I'm interested in here is general conservation laws that would be valid in any spacetime, not the kind of conservation laws that hold for test particles in a spacetime with some special symmetry expressed by a Killing vector.

Oh boy! This turns out to be a big question which has been intensively studied for nearly one century, since no-one (either mainstreamers or dissident fringe figures) is happy with results known for a long time which show that naive notions of conservation laws (e.g. based upon Gauss's law) won't work in curved manifolds. As a result we have

• some really good ideas which are however at present technically and conceptually difficult to use
• some really bad ideas from fringe figures (often well known for being involved in every controversy they can find)
• some old but still useful ideas which sometimes work for limited purposes in limited circumstances
• nothing noncranky which is fully general, other than
• Noether "charges" from the analysis of variational symmetries in a Lagrangian formulation of a PDE (or Hamiltonian formulation of the geodesic equations, but Ben expressly ruled out anything depending on Killing vector fields)

I should also point out that several researchers appear to have been driven round the bend by trying too hard to do the impossible in this area, so I recommend starting out with the limited ambition of understanding better some of the ideas which have been explored so far, rather than turning gtr on its head.

Before I say anything else, in case some SA/M with little background in gtr, but who appreciates the utility of conservation laws, Gauss's law, etc. in elementary mathematical physics, is intrigued by the news that Gauss's law doesn't work too well in gtr, the canonical nontechnical reference is the UseNet Physics FAQ at Chez Baez:

math.ucr.edu/home/baez/physics/Relativity/GR/energy_gr.html

Is Energy Conserved in General Relativity?

In special cases, yes. In general -- it depends on what you mean by "energy", and what you mean by "conserved".

The remainder of this post is addressed to those who have studied at least one modern gtr textbook.

First, AFAIK, there is no up-to-date comprehensive survey of the status of conservation laws in gtr. The subject is apparently far to massive for that, no pun intended.

Fool that I am, I will attempt to provide, if not a survey, at least a semi-annotated list of suggested further reading.

I think it is important to go way back and begin with an old review paper, since anything published later will probably assume the reader has read this review (or else it was probably written by an ignoramus):

J. N. Goldberg,
Conservation Laws in General Relativity
chapter in Gravitation: An Introduction to Current Research, ed. by Louis Witten, Wiley 1962.

That is an invaluable book much too hard to obtain from "downsized" university libraries, waanhnh!--- it also contains several other must-read review papers from the early days of the Golden Age of Relativity, including the review of exact solutions by Ehlers and Kundt, a review of ADM, and more. If you can't find it at your uni, ask Los Alamos, they may have the last surviving copy.

Next, several textbooks such as MTW and Carroll have excellent discussions of conservation laws. See in particular Carroll or Hawking & Ellis for a differential law (generally valid in gtr) which explains how Ricci curvature can generate Weyl curvature. As Carroll explains, the well known law [itex]{T^{ab}}_{;b} = 0[/itex], can easily be mistaken for a "conservation law", but that is not the role it plays in gtr, despite its obvious similarity to [itex]{T^{ab}}_{,b} = 0[/itex] in flat spacetime.

The failure of conservation laws like [itex]{T^{ab}}_{,b} = 0[/itex] to make sense in gtr was known to Einstein and other early investigators, and it bugged the heck out of them, as you would imagine. And it still bugs the heck out of most modern researchers.

Here are some of the ideas which have been explored (neccessarily, there is some overlap in the following list):

• Pseudotensors: Einstein and several others came up with various versions of gravitational energy-momentum pseudotensors, which we can think of as a "virtual additional term" in the energy-momentum tensor, a term which purports to track the location and tranfser of the energy and momentum of the gravitational field. They are called pseudotensors because they behave like tensors under some subgroup of the full diffeomorphism group. These pseudotensors fell into disfavor many times as various failings came to light. For example: about 15 years ago some researchers started playing with GRTensorII and found that pseudotensors give hugely inconsistent results for most the familiar simple exact solutions. Despite this, right now they are again being promoted by some researchers.
  
  With suitable caution, you can try
  
  arxiv.org/abs/hep-th/0308070
  The Energy-Momentum Problem in General Relativity
  S. S. Xulu
  

  Energy-momentum is an important conserved quantity whose definition has been a focus of many investigations in general relativity. Unfortunately, there is still no generally accepted definition of energy and momentum in general relativity...quasi-local masses have their inadequacies...in this work we use energy-momentum complexes to obtain the energy distributions in various space-times.
  
  We elaborate on the problem of energy localization in general relativity and use energy-momentum prescriptions of Einstein, Landau and Lifgarbagez, Papapetrou, Weinberg, and M{\o}ller ... The Cooperstock hypothesis for energy localization in GR is also supported.

  (Note that claims for pseudotensors are IMO often overstated, and the suggestion of Cooperstock is quite controversial.)
• Superenergy tensors: these include the well known Bel-Robinson "superenergy tensor", which is a fourth-rank tensor with strong symmetry properties. I don't know of any extensive review, but try
  
  arxiv.org/abs/gr-qc/9912050
  Applications of Super-Energy Tensors
  J. M. M. Senovilla
  

  Abstract: In this contribution I intend to give a summary of the new relevant results obtained by using the general superenergy tensors. After a quick review of the definition and properties of these tensors, several of their mathematical and physical applications are presented. In particular, their interest and usefulness is mentioned or explicitly analyzed in 1) the study of causal propagation of general fields; 2) the existence of an infinite number of conserved quantities in Ricci-flat spacetimes; 3) the different gravitational theories, such as Einstein's General Relativity or, say, $n=11$ supergravity; 4) the appearance of some scalars possibly related to entropy or quality factors; 5) the possibility of superenergy exchange between different physical fields and the appearance of mixed conserved currents.

• Just as important as conserved quantitites are fluxes which track nonconservation, so to speak!
  • Komar mass and momentum are defined in terms of Killing vectors for stationary asympotically flat spacetimes, which often arise models of an "isolated gravitating system" (Ben ruled Killing vectors out, but I think we should rule them back in). One of the most important achievements in classical gtr to date has been the introduction of Bondi mass and momentum, which generalize these to nonstationary situations, and allow us to track globally the energy and momentum carried off to conformal infinity by gravitational radiation (and other massless radiation) from an isolated system.
  • In addition, Killing vector fields and their generalizations give rise to various notions of conserved currents, and you can look for terms like Bach currents. Random example:
    
    arxiv.org/abs/astro-ph/0007046
    Riemannian collineations in General Relativity and in Einstein-Cartan cosmology
    L.C.Garcia de Andrade
    

    Riemannian vectorial collineations along with current Killing conservation are shown to lead to tensorial collineations for the energy-stress tensor in general relativity and in Einstein-Cartan Weyssenhoff fluid cosmology.

  • In addition, for any system of possibly nonlinear PDEs arising as the Euler-Lagrange equations of some Lagrangian (for example, the Ernst equation, whose stationary axisymmetric case gives rise to the Ernst family of all stationary axisymmetric vacuum solutions in gtr), variational symmetries give rise to Noether currents and Noether charges (e.g. you might obtain "for free" a conserved quantity analogous to the energy contained in a solitonic wave).
• Also important are some other quantities, defined in special classes of spacetime models, whose unexpected behavior signals further phenomena to be aware of: in paticular the Misner-Sharp mass appears naturally in various spherically symmetric models, and plays a role in the Israel-Poisson notion of "mass inflation" in the interior of black hole (with some infalling massless radiation). Compare and contrast some remarks I hinted at in the BRS on Weyl vacuums, where I sketchily indicated some related ways in which "mass" behaves unlike Newtonian intuition in gtr.
• Quasilocal notions of energy and momentum: currently these seem to offer the closest approach to what Ben wants; for a review see
  
  relativity.livingreviews.org/Articles/lrr-2009-4/
  Quasi-Local Energy-Momentum and Angular Momentum in General Relativity
  László B. Szabados
  See also
  
  arxiv.org/abs/gr-qc/0004074
  Quasi-Local Conservation Equations in General Relativity
  J.H. Yoon
  

  A set of exact quasi-local conservation equations is derived from the Einstein's equations using the first-order Kaluza-Klein formalism of general relativity in the (2,2)-splitting of 4-dimensional spacetime. These equations are interpreted as quasi-local energy, momentum, and angular momentum conservation equations. In the asymptotic region of asymptotically flat spacetimes, it is shown that the quasi-local energy and energy-flux integral reduce to the Bondi energy and energy-flux, respectively. In spherically symmetric spacetimes, the quasi-local energy becomes the Misner-Sharp energy. Moreover, on the event horizon of a general dynamical black hole, the quasi-local energy conservation equation coincides with the conservation equation studied by Thorne {\it et al}. We discuss the remaining quasi-local conservation equations briefly.

• Another venerable approach seeks "conservation laws" appropriate for working within the ADM formalism and other initial-value approaches to gtr; see for example
  
  arxiv.org/abs/gr-qc/0003019
  Noether Charges, Brown-York Quasilocal Energy and Related Topics
  L. Fatibene, M. Ferraris, M. Francaviglia, and M. Raiteri
  

  The Lagrangian proposed by York et al. and the covariant first order Lagrangian for General Relativity are introduced to deal with the (vacuum) gravitational field on a reference background. The two Lagrangians are compared and we show that the first one can be obtained from the latter under suitable hypotheses. The induced variational principles are also compared and discussed. A conditioned correspondence among Noether conserved quantities, quasilocal energy and the standard Hamiltonian obtained by 3+1 decomposition is also established. As a result, it turns out that the covariant first order Lagrangian is better suited whenever a reference background field has to be taken into account, as it is commonly accepted when dealing with conserved quantities in non-asymptotically flat spacetimes. As a further advantage of the use of a covariant first order Lagrangian, we show that all the quantities computed are manifestly covariant, as it is appropriate in General Relativity.

• Even the mass/momentum of test particles can be problematic; for a review see
  
  relativity.livingreviews.org/Articles/lrr-2004-6/
  The Motion of Point Particles in Curved Spacetime
  Eric Poisson
  Things get worse when one tries to go to the next approximation and consider objects which are small but not that small. In particular, one can search for "conserved quantities" in post-Newtonian formalism; see
  
  arxiv.org/abs/gr-qc/9503041
  Conservation laws for systems of extended bodies in the first post-Newtonian approximation.
  Thibault Damour, David Vokrouhlicky
  

  The general form of the global conservation laws for $N$-body systems in the first post-Newtonian approximation of general relativity is considered. Our approach applies to the motion of an isolated system of $N$ arbitrarily composed and shaped, weakly self-gravitating, rotating, deformable bodies and uses a framework recently introduced by Damour, Soffel and Xu (DSX). We succeed in showing that seven of the first integrals of the system (total mass-energy, total dipole mass moment and total linear momentum) can be broken up into a sum of contributions which can be entirely expressed in terms of the basic quantities entering the DSX framework: namely, the relativistic individual multipole moments of the bodies, the relativistic tidal moments experienced by each body, and the positions and orientations with respect to the global coordinate system of the local reference frames attached to each body. On the other hand, the total angular momentum of the system does not seem to be expressible in such a form due to the unavoidable presence of irreducible nonlinear gravitational effects.

• Another idea: consider asymptotically flat spacetimes and models of "isolated systems", see
  
  arxiv.org/abs/0906.2155
  Null Geodesic Congruences, Asymptotically Flat Space-Times and Their Physical Interpretation
  T. M. Adamo, C.N. Kozameh, E.T. Newman
  (This paper offers an excellent discussion of conservation of Bondi mass and angular momentum of isolated systems, taking account of that lost to radiation carrying away mass and momentum to conformal infinity.)
  
  arxiv.org/abs/0802.3314
  On Extracting Physical Content from Asymptotically Flat Space-Time Metrics
  C. Kozameh, E. T. Newman, G. Silva-Ortigoza
  Next, consider quasigroups which behave nicely "at conformal infinity"; see
  
  arxiv.org/abs/gr-qc/0403044
  Quasigroups, Asymptotic Symmetries and Conservation Laws in General Relativity
  Alexander I. Nesterov
  

  A new quasigroup approach to conservation laws in general relativity is applied to study asymptotically flat at future null infinity spacetime. The infinite-parametric Newman-Unti group of asymptotic symmetries is reduced to the Poincar\'e quasigroup and the Noether charge associated with any element of the Poincar\'e quasialgebra is defined. The integral conserved quantities of energy-momentum and angular momentum are linear on generators of Poincar\'e quasigroup, free of the supertranslation ambiguity, posess the flux and identically equal to zero in Minkowski spacetime.

• Another idea: formulate a general theory of conservation laws for classical field theories; examples include:
  
  arxiv.org/abs/gr-qc/9911095
  A General Definition of "Conserved Quantities" in General Relativity and Other Theories of Gravity
  Robert M. Wald and Andreas Zoupas
  

  In general relativity, the notion of mass and other conserved quantities at spatial infinity can be defined in a natural way via the Hamiltonian framework: Each conserved quantity is associated with an asymptotic symmetry and the value of the conserved quantity is defined to be the value of the Hamiltonian which generates the canonical transformation on phase space corresponding to this symmetry. However, such an approach cannot be employed to define `conserved quantities' in a situation where symplectic current can be radiated away (such as occurs at null infinity in general relativity) because there does not, in general, exist a Hamiltonian which generates the given asymptotic symmetry. (This fact is closely related to the fact that the desired `conserved quantities' are not, in general, conserved!) In this paper we give a prescription for defining `conserved quantities' by proposing a modification of the equation that must be satisfied by a Hamiltonian. Our prescription is a very general one, and is applicable to a very general class of asymptotic conditions in arbitrary diffeomorphism covariant theories of gravity derivable from a Lagrangian, although we have not investigated existence and uniqueness issues in the most general contexts. In the case of general relativity with the standard asymptotic conditions at null infinity, our prescription agrees with the one proposed by Dray and Streubel from entirely different considerations.

  
  
  arxiv.org/abs/hep-th/9608008
  Asymptotic conservation laws in field theory
  Authors: I. M. Anderson, C. G. Torre (Utah State University)
  (Submitted on 1 Aug 1996 (v1), last revised 14 Oct 1996 (this version, v2))
  

  A new, general, field theoretic approach to the derivation of asymptotic conservation laws is presented. In this approach asymptotic conservation laws are constructed directly from the field equations according to a universal prescription which does not rely upon the existence of Noether identities or any Lagrangian or Hamiltonian formalisms. The resulting general expressions of the conservation laws enjoy important invariance properties and synthesize all known asymptotic conservation laws, such as the ADM energy in general relativity.

• Another idea: look for "conserved quantities" in the well-known GEM formulation of linearized gtr; try
  
  arxiv.org/abs/gr-qc/0311030
  Gravitoelectromagnetism: A Brief Review
  Bahram Mashhoon
  

  The main theoretical aspects of gravitoelectromagnetism ("GEM") are presented. Two basic approaches to this subject are described and the role of the gravitational Larmor theorem is emphasized. Some of the consequences of GEM are briefly mentioned.

• Another class of ideas: reformulate gtr in such a way as to remove the mathematical problems entirely. Or rather, formulate an arbitrarily good mimic of gtr which allows one to work in a convenient way with conserved quantities, including a conserved notion of the energy/momentum in the gravitational field itself. One example is the work of Itin on teleparallel gravity. See
  also
  
  arxiv.org/abs/0905.4026
  Conservation of Energy-Momentum in Teleparallel Gravity
  Mariano Hermida de La Rica
• Last but not least, one can formulate a completely new classical gravitation theory which is constructed to ensure that conservation laws can be formulated and proven; the list of candidates which has been proposed (but in almost every case, too little studied) is far too long for me to even think about attempting to itemize them here!

I should stress that scientific controversies (and alas, a generous dollop of pseudoscientific suggestions) are impossible to avoid in such a huge topic, and I am not neccessarily endorsing anything the authors of the above papers say; I am simply trying to briefly indicate the depth and breadth of the work which has been done so far.

 

[Chris Hillman]

"News" from National Geographic, sort of

It seems that a reporter recently attended an astronomy conference and wrote an article for National Geographic recounting a number of things she heard.

In "Rogue black holes?!"

www.physicsforums.com/showthread.php?p=2873886#post2873886

Astronuc asked

Astronomers have long known about rogue black holes?

Yup, for a long time. But not to worry, the galaxy is so large that Earth isn't likely to encounter one in the forseeable future.

How long?

Redmount and Rees 1989 discussed possible recoil effects due to asymmetric emission of gravitational waves during the merger of two black holes, and I believe there are even earlier discussions. So the possibility has been discussed for at least two decades, and during the last decade there have been an increasing number of numerical simulations plus observations supporting the idea that rogue black holes are common.

Rees discussed possible recoil effects in his 1998 review of "Astrophysical Evidence for Black Holes", published as a chapter in the Chandrasekhar symposium at the U of C, and its been well known for a long time in the field.

Kelly Holley-Bockelmann, of Tennessee's Vanderbilt University, and her colleagues were the first to show that the objects could arise from violent mergers.

Using a computer model, Holley-Bockelmann found that two combining black holes rotating at different speeds or of different sizes give the newly merged black hole a big kick.

I don't see anything new or surprising here. All of these features have been discussed previously.

[Gravitational wave recoil effects during a merger] sends the object hurtling in an arbitrary direction at velocities as high as 2,485 miles (4,000 kilometers) per second.

Not arbitrary; the direction of kick is likely to align roughly with the orbital phase at the moment of merge, and there may also be a component orthogonal to the plane of coalescence due to spin-spin interaction (a strong field gravitomagnetism phenomenon).

"This is much higher than anyone predicted," Holley-Bockelmann said. "Even the average kick velocity of 200 kilometers [124 miles] per second is extremely high."

This claim is AFAIK a few years old.

The National Geo article also discusses new estimates of the number of intermediate mass black holes in our galaxy, but again, it has been known for some years that these are after all not uncommon objects.

Is this a new revelation about DM and BHs?

Not the stuff I discussed above. I suspect this is the predictable result of a science reporter attending a meeting, hearing something in a talk which was spun as "new", and not checking with experienced researchers before writing a story.

More often such misinformation comes straight from PR flacks attempting to portray mundane faculty research as a "revolutionary advance"; as everyone here probably knows, many news outlets reprints such press releases verbatim without any pretense of fact checking. In this case, the reporter put her name on the piece so she may be culpable--- especially since it is impossible to think of a more convenient venue for a science reporter to ask some senior people in the field to comment/explain than at a major conference.

The apparent (indirect) observation of what is inferred to be "dark matter" in an accretion disk, now--- that really is new, AFAIK:

They also found hints that dark matter may play an important role in the hot disks that form around companion-consuming black holes.

 

[Chris Hillman]

Shannon entropy, popularity of normal distributions, and Ehrenfest paradox ad nauseum

In "Entropy of a product of positive numbers"

www.physicsforums.com/showthread.php?t=428040

clamshell asks a question about Shannon entropy which doesn't really make sense

I accept that the entropy (Shannon's) of a sum of positive
numbers is the sum of the -P_n * LOG(P_n) for each number
in the sum of numbers where P_n is the average contribution
of a term. IE, if T = A + B, then
S = -(A/T)*LOG(A/T) - (B/T)*LOG(B/T).
But what about the entropy of A*B?

Please don't respond with "why do it?", just give me some idea of how you might attempt to do it.

The Boltzmann entropy can be defined for any partition of some finite set into r blocks [itex]X = \union_{j=1}^r A_r[/itex]. It is simply the logarithm (any base you like) of the multinomial coefficient
[tex]
H (\pi) =
\log \,
\left( \begin{array}{ccccc}
& & n & & \\
n_1 & n_2 & \ldots & n_{r-1} & n_r
\end{array} \right)
[/tex]
where
[tex]
|X| = n, \; |A_j| = n_j
[/tex]
If we put p_j = n_j/n we obtain a probability measure on the partition, and applying Stirling's approximation under the assumption that all the blocks are large (i.e. all the n_j are large), we obtain the approximation
[tex]
H(\pi) \approx -\sum_{j=1}^r p_j \log p_j
[/tex]
We can define this expression to the Shannon entropy [itex]H_p(\pi)[/itex] of a finite partition of any set X endowed with a probability measure p (where at first one should probably think of varying the partition while keeping p fixed). Notice that the blocks need no longer be finite sets, and that both Boltzmann and Shannon entropies characterize the "variety" of the finite partition. The first is a purely combinatorial notion while the second is a probabilistic notion.

More generally, if we have measure-preserving transformation g on X, with a bit more work we can define the Shannon entropy of the transformation g, which is the definition used in ergodic theory. Shannon himself used this definition for Markov chains, and he showed that his entropy satisfies a number of very important formal properties which justify its interpretation as a measure of "the variety of alternatives", the interpretation Shannon stressed in his founding paper, Shannon 1948. The most important of these formal properties states that if you refine some partition, you can compute the new entropy by taking a weighted average of the entropies of the blocks and adding this to the original entropy. In terms of physics, this corresponds to the fact that refining a partition increases the Boltzmann entropy, which is traditionally interpreted in terms of "fine-graining your macrostates". Put in other words, if you can identify a refined classification of microstates into macrostates, your uncertainty concerning which macrostate an unknown microstate belongs to is larger.

Typical questions answered in the theory of entropy include: "if I learn that the unknown state of the system lies in a particular block of one partition, how much information do I gain about which block of another partition it lies in"? See the BRS thread "Exploring the Rubik Cube with GAP"

www.physicsforums.com/showthread.php?t=422410

for a group theoretic interpretation and generalization of Boltzmann entropy. Here, the "alternatives" in question are alternative "motions" under some group action, say a group of transformations of X, and we can ask questions such as these: "if we learn the motion of one subset A under an unknown transformation on X, how much information do we gain about the motion of another subset B?"

Clamshell in effect considers a partition into only two blocks and assigns one of them an arbitrary probability p where 0 < p <1, so that the other block has probability 1-p. Then of course the Shannon entropy of this partition into two blocks is simply
[tex]
H(\pi) = -p \log p - (1-p) \log (1-p)
[/tex]
Then he asks about the entropy of the "product" of the two blocks! That simply doesn't make sense. Probably clamshell is trying to ask about the refinement property but is getting himself confused.

One can certainly try to "categorify" these notions of entropy, and John Baez and myself have discussed that in various internet posts.

BTW, re the remarks of marcus in

www.physicsforums.com/showthread.php?p=2876424#post2876424

Shannon entropy in ergodic dynamics is simply the Hausdorff dimension of a certain fractal set, and the group action generalization of Boltzmann entropy is also a generalization of the notion of "degrees of freedom".

Re

www.physicsforums.com/showthread.php?t=427247

striphe (whose user name is unpleasantly suggestive and who has apparently been posting quite a bit of fringe stuff) asked about mean and standard deviation.

FWIW, the emphasis in elementary statistics on mean and standard deviation is mainly due to Gauss in the early 19th century and Fisher in the early 20th century, and as both researchers knew, the reason why these are so convenient is that variance is essentially euclidean geometry in disguise (the Pythagorean theorem). This ensures that variance is easy to compute and work with, and obeys many very simple laws which can be understood in simple geometric terms. See for example M. G. Kendall, A Course in the Geometry of n Dimensions, Dover reprint, for a short book which stresses this fact. In addition, as Chebyshev showed later in the 19th century, variance remains useful even for general (non-normally distributed) "random variables", although of course many theorems fail in general. These and other considerations led Fisher to develop an extensive theory of estimators suitable for deciding which probability measure belonging to some family of measures (typically defined by some formula giving the "density" in terms of a finite list of parameters) best fits given data.

In contrast, while "nonparametric statistics" such as estimators of Shannon entropy have in some sense better justified interpretations, due to the work of Shannon and numerous subsequent researchers such as Kullback, and may enjoy powerful formal properties of their own, and may even support an abstract notion of (highly non-Riemannian) geometry permitting a "geometric intrepretation" of the statistic, there are typically practical problems with using such estimators--- and even legal problems, due to the absurd legal requirements in the U.S. that federally funded medical researchers use only "unbiased estimators", a requirement which more or less ensures bad decisions when working with nonlinear statistics.

Re

www.physicsforums.com/showthread.php?t=428147

I am sooo sick of watching people make silly mistakes while trying to discuss the so-called "Ehrenfest paradox" (which was completely resolved by Langevin c. 1927 in the manner expected by Einstein, using a frame field). Just one which has already appeared: you cannot spin up a rigid body in str. If it is already spinning, it is possible that its component particles might be in Born rigid rotation, but if you try to alter that state of motion, it cannot remain rigid. This makes it nontrivial to compare alleged "identical disks", one spinning and one not! Also, almost everyone confuses submanifolds with quotient manifolds, which is disastrous here. The so-called "spatial geometry of the rigidly rotating disk" refers to a certain Riemannian manifold which arises as a quotient by the congruence of world lines of Langevin observers, not as a submanifold of Minkowski spacetime! As Einstein expected, the geometry of this manifold turns out to be very nearly hyperbolic near the center, with a curvature depending upon the rotation rate of the disk.

 

[Chris Hillman]

BRS: the Ehrenfest thread

That's right, with this post, which I hope won't hijack this running thread, this will be the third concurrently running thread in the BRS related to the so-called "Ehrenfest paradox" and "Bell paradox".

In the very long thread "Ehrenfest / rod thought experiment." (which I haven't been reading--- for reasons of bloodpressure, as someone put it!--- but which seems to actually be mostly more related to the "Bell paradox")

www.physicsforums.com/showthread.php?t=428147&page=5

Ben Crowell asked

Actually, is there a standard definition of a static observer? The books I have seem to define a clear notion of a static spacetime and of static coordinates used to describe a static spacetime (i.e., coordinates in which the metric is diagonal and time-independent). But defining a property of coordinates isn't quite the same as defining a property of an observer, since observers are local and coordinates are global.

Static spacetime (M,g): there exists a timelike vorticity-free (thus, hypersurface orthogonal) Killing vector field [itex]\xi[/itex]. Static observer: the ones whose world lines are integral curves of this Killing vector field.

Note: usually the Killing vector has to be renormalized to make a timelike unit vector field, which is then the tangent vector field to the (proper time parameterized) world lines of the static observers.

In the special case of homogeneous static spacetimes, we actually have many timelike Killing vector fields formed by various linear combinations of "translational" Killing vector fields with a timelike Killing vector field. But Schwarzschild vacuum (in particular) is only static, not homogeneous.

 

[Chris Hillman]

BRS: A very common misunderstanding of how science works

In "Are the foundations stable"

physicsforums.com/showthread.php?t=429435

EnergyLoop asks

We are constantly finding new information that does not fit the current model, but by adding a new constant or variable into the equations it repairs the problem, but gives us new things to look for such as dark matter and dark energy. I can’t help thinking about Aristotle’s crystalline spheres and the Earth centered universe, this was a simple concept initially, until the motion of the planets was realized, then it became a complex mathematical model to try to explain this motion, Copernicus then simplified the problem, and removed the complexity.
I hope we are not heading in that direction again, is the foundation sound, equations are build on previously established equations does anyone re-examine these?

This seems to have become a very common refrain, particularly since the recent discovery of an accelerating expansion. (Cosmologists quickly adjusted; the general public did not!) I'd actually like to see a PF FAQ somewhere which addresses this, mentioning some of the same points I've made so often in the past, including:

• All scientific knowledge is provisional. Constantly finding new information and constantly trying to fit new data or theoretical speculations into the body existing well-established theories is precisely what scientists do on a day-to-day basis. The fact that scientific theories can be tested by comparing quantitative predictions with quantitative data, and the fact that scientific theories are constantly up for revision is what makes science the most powerful tool known to man for the discovery and organization of knowledge about the natural world.
• Scientific knowledge consists of a vast body of experimental/observational data plus the terminology, notation, and theories we use to interpret the data. We make theories and we make predictions from theories using mathematical reasoning, and you need to know the appropriate mathematical background to understand the theories.
• When new data cannot be fit into existing theories, scientists look for explanations. First and foremost, a careful examination of the possibility that the data collection or analysis contained some subtle systematic bias or other flaw. If that fails, then one searches for the change to the theories which is "the least possible".
• The public seems to generally misunderstand the nature of scientific advances: they should be astonished not by how much has changed, but by how little, even in such an extreme revelation as the "accelerating Hubble expansion". That is, one benefit of knowing the data and the theories is that you can appreciate how "introducing nonzero Lambda" is actually the smallest possible change to the theories. Also, the data hasn't changed, only our interpretation. Making a minimal change means that only a very small portion of our interpretation/understanding of the universe changed as the result of that particular "cosmological revolution".
• To repeat: the public seems to generally misunderstand the nature of scientific revolutions. Newtonian gravitation was never "discarded", it is still used, and more often than gtr, which is a bit harder to work with. If and when a successful theory of quantum gravity appears, gtr will still be used (most likely) because the new theory will be (most likely) a bit harder to work with. Similar remarks hold for non-relativistic physics, and various specific theories which are known to be "wrong" but are still useful for limited purposes.

In "Dark Matter Distribution Around Galaxies"

www.physicsforums.com/showthread.php?t=429521

RLutz asks

Is there any reason why galactic black holes might have something to do with dark matter creation? The squashed beachball of dark matter sort of looks like what I would expect say field lines coming out of a pulsar to look like or something.

Ditto Chalnoth, plus a reminder that a black hole of mass M gravitates just like any star of mass M; unless you are very close to it you won't encounter the strong portions of the exterior field which result from the fact that the hole is so much more compact than the star.

In "Black Holes are Tears in Space"

www.physicsforums.com/showthread.php?t=429376

I`m not a scientist, i`m actually a 3D Artist. I just have a lot of faith in science unfortunately didn`t have the attention span to pay attention enough in high school and even more tragic is that my university didn`t offer any science courses!

Actually, that is tragic. Due to the global financial crisis, at least one state university in the U.S. has just eliminated its science majors.

Could it be possible that a black hole is a tear in space? It seems like it could be a way of explaining why some say you could travel through a black hole or worm hole and wind up somewhere else. If space itself was really in a shape we couldn't comprehend then maybe a tear in one place could wind up opening in an entirely different place. Does that make sense?

It's vaguely reminiscent of various possibilities discussed in serious physics like wormholes or curvature singularities, but much too vague to make much sense in a scientific discussion. So the best short answer is probably: a black hole is a region of spacetime characterized by the presence of an event horizon, which you can think of as an imaginary surface which you can fall through, but once you do, you can't ever emerge from behind the horizon, at least not into the same external region of spacetime in which you started. A very good book for poets which IMO can enable persons with only a high school science education to actually understand this, sort of, from (good!) pictures, is Geroch, General Relativity from A to B, University of Chicago Press.

In "Re: Big Bang and PreExisting Void?"

www.physicsforums.com/showthread.php?t=425597&page=4

JDoolin, whose "knowledge" of cosmological models is apparently based upon Wikipedia, not textbooks plus graduate level schoolwork, is arguing with Chalnoth and others about the Milne chart for the Minkowski vacuum, claiming that this chart is "inequivalent" to the cartesian chart. Of course that hinges on the meaning of "inequivalent"; gtr is however based upon Lorentzian geometry, and in Lorentzian geometry, any chart covering a region U in a spacetime (M,g) is just as good as any other.

In "velocity of gravity wave"

physicsforums.com/showthread.php?t=429166

spikenigma seems to think the European Gravity Field and Steady-State Ocean Circulation Explorer project has something to do with comparing the speed of gravitational and EM radiation! Needless to say, the investigators say something very different: from

www.esa.int/esaLP/ESAJJL1VMOC_LPgoce_0.html

GOCE will be gathering data or around 20 months to map the Earth's gravity field with unprecedented accuracy and spatial resolution. The final gravity map and model of the geoid will provide users worldwide with a well-defined data product that will lead to:

• A better understanding of the physics of the Earth's interior to gain new insights into the geodynamics associated with the lithosphere, mantle composition and rheology, uplift and subduction processes.
• A better understanding of the ocean currents and heat transport.
• A global height-reference system, to serve as a reference surface to study topographic processes and sea-level change.
• Better estimates of the thickness of polar ice-sheets and their movement.

• 
  One can use the next section to compute whether or not GOCE will be able to track ballistic missile submarines but in any case, it will be clear to anyone here, I think, that GOCE has nothing to do with "speed of gravity".

 

[Chris Hillman]

In "the arrow of time"

www.physicsforums.com/showthread.php?t=337236&page=11

A-wal expresses in a memorable way one key element of Simple Physics crackpottery:

You think I don’t understand the concepts just because I don’t know how to speak your language.
...
Would you watch your favorite dvd in binary code? The code is necessary but no one cares what it looks like.
...
I really don’t want to think like physicists do. I want to get this straight in my head and still think the way I do. I don’t know how you can do it like that.

Translation:

I refuse to learn to reason scientifically about scientific issues. But I insist upon exercising my constitutional right to make incontestable statements concerning scientific issues any time, any place. My way of muddled "thought" [sic] has just as much "validity" [sic] as all your hi-falutin equations. I don't need no stinkin' math!

He also offers a memorable anti black hole rant (based on willful ignorance, one might say):

I don’t mind if I’m wrong. My ego isn’t tied up in this and I have nothing to prove. I find it difficult to accept what I don’t understand and I’m not convinced by what I’ve been told. How the hell can an object that can never reach the horizon from any external perspective ever cross the horizon from its own perspective? Is not just the light from those objects that’s frozen. How could it be if they could always escape? They’re moving slower and slower through time relative to you because time in that region is moving slower and slower relative to you. If the time dilation/length contraction go up to infinity then no given time can ever long enough and no distance can ever be short enough locally if it’s infinitely length contracted from a distance! Are those inertial coordinates you use to describe an object crossing the event horizon even relative? Does it take into account the fact that you’re constantly heading into an ever increasingly sharpening curve?

 

[Chris Hillman]

Multiple misunderstandings of analysis of Hagihara observers

In

www.physicsforums.com/showthread.php?t=431407

several posters seem to be trying answer this question:

How does the tidal tensor (electroriemann tensor) distort a small sphere of test particles in circular orbit around an isolated massive object?

The easiest way to analyze this is to study an appropriate frame field associated with the Hagihara observers, who move in circular orbits in planes parallel to the equatorial plane, where we are of course in the exterior region of the Schwarzschild vacuum. (We can ask the same question and proceed the same way for suitable observers in the Kerr vacuum, but that situation is more complicated in several ways.)

The good news is that some participants appear to be trying to learn about Hagihara observers. The bad news is that they are reading a Wikipedia article (I myself wrote an early version of that particular article, but we should presume that the current version has been trashed by years of edits by persons who didn't know what they were doing, or didn't care) instead of good textbooks. Thus, everyone participating in that thread (as of 23 September 2010) is badly confused on many many points, e.g.

• "frame": in the WP article, frame means frame field, not "local coordinate chart" or whatever these posters are trying to do to apply the EP on a curved spacetime over a local neighborhood rather than in the tangent space to a single event,
• none of the participants appear to understand the distinction between components wrt a frame field and components wrt a coordinate basis,
• the WP article Lut Mentz cites gives frame field components, not coordinate basis components,
• acceleration vector, expansion scalar, shear tensor, and vorticity tensor all refer to a timelike congruence (not neccessarily geodesic, although many books make that assumption for simplicity, and the Hagihara observers are geodesic observers in the equatorial plane),
• the timelike unit vector in a frame field defines a timelike congruence, but the frame field also involves three unit spacelike vector fields, with all four being mutually orthogonal at each event,
• the posters appear to be confusing a congruence having nonzero vorticity tensor with a spinning frame field,
• the Hagihara observers are only geodesic observers in the equatorial plane; their world lines correspond to circular orbits parallel to the equatorial plane so obviously most of these require some acceleration to exist,
• to quote the components of a tensor, it helps to adopt a frame and to quote the components wrt the frame, but note that one naturally derives first a spinning frame in which [itex]\vec{e}_2[/itex] points radially outward, and only later finds the nonspinning frame,
• small spheres of Haghihara observers in the equatorial plane shear as they orbit, but don't change volume (vanishing expansion scalar!),
• if one adopts the nonspinning frame, then Lense-Thirring precession shows up (in fact you can derive an exact version of the Lense-Thirring precession formula using this approach!); the nonspinning frame of the Hagihara observers slowly spins wrt the frame of a distant star, so to speak.

For what it is worth, you can derive a frame field adapted to Hagihara observers by starting with the frame of static observers
[tex]
\begin{array}{rcl}
\vec{e}_1 & = & \frac{1}{\sqrt{1-2m/r}} \; \partial_t \\
\vec{e}_2 & = & \sqrt{1-2m/r} \; \partial_r \\
\vec{e}_3 & = & \frac{1}{r} \; \partial_\theta \\
\vec{4}_4 & = & \frac{1}{r \sin(\theta)} \; \phi
\end{array}
[/tex]
in the [itex]\vec{e}_4[/itex] direction, at each event, by an undetermined boost, with the boost parameter depending only on r. Then require that the acceleration of the new frame vanish in the equatorial plane. This gives an ODE for the boost parameter as a function of r which can easily be solved. The result is
[tex]
\begin{array}{rcl}
\vec{f}_1 & = & \frac{1}{\sqrt{1-3m/r}} \;
\left( \partial_t + \sqrt{\frac{m}{r}} \;
\frac{1}{r \, \sin(\theta)} \; \partial_\phi \right) \\
\vec{f}_2 & = & \sqrt{1-2m/r} \; \partial_r \\
\vec{f}_3 & = & \frac{1}{r} \; \partial_\theta \\
\vec{f}_4 & = & \frac{\sqrt{1-2m/r}}{\sqrt{1-3m/r}} \;
\left( \sqrt{\frac{m}{r}} \; \partial_t +
\frac{1}{r \, \sin(\theta)} \; \partial_\phi \right)
\end{array}
[/tex]
(Recall that the static frame and the chart are only defined on r > 2m; notice that the Hagihara frame is only defined on r>3m, at best!) Then by construction
[tex]
\nabla_{\vec{f}_1} \vec{f}_1 = 0
[/tex]
in the equatorial plane (off this plane, the acceleration is nonzero!), the expansion scalar vanishes, the only independent nonzero component of the shear tensor is
[tex]
\sigma_{24} = \frac{-3}{4} \sqrt{\frac{m}{r^3}} \;
\frac{1-2m/r}{1-3m/r}
[/tex]
while the only independent nonzero component of the vorticity tensor is
[tex]
\omega_{24} = \frac{-1}{4} \sqrt{\frac{m}{r^3}} \;
\frac{1-6m/r}{1-3m/r}
[/tex]
The tidal tensor is
[tex]
\begin{array}{rcl}
E_{22} & = & \frac{-2m}{r^3} \; \frac{1-3m/2/r}{1-3m/r}
\approx \frac{-2m}{r^3} \; \left( 1-\frac{3m}{2r} \right) \\
E_{33} & = & \frac{m/r^3}{1-3m/r}
\approx \frac{m}{r^3} \; \left( 1 + \frac{3m}{r} \right) \\
E_{44} & = & \frac{m}{r^3}
\end{array}
[/tex]
All these expressions are refer to the frame [itex]\vec{f}_1, \ldots \vec{f}_4[/itex], and are only valid in the equatorial plane. And the acceleration vector, expansion scalar, shear tensor, and vorticity tensor all refer to the timelike unit vector field [itex]\vec{f}_1[/itex], whose integral curves are the world lines of the Hagihara observers.

So small spheres of inertial observers orbiting very nearly in the equatorial plane are sheared parallel to that plane, but maintain constant volume.

To study precession, you should introduce an undetermined secular rotation about [itex]\vec{f}_3[/itex], with the rate of rotation depending only on r, and demand that the Fermi derivatives of [itex]\vec{f}_2, \ldots \vec{f}_4[/itex] with respect to [itex]\vec{f}_1[/itex] should vanish in the equatorial plane. This gives an ODE for the rotation rate which you can solve, finding that the cumulative angle of rotation (at each event along the world line of a equatorial Hagihara observer) is
[tex]
\psi = t \, \sqrt{\frac{m}{r^3}} \; \sqrt{1- 3 m/r}
[/tex]
(The Lense-Thirring precession formula given in many textbooks is only an approximation of the exact result.)

With respect to the new, nonspinning frame, all the tensors just mentioned have different components from what we found for the first frame, in which the "principle axes" are seen to very slowly spin wrt the spatial vectors of the nonspinning frame. But the tensors are defined in terms of [itex]\vec{f}_1[/itex] which is shared by the two frames, and they are three dimensional tensors, so their traces and quadratic invariants (and higher order invariants) will neccessarily agree regardless of which frame you compute the components in!

You can compare all this with the Lemaitre frame appropriate for studying the physical experience of observers falling in freely and radially. Their tidal tensor wrt the Lemaitre frame is [itex]m/r^3 \; \operatorname{diag}(-2,1,1)[/itex]. So:

A small sphere of test particles released from rest inside Fr. Lemaitre's spaceship elongates radially and compresses orthogonally, while keeping constant volume, so forming a prolate spheroid; if Fr. Lemaitre looks out the window, he can see that the long axis of the prolate spheroid is aligned parallel to the direction toward the massive object.

A small sphere of test particles released from rest inside Dr. Hagihara's spaceship behaves similarly, but compresses slightly more than expected orthogonally to the equatorial plane, and slightly less radially, so forming a triaxial ellipsoid. If he looks out the window, he can see that the long axis of this triaxial ellipsoid is parallel with the direction of the massive object. In addition, assuming Dr. Hagihara's spaceship is gyrostabilized, as he keeps returning to "1 January" in his orbit, over time he notices a very gradual precession wrt the distant stars of where he is in his orbit on 1 January by his clock, and he also observes his spaceship to be very very slowly spinning as it orbits.

The tidal and precession effects have very different characteristic time scales, however--- the participants in the thread in question have not yet recognized this.

If Dr. Hagihara looks out the window, he can see that his spaceship, which is gyrostabilized, is nevertheless very slowly spinning wrt the massive object, and also wrt "the distant fixed stars". Looking at neighboring spaceships (also inertial and initially motionless wrt him), he sees that they appear to be very slowly shearing orthogonal to the equatorial plane (because the ships further out are moving more slowly in their orbits, as per Kepler) and that their trajectories are slowly swirling about his ship in the sense of nonzero vorticity.

All of these things can be studied in various limits and are consistent with Newtonian expectation in a suitable slow motion weak gravity limit.

If I am somehow making this sound hard, that is not my intent. This is not hard. It does however involve learning several new concepts (new for most people, or at least not yet understood by most people) and one needs to keep all these things straight: coordinate charts, frame fields, congruences, tensors defined on spacetime independent of any congruence, effectively three-dimensional tensors defined wrt a specific congruence, kinematic decomposition of a congruence, components of a tensor, invariants of a tensor, when orthogonal hyperslices do and do not exist, etc.

In

www.physicsforums.com/showthread.php?t=431572

the OP appears to be enthused by the recent widely publicized claims of Nikodem J. Poplawski which are based on elementary misunderstandings as I explained elsewhere in the BRS, so the short answer there is that Poplawski doesn't yet understand the theory of Lorentzian manifolds sufficiently well to avoid mistakes, and his claims are based upon such mistakes. It is possible that the OP might also be vaguely referring to notions of "baby universes" being born inside black holes, which is an intriguing speculative suggestion, but not one which is currently very well supported even by theoretical arguments, and of course not at all by observation!

 

[Chris Hillman]

Gravity shielding: how to

In "e.p. implies no gravitational shielding?; Feynman?"

www.physicsforums.com/showthread.php?t=432327

Ben Crowell asks how/why gtr forbids gravitational shielding. I haven't yet had a chance to read that thread, but it reminds me of an amusing observation I made a decade or so ago in a UseNet post: there is a large class of static minimally coupled massless scalar field (mcmsf) solutions, in which the spacetime is a curved Lorentzian manifold, but which exhibit "zero gravity", e.g. static observers can hover over a region where the energy of the mcmsf is concentrated without any need to fire their rocket engines!

See "BRS: Static Axisymmetric "Gravitationless" Massless Scalar Field Solutions"

www.physicsforums.com/showthread.php?t=433793

(Mcmsf solutions are exact solutions in which the only contribution to the stress tensor comes from the field energy of a massless scalar field, which is minimally coupled to curvature.)

Exercise: read Post #1 in the above cited BRS. Find the static spherically symmetric solution in this class--- note that it is not given by choosing
w = \frac{1}{\sqrt{z^2+r^2}}
Compute its Komar mass. Think about matching across nested spherical shells to a Minkowski region inside the inner shell, and to a Schwarzschild or Minkowski region (depending upon what you found for the Komar mass) outside the outer shell. (Note that the scalar field "wants" to model a long range interaction, so any such matching will require some explanation: what is the physical reason why the scalar field is nonzero only between the two nested spherical shells?)

 

[Chris Hillman]

Penrose Diagram Confusions, plus: Expanding Space

In "rotating black holes in Penrose diagrams"

www.physicsforums.com/showthread.php?t=433568

the OP is badly confused and I am not up to the task of trying to suggest what to say to him, but its good that several SA/Ms are trying to help. One minor clarification:

The Kerr solution, like the Schwarzschild solution, assumes a perfectly symmetrical collapse.

AFAIK no rotating analog of the Oppenheimer-Snyder collapsing dust ball, which produces a non-rotating hole, is yet known. However, if you look at geodesic congruences of world lines of freely falling test particles, the Doran congruence in the Kerr vacuum is generalizes the Lemaitre congruence from the nonrotating case (Schwarzschild vacuum), and the Doran observers in the equatorial plane do exhibit planar motion as they spiral in.

The Penrose diagram of the interior an actual rotating black hole caused by gravitational collapse is still a matter of some speculation and debate.

AFAIK, the situation remains the same as ten years ago: despite plausible analogies suggesting what to expect, nothing rigorous is known about the interiors of generic rotating holes (e.g. formed by generic collapse of ordinary matter, with some matter or radiation falling into the hole from the exterior region). The very nice paper on mass-inflation cited by JesseM discusses generic charged holes by way of pursuing the analogy.

After having read [the cited arXiv eprint] I do believe that even without [even with?] the mass inflation, there is no white hole.
the structure of the "white hole" if we look at the metric should be exactly the same as the structure of the black hole, and matter could excape because it can be accelerated faster than the speed of light (like matter inside the event horizon) . So this would not be a white hole but a black hole, because the metric would be the same.
Does anyone disagree with this?

I think the appropriate response is: "no-one has any idea what you are trying to say". I bolded some obviously problematic claims (although I can't tell whether he is trying to make a claim or to deny one!).

FWIW, the eternal Schwarzschild and Kerr spacetimes contain both "white hole" and "black hole" event horizons, or more properly, horizons from which particles must emerge from a past interior region into an exterior region, and horizons in which particles which fall from an exterior region into a future interior region cannot re-emerge into the original exterior region. In addition, the eternal Kerr vacuum (and the RN non-null electrovacuum) also contain Cauchy horizons, which must not be confused with event horizons. The eternal Kerr vacuum also contains both and interior asymptotically flat regions (negative Komar mass) as well as exterior asympotically flat regions (positive Komar mass).

BTW, "relativityfan" is making my troll antennae twitch uncomfortably I sense a whiff of Chip on Shoulder, which raises the question of whether this user is using an ironically chosen handle at PF. It's always worth remembering that one reason to "write defensively" when posting in the public areas is that you never know when some putative newbie is planning to cherry pick responses to some "naive question" in order to, say, quote them in some anti-science website. A large number of religiously committed creationists do this quite a bit with regard to anything related to the standard hot Big Bang theory.

In "Geodesics doubts"

www.physicsforums.com/showthread.php?t=424278

the OP is again rather confused (as the poster later admitted). FWIW,

As I'm familiar with the phrase, "space is expanding" is not a coordinate-dependent phenomenon.

Depending on context, it might be reasonable to interpret "space is expanding" to refer, in an exact fluid solution, to the congruence of world lines of the fluid particles, and then the acceleration vector, expansion scalar, shear tensor, and vorticity tensor of this congruence are all geometric, coordinate-free notions. In particular, the expansion scalar gives a coordinate-free notion of whether or not a small ball of fluid particles has expanding or contracting volume. (Oops, just saw that in Post #14, George Jones already said something similar. And yay!, in Post #13, Ben Crowell pointed out that as soon as you start talking about "velocity in the large", things get tricky, and you need to be more precise about what operationally significant notion for defining "distance in the large" you have in mind.)

Expansion of space is purely coordinate dependent.

I think I know why Ich said this, and depending upon how you think of things, that's not wrong either, but wow, this certainly shows why it is so important to introduce some math, or at least to say that without using math, people are likely to wind up talking about different things and thus making apparently mutually contradictory statements.

Another more general set of fundamental observers, independent of FRW symmetries, is defined to be at rest in normal coordinates, maybe one could call them "Einstein observers", as they reproduce the inertial frames of SR if curvature is negligible, on which most people base their intuition.

I see that Ich is thinking of Riemann normal coordinates (on a spatial hyperslice? in a cosmological model?) but I don't understand how he intends to define his observers. If the congruence of their world lines is nonexpanding, however, it will generally be nongeodesic. Also, a rigid congruence is one with vanishing expansion tensor, but not all spacetimes admit such congruences.

From what I've been learning from this thread, we have grounds for selecting a 'canonical' coordinate system - i.e. a point of view where the universe appears homogenous and isotropic.

I think at least two posters are thinking about "preferred timelike congruences" in cosmological models. There are at least two possible interpretations of what this might mean:

• vorticity-free congruence whose spatial hyperslices are homogeneous and isotropic (this puts strong constraints on the spacetime)
• congruence of the world lines of the fluid or dust particles which model galaxies; in a fluid this might not be a geodesic congruence but in a dust solution, it will be a timelike geodesic congruence; however if the vorticity tensor is nonvanishing these world lines will not admit a family of orthogonal hyperslices.

The OP asked Ben

bcrowell, this is what I call gaslight, are you saying that considering expansion as physical fact (as I and many others do) or as a coordinate artifact is just a matter of taste?

The problem is that he still doesn't realize that various notions of "expansion" have been referred to without definition in the thread. If one understand this to refer to the expansion scalar of a timelike congruence having some agreed upon physical significance in a given gtr model, then yes, this is coordinate-free. If one understands "expansion" to mean something else, then it could well be coordinate-dependent. Re what Ben said in his Post #36, if we consider "expansion" to refer to galaxies in a cosmological model, it is reasonable to stipulate that we will use the expansion tensor of the congruence to describe how nearby pairs of galaxies are moving wrt each other. But this only tells us about nearby galaxies, which is probably not what the OP wanted!

Hmm... velocity in the large, visual appearances... as the number of valid but conceptually subtle concepts mentioned in this thread increases, someone who doesn't already understand all of them will probably gain the (false) impression that the signal to noise ratio is increasing. Certainly the OP seems to be becoming increasingly confused, not less so. But maybe that's part of the learning experience.

 

[Chris Hillman]

Tidal tensor of some familiar cosmological models

In "Weyl curvature and tidal forces"

www.physicsforums.com/showthread.php?t=433916

User:TrickyDicky asks about the tidal tensor of the FRW models. He is correct that the Weyl tensor of these models vanishes identically; all their curvature is Ricci curvature, due to the immediate presence of matter (usually taken to be radiation fluid or dust) as per Einstein's field equation. However, the tidal tensor is the electroriemann tensor, not the electroweyl tensor! Thus, a small sphere of initially comoving test particles in an FRW model will contain nonzero mass, so it will contract; by symmetry, the gravitational attraction of nonzero mass outside the sphere cancels out. The tidal tensor shows this isotropic tidal compression.

For example, consider the FRW dust with E^3 hyperslices:
[tex]
ds^2 = -dt^2 + t^{4/3} (dx^2 + dy^2 + dz^2), \; \;
0 < t < \infty, \; -\infty < x, \, y, \, z < \infty
[/tex]
Take the frame of the dust particles
[tex]
\begin{array}{rcl}
\vec{e}_1 & = & \partial_t \\
\vec{e}_2 & = & t^{-2/3} \; \partial_x \\
\vec{e}_3 & = & t^{-2/3} \; \partial_y \\
\vec{e}_4 & = & t^{-2/3} \; \partial_z
\end{array}
[/tex]
Then the tidal tensor is
[tex]
{E\left[\vec{e}_1\right]}_{ab} =
\frac{2}{9 t^2} \; \operatorname{diag} (1,1,1)
[/tex]
indicating isotropic tidal compression.

For comparison, for the Schwarzschild vacuum in the frame of static observers or Lemaitre observers,
[tex]
{E\left[\vec{e}_1\right]}_{ab} =
\frac{m}{r^3} \; \operatorname{diag} (-2,1,1)
[/tex]
(traceless, as must happen for a vacuum solution!), which indicates radial tidal tension and orthogonal tidal compression.

The Jacobi geodesic formula states
[tex]
\ddot{\vec{\xi}}^a = -{E^a}_b \, \xi^b
[/tex]
where [itex]\vec{\xi}[/itex] is a connecting vector (spacelike, short, points from fidudical geodesic to a neighboring geodesic as you let parameter run in both proper time parameterized geodesic curves), and where overdot denotes differentiation wrt proper time,
[tex]
\dot{(\cdot)} = \nabla_{\vec{e}_1} (\cdot)
[/tex]
Or in terms of matrix algebra, if we think of vectors as column matrices,
[tex]
\ddot{\vec{\xi}} = -{\cal E} \, \vec{\xi}
[/tex]
IOW, at each event we have a linear operator which acts on spacelike vectors in the projection of the tangent space to the normal hyperplane element, and this takes each vector to its second derivative wrt proper time, assuming it is connecting our fiducial geodesic to a very nearby one. This only makes sense because the tidal tensor is defined in terms of the Bel decomposition of the Riemann tensor with respect to a particular timelike congruence--- assumed here to be a geodesic congruence.

I say it because I've just read that the Weyl curvature only happens in in empty spacetime, without any gravitational source nearby.

That's not true, assuming "empty spacetime" means a vacuum or maybe electrovacum region. Weyl curvature can happen anywhere, even in a region containing matter. If that weren't true, we could shield against gravitational waves using cardboard boxes.

Two bookmarkable links:

www.math.ucr.edu/home/baez/einstein/
www.math.ucr.edu/home/baez/gr/gr.html

These should help TrickyDicky.

For those of you who use Maxima, here is a Ctensor file you can run in batch mode under wxmaxima, which allows you to easily verify that the Weyl tensor vanishes and that the electroriemann tensor (tidal) tensor has the frame field components I just mentioned:

/* 
FRW dust with E^3 hyperslices; comoving cartesian chart; nsi coframe 

All FRW dusts can be embedded with codimension one.

*/
load(ctensor);
cframe_flag: true;
ratchristof: true;
ctrgsimp: true;
/* define the dimension */
dim: 4;
/* list the coordinates */
ct_coords: [t,x,y,y];
/* define background metric */
lfg: ident(4);
lfg[1,1]: -1;
/* define the coframe */
fri: zeromatrix(4,4);
fri[1,1]: -1;
fri[2,2]:  t^(2/3);
fri[3,3]:  t^(2/3);
fri[4,4]:  t^(2/3);
/* setup the spacetime definition */
cmetric();
/* display matrix whose rows give coframe covectors */
fri;
/* compute a matrix whose rows give frame vectors */
fr;
/* metric tensor g_(ab) */
lg;
/* compute g^(ab) */
ug: invert(lg);
christof(false);
/* Compute fully covariant Riemann components R_(mijk) = riem[i,k,j,m] */
lriemann(true);
/* Compute R^(mijk) */
uriemann(false);
/* Compute Ricci componets R_(jk) */
ricci(true);
/* Compute trace of Ricci tensor */
tracer;
/* Compute R^(jk) */
uricci(false);
/* Compute and display MIXED Einstein tensor G^a_b */
/* For (-1,1,1,1) sig Flip sign of top row to get G^(ab) */
einstein(false);
/* WARNING! leinstein(false) only works for metric basis! */
/* Compute Kretschmann scalar */
rinvariant();
expand(factor(%));
/* Covariant Einstein tensor as matrix */
matrix([-ein[1,1],-ein[1,2],-ein[1,3],-ein[1,4]],
[ein[2,1],ein[2,2],ein[2,3],ein[2,4]],
[ein[3,1],ein[3,2],ein[3,3],ein[3,4]],
[ein[4,1],ein[4,2],ein[4,3],ein[4,4]]);
/* electroriemann tensor */
matrix([lriem[2,2,1,1], lriem[2,3,1,1],lriem[2,4,1,1]],
[lriem[3,2,1,1],lriem[3,3,1,1],lriem[3,4,1,1]],
[lriem[4,2,1,1],lriem[4,3,1,1],lriem[4,4,1,1]]);
/* magnetoriemann tensor */
matrix([lriem[2,4,3,1],lriem[2,2,4,1],lriem[2,3,2,1]],
[lriem[3,4,3,1],lriem[3,2,4,1],lriem[3,3,2,1]],
[lriem[4,4,3,1],lriem[4,2,4,1],lriem[4,3,2,1]]);
/* Weyl tensor vanishes */
weyl(true);
/* Construct NP tetrad for our frame, compute Weyl spinors and Petrov type */
nptetrad(true);
weyl(false);
psi(true);
factor(psi[0]);
factor(psi[1]);
factor(psi[2]);
factor(psi[3]);
factor(psi[4]);
petrov();

For comparison, here is the Schwarzschild vacuum in the frame of the Painleve observers:

/* 
FRW dust with E^3 hyperslices; comoving cartesian chart; nsi coframe 

All FRW dusts can be embedded with codimension one.

*/
load(ctensor);
cframe_flag: true;
ratchristof: true;
ctrgsimp: true;
/* define the dimension */
dim: 4;
/* list the coordinates */
ct_coords: [t,x,y,y];
/* define background metric */
lfg: ident(4);
lfg[1,1]: -1;
/* define the coframe */
fri: zeromatrix(4,4);
fri[1,1]: -1;
fri[2,2]:  t^(2/3);
fri[3,3]:  t^(2/3);
fri[4,4]:  t^(2/3);
/* setup the spacetime definition */
cmetric();
/* display matrix whose rows give coframe covectors */
fri;
/* compute a matrix whose rows give frame vectors */
fr;
/* metric tensor g_(ab) */
lg;
/* compute g^(ab) */
ug: invert(lg);
christof(false);
/* Compute fully covariant Riemann components R_(mijk) = riem[i,k,j,m] */
lriemann(true);
/* Compute R^(mijk) */
uriemann(false);
/* Compute Ricci componets R_(jk) */
ricci(true);
/* Compute trace of Ricci tensor */
tracer;
/* Compute R^(jk) */
uricci(false);
/* Compute and display MIXED Einstein tensor G^a_b */
/* For (-1,1,1,1) sig Flip sign of top row to get G^(ab) */
einstein(false);
/* WARNING! leinstein(false) only works for metric basis! */
/* Compute Kretschmann scalar */
rinvariant();
expand(factor(%));
/* Covariant Einstein tensor as matrix */
matrix([-ein[1,1],-ein[1,2],-ein[1,3],-ein[1,4]],
[ein[2,1],ein[2,2],ein[2,3],ein[2,4]],
[ein[3,1],ein[3,2],ein[3,3],ein[3,4]],
[ein[4,1],ein[4,2],ein[4,3],ein[4,4]]);
/* electroriemann tensor */
matrix([lriem[2,2,1,1], lriem[2,3,1,1],lriem[2,4,1,1]],
[lriem[3,2,1,1],lriem[3,3,1,1],lriem[3,4,1,1]],
[lriem[4,2,1,1],lriem[4,3,1,1],lriem[4,4,1,1]]);
/* magnetoriemann tensor */
matrix([lriem[2,4,3,1],lriem[2,2,4,1],lriem[2,3,2,1]],
[lriem[3,4,3,1],lriem[3,2,4,1],lriem[3,3,2,1]],
[lriem[4,4,3,1],lriem[4,2,4,1],lriem[4,3,2,1]]);
/* Weyl tensor vanishes */
weyl(true);
/* Construct NP tetrad for our frame, compute Weyl spinors and Petrov type */
nptetrad(true);
weyl(false);
psi(true);
factor(psi[0]);
factor(psi[1]);
factor(psi[2]);
factor(psi[3]);
factor(psi[4]);
petrov();

 

[Chris Hillman]

Sectional curvature

In "Weyl curvature and tidal forces"

www.physicsforums.com/showthread.php?t=433916

it looks like the Baez pages cleared up the main confusion, but TrickyDicky introduced a new one!

the spacetimes of constant sectional curvature like Schwartzschild

I don't know how Tricky got that confusion, but its not true.

Take any two-dimensional submanifold S of any Loretzian or Riemannian manifold M. It inherits a metric from the parent and thus has a Gaussian curvature at each point. Choose a point P and consider the tangent plane to S at P. Associated with this is a sectional curvature obtained from the action of the Riemann tensor on bivectors. Computing this wrt a frame field you obtain the Gaussian curvature of S at P.

The metric tensor on M at T_P M induces a metric tensor on the space of bivectors at P. If you use a coordinate basis to compute sectional curvature, you have to divide by a normalization factor which arises from this metric on bivectors. If you use a frame field, you don't need to do that.

Schwarzschild vacuum certainly does not have constant sectional curvature! That is a very rare property for a Riemannian/Lorentzian manifold to have!

My initial guess was that Tricky misread something somewhere concerning a venerable approach to finding initial data with which to start a numerical simulation using an initial value approach to gtr, such as ADM formalism. In this approach, one looks for a conformally flat three manifold to start the evolution; for example, "space at a time" in a time symmetric evolution in which dust is thrown out, hovers momentarily, and falls back---- the "space at a time" where the dust momentarily hovers may be conformally flat. But most spatial hyperslices certainly are not.

BTW, in a Lorentzian manifold, the Bach tensor measures the departure of a given hyperslice from being conformally flat, similarly to the fact that the Weyl tensor measures the departure of the spacetime itself from being conformally flat.

But then Tricky said this:

Schwarschild solution has constant sectional curvature (that of a parabole)

It seems that Tricky misread a WP article (or some idgit munged a WP article). He must be referring to the Flamm paraboloid, an embedding of a t=t_0 hyperslice in the exterior region of Schwarzschild vacuum written in the Schwarzschild chart (only valid on the exterior). But that certainly does not have "constant sectional curvature".

I think this illustrates that people simply cannot hope to understand gtr from reading WP articles or popular books without sufficient mathematical background and ability to understand the math. But maybe I am too pessimistic--- Tricky did seem to learn something valuable from Baez's pages in the end.

the interior Schwarzschild solution (ie. one which contains matter) is conformally flat.

The bolded phrase is ambiguous: newbies typically don't know how to guess correctly whether an author means "Schwarzschild incompressible perfect fluid ball" or "future or past interior region of the Schwarzschild vacuum". Here, atyy means the first.

Penrose is talking about the vacuum Schwarzschild solution, which as far as I understand has non-zero Weyl curvature.

In a vacuum, the Ricci tensor vanishes, so the Weyl tensor agrees with the Riemann tensor in a vacuum region!

The "exact" solution describing our non-uniform universe must somehow contain corrections to all these approximations which must be joined up to each other somehow.

Correct. Just like in any field theory.

is deflection of light outward the sun's rim produced by Weyl curvature or Ricci's?

Weyl. The deflection is an effect of the curvature in the vacuum region outside the Sun (in an idealized model formed by matching a static spherically symmetric perfect fluid (ssspf) ball such as the Schwarzschild perfect fluid across the zero pressure surface to an exterior Schwarzschild vacuum region. Since the curvature there is entirely Weyl curvature, this lensing is a Weyl effect.

In a conformally flat region, by definition there can be no lensing. Thus, no lensing in FRW models, or in the interior of the Schwarzschild incompressible fluid ball (pretending for the sake of argument that light can propagate freely at the speed of light there, which of course isn't true!). Note: most ssspf solutions are not conformally flat, nor are many cosmological models conformally flat.

I was confusing the spatial curvature(paraboloid) of the Schwarzschild metric that is indeed of constan sectional curvature

Not if he is talking about the hyperslice which corresponds to the Flamm paraboloid, and I don't know what other paraboloid he could be talking about.

 

[Chris Hillman]

Re

www.physicsforums.com/showthread.php?t=434034&page=2

an interesting point is that a certain increasingly populous class of satellites, generally launched with little fanfare, are increasingly using various kinds of stealth technology (e.g. nonreflective surfaces), and increasingly engage on a timescale of minutes in various evasive behaviors (e.g.furl sails at certain times, change their geometry in other ways when passing over certain areas, frequently change orbits).

Interestingly, many evasive techniques appear to be aimed specifically at foiling casual observation by amateur astronomers (through telescopes, but certainly these things don't wish to needlessly draw the attention of naked eye observers either). Rather astonishing, considering the expense involved, but apparently true.

Be this as it may, the net result is an increasing frequency with which amateurs notice odd flashes in the night sky, which appear rather unlike flashes from conventional communication satellites in long term, known, stable orbits.

What if anything to say about this in the public areas of PF is, I guess, a matter for discussion in the SA forum.

 

[Chris Hillman]

Precession, Komar integrals, plus Kleinian geometry

Re "Perihelion advance expressed differently"

www.physicsforums.com/showthread.php?t=434694

• As Lut Mentz hinted, one of the nice things about linear field equations is that you can separate out various effects and treat them seperately. In the case of the precession of Mercury, there is a much larger effect due to the influence of the major planets. We can get away with computing the gtr residual or extraNewtonian precession using a simplified model which ignores the outer planets entirely (and the quadrupole moment of the Sun, and...) only because we are using the linearized approximation, which is good enough to obtain the gtr residual to the desired accuracy for Mercury and other cases in our solar system.
• To second order (in an appropriate perturbation expansion) you can think of the motion as Kepler motion on an ellipse in a tranparent piece of plastic which you slowly rotate with constant angular velocity. But higher order terms make the actual motion more complicated, and nonlinear corrections make it even more complicated (in a peturbative approach).
• The perturbation expansion just mentioned refers to the Einstein-Binet equation for timelike geodesics in the exact Schwarzschild vacuum, but Einstein 1915 used a weak field approximate solution instead, which turns out to introduce an unwanted problem. So much better to follow the textbook approach.

Re "Newtonian generalization of Komar mass"

www.physicsforums.com/showthread.php?t=434722

Jonathan Scott (uh-oh!) asks a murky question about Komar mass. I expect Pervect will chime in there, to say (at least) that Komar mass is not defined the way JS suggested. Carroll's textbook has a very nice discussion, but I don't care for his notation! Here's how I'd describe the definition:

Suppose you have an asympototically flat spacetime (Lorentzian 4-manifold) which admits a timelike vorticity free Killing vector [itex]\vec{\xi}[/itex], i.e. we have an asymptotically flat static spacetime. Although this isn't needed, to simply the discussion let's also suppose we have introduced a Schwarzschild type chart so that our line element has the form
[tex]
ds^2 = -f^2 dt^2 + g^2 dr^2 + r^2 \; d\Omega^2
[/tex]
where f,g are metric functions of r only, where we suppose that our Killing vector field [itex]\vec{\xi} = \partial_t[/itex]. Let [itex]\vec{N}[/itex] be the outward pointing unit normal to the spheres r=r0, and let the timelike unit vector field [itex]\vec{U}[/itex] be the normalization of the Killing vector field [itex]\vec{\xi}[/itex]. Then

• Average over the sphere at r = r_0 (Schwarzschild radial coordinate) the quantity
  [tex]
  \vec{N} \cdot \nabla_{\vec{U}} \vec{\xi}
  [/tex]
  (don't forget to use the appropriate Jacobian factor in the integrand!)
• Let r0 tend to infinity

With more thought you can see that this does not actually depend upon adopting a particular coordinate chart, but only upon the assumptions that our spacetime manifold is asymptotically flat and static. Strictly speaking, we don't even need gtr to define any of these notions! It is true that in practice, we need to adopt a chart in which it is mathematically convenient to average over spheres, or at least approximate spheres, provided they become round as r0 -> infty, and provided that our radial coordinate has the required asymptotic properties.

If you apply this to a simple model of an isolated nonrotating star, consisting of a static spherically symmetric perfect fluid ball matched across the zero pressure surface to a portion of a Schwarzschild vacuum exterior region, then you obtain the mass of the spacetime, i.e. the mass of the star. Since the whole point is to take a limit, the Komar mass really only cares about the "shape" of the vacuum exterior, in fact only about the "shape at spatial infinity"! Scott may be thinking of a perfect fluid which has pressure decreasing only asymptotically to zero, and which is asymptotically flat, but if so, not everything which "looks" AF really is, so this needs to be checked. Or he may simply be confused about the definition of Komar mass.

The fun thing about Komar mass is that you can also apply this setup to stationary axisymmetric spacetimes, such as an asymptotically flat sheet (exterior or interior) of the Kerr vacuum, and then you can define and compute Komar angular momentum (about the symmetry axis) in addition to Komar mass. In the case of the Kerr vacuum you obtain the usual mass and angular momentum parameters for the Kerr vacuum solution as usually written (e.g. in the Boyer-Lindquist chart). This requires averaging over approximately round spheres, which turns out to be good enough, as long as they become round in the limit r0 -> infty, and as long as r is an appropriate radial coordinate wrt asymptotic flatness.

These Komar integrals only apply to spacetime models in which we have appropriate Killing vector fields. A more general definition, ADM mass, agrees with Komar mass when the Komar integrals are defined.

In "Is Minkowski space the only Poincare invariant space?"

www.physicsforums.com/showthread.php?t=434555

Arkadiusz Jadczyk (uh-oh!) wrote

If you mean just "a manifold", then you can take any homogeneous space P/H, where P is the Poincare group and H its closed subgroup.

He is correct (that's the basic idea of Kleinian geometry, in fact, one of my fav topics). The OP was probably thinking of four dimensional manifolds. More generally, G/H where G is any Lie group and H any closed subgroup.

Actually, this only gives the smooth manifold portion of a much wider notion of Kleinian geometry. We can in fact take G to be any group, as in "BRS:Exploring the Rubik Group". For example, in order to study things like finite projective spaces over some finite field, we might take G=PGL(d+1,p^n). Then we get finite analogues of various familiar geometries. This turns out to be closely related to the study of finite simple groups--- in This Week, John Baez often discussed various aspects of Kleinian geometry, including these connections.

Minkowski spacetime arises from the case where G is the ten dimensional Poincare group and H is the six dimensional Lorentz group (notice that 10-6=4, as per my posts in "BRS:Exploring the Rubik Group"), and you can obtain discrete quotient spaces of Minkowski spacetime using Klein's approach. It matters very much whether or not we include "improper motions" in G or H!

It is easier to explain the simpler case of the round sphere S^2 and its discrete quotient round RP^3. Let's take G = SO(3), which will give Riemannian isometries on "round manifolds". In particular, S^2, as a homogeneous Riemannian two-manifold, arises as SO(3)/SO(2), while RP^2, as a homogeneous Riemannian two-manifold, arises as SO(3)/O(2) (quotient by a larger one dimensional closed subgroup, a discrete supergroup of SO(2), which gives a discrete two-fold quotient of S^2, by identifying antipodal points). In both cases, each element of SO(3) acts on S^2 or RP^2 as a proper isometry, in the sense of Riemannian geometry.

Other ways of representing "the sphere" give or remove various structural features, as appropriate depending on context, e.g. we might be interested in removing some of the Riemannian manifold structure. In particular we can remove some of the metric space structure, while retaining just enough structure to define conformal geometry on the sphere. To do this we should take G to be the Moebius group, and then we can find a closed subgroup H such that G/H is the sphere, but this is the sphere endowed with conformal geometry rather than Riemannian metric geometry. Now each element of the Moebious group (recall their classification into elliptic, parabolic, hyperbolic, loxodromic elements!) acts on S^2 as a conformal motion.

Twistor theory starts by exploiting the Lie group isomorphism between the Lorentz group and the Moebius group.

So it really matters here whether one thinks of something like the rotation group as SO(3) or O(3), and similarly for how you think of the "euclidean group" (the semi-direct product of the group of translations with the rotation group, which makes the group of translations a normal abelian subgroup of the euclidean group). Similarly for the Poincare group and the Lorentz group.

A great deal is known about the possible isometry groups for various kinds of exact solutions in gtr; see Stephani et al., Exact Solutions of the Einstein Field Equations, Cambridge University Press.

 

[Chris Hillman]

Radiation Pressure

In "Radiation pressure"

www.physicsforums.com/showthread.php?t=434798

TrickyDicky wrote:

my main confusion comes from the fact that if the Stress-energy tensor for electromagnetic radiation is traceless, that would imply the pressure components of the tensor equal zero, and yet it's obvious radiation exerts pressure when absorbed or reflected and radiation pressure plays an important role in star dynamics.

(the bolded phrase is of course incorrect). Replies included:

What nicksauce is saying is that the stress-energy tensor of radiation looks like:
[tex]
\begin{bmatrix}
\rho & 0 &0 & 0 \\
0 & -p & 0 & 0 \\
0 & 0 &-p & 0 \\
0 & 0 & 0 & -p
\end{bmatrix}
[/tex]
With p = rho/3, the trace is zero.

Components wrt a frame field, of course!

I think there might be some confusion here. For radiation pressure to accelerate a bit of matter, the radiation cannot be impinging in completely isotropic fashion. But if an electron is moving wrt the CMBR (for example), it does experience a drag force from radiation pressure which scales like the fourth power of the temperature; see Peebles, 5.6.

Also, nicksauce/phyzguy gave the contribution to the stress tensor of a radiation fluid (as in an early epoch in cosmology) rather than the contribution of an EM wave, which might be closer to what Tricky wanted. For a plane wave (components wrt a suitably "aligned" frame field!)
[tex]
\begin{bmatrix}
\varepsilon & \varepsilon &0 & 0 \\
\varepsilon & \varepsilon & 0 & 0 \\
0 & 0 & 0 & 0 \\
0 & 0 & 0 & 0
\end{bmatrix}
[/tex]
Which is also traceless, and shows a directional pressure term. The off-diagonal terms shows the momentum. A spherical wave looks like a plane wave in a very small region far from the source of the radiation.

Bit rushed, so can't say more right now...

 

[Chris Hillman]

BRS: proper distance, underdensities, warp drives, &c., ad nauseum

In ""Proper distance" in GR"

www.physicsforums.com/showthread.php?t=437895

I am aware of two meanings of the term "proper distance" in GR. The first is when you have points in flat space-time, or space-time that's locally "flat enough", in which case it is defined as it is in SR, as the Lorentz interval between the two points. This usage of the term implies that one is considering short distances, or is working in a flat space-time.

Right, and in this case one can describe an unambiguous "proper distance in the large", because of a remarkable property of Minkowski spacetime: through each pair of events there is precisely one geodesic. There are a handful of other homogeneous (transitive isometry group) spacetimes with the same property --- they are often are often used as cosmological models, but that's not really relevant here--- such as de Sitter lambda vacuum.

A good exercise is to identify these spacetimes and to work out the "proper distance in the large" formula, analogous to the Pythagoras-Minkowski formula
[tex]
d( (t,x,y,z), (t',x',y',z')) = -(t-t1)^2 + (x-x1)^2 + (y-y1)^2 + (z-z1)^2
[/tex]

In a more general spacetime, there will be multiple geodesics between two "non-nearby" events, so there is no hope of a "proper-distance in the large" formula. However, if one clearly has in mind a specific geodesic curve, one can integrate ds along the curve and call that proper distance. Actually, one normally says "proper time" if the curve is everywhere timelike and "proper distance" if the curve is everywhere spacelike. Curves which are timelike here and spacelike there are not often considered! And for null curves, of course, "proper distance" makes no sense.

In the case of something like an FRW model, this is the idea behind integrating along a spacelike geodesic which lies entirely in some "constant time slice". (Note that in a generic hyperslice will bend away from a generic spacelike geodesic which is tangent to the slice at some event on the slice.)

I don't think there's anything to be gained by arguing over whether some definition of "spatial distance in the large" is the "right" definition. There are multiple distinct operationally significant definitions possible, and that's all there is to it. OTH, if you want to discuss in coordinate-free, geometrically meaningful terms the relative motion of a family of observers whose world lines are given by some timelike congruence, then the decomposition of the associated timelike unit vector field into acceleration vector, expansion tensor, and vorticity vector, is just what you want.

Suppose a congruence of timelike worldlines of "fundamental" observers is picked out by phyics, symmetry, etc. Consider spacelike curve that intersects each worldlne in the timelike congruence orthogonally, and that has unit length tangent vector. Proper distance for the congruence is given by the curve parameter along such a spacelike curve. Sometimes these spacelike curve are geodesics, and sometimes they are not.

But there are many ways of continuing a curve given just one tangent vector, so there is too much multiplicity here to be really useful, I think. OTH, the decomposition is unique, but of course dependent upon choice of congruence! Also, don't forget that only an irrotational congruence admits a family of hyperslices everywhere orthogonal to the world lines--- for a congruence with vorticity, no "constant time slices" exist--- oh, I see now, George already said this:

A congruence is hypersurface orthogonal if and only if the vorticity of the congruence vanishes.

I believe there is a general consensus, then, that the term "proper length" in GR needs additional specification besides two points: the curve along which the length may be specified, or the hypersurface of "constant time" in which the curve lies might be specified as an alternative, or indirect means might be used to specify the hypersurface (for instance it being orthogonal to a particular preferred family of observers).

Wish I'd being paying attention, because I demur: in a generic situation, there will be no "hypersurface of proper time" because the congruence has vorticity. However, the decomposition always makes sense and is always informative. But in the literature, I'd say that the general usage is that "proper distance" (or "proper time" for timelike curves) is the integral of ds along a everywhere spacelike (everywhere timelike) geodesic, bearing in mind that there may be more than one such geodesic between two events, and that most spatial hyperslices will bend away from a spacelike geodesic tangent to some event on the hyperslice, and that such a slice need not have any nice relation to any timelike congruence which may be physically interesting.

the curve is specified implicitly as (informally) "the shortest curve connecting the two points" or more formally the distance is specified as the greatest lower bound of all curves connecting the two points.

I think pervect was thinking of spacelike geodesics between two nearby events, but in general there will be multiple geodesics between two events (e.g. on an ordinary two-sphere) giving different lengths between the two points. Also, in the sequel of the thread, some posters appear to be confused about the variational principle behind the notion of geodesics: it says that when we have a geodesic curve between two events, and make a small variation (small to first order), the integral of ds is consant to second order. It doesn't say whether this integral increases or decreases. In flat spacetime, it is true that for a timelike geodesic, it will decrease, and for a spacelike geodesic, it will increase. But the point is that for a non-geodesic curve, a first order variation will result in a first order change in the integral of ds. So the variational principle says that the integral of ds is stationary, not that it is an extremum.

I thought for a specific spacetime with a specific curvature, there could only be one geodesic that precisely defines the shortest path. And that would also be the most "natural" definition of proper distance.

In Riemannian geometry, there is quite a bit of theory on inferring properties of geodesics from properties of the curvature tensor, and vice versa. Most of this depends on positive definiteness. There is also considerable theory relating properties of geodesics to properties of curvature tensor in Lorentzian geometry, but it has a different flavor since no positive definiteness. See respectively Berger, A Panorama of Riemannian Geometry and Stephani et al., Exact Solutions of Einstein's Field Equations.

In "Opposite side" of GR"

www.physicsforums.com/showthread.php?t=437613

the OP is struggling to discuss something like this: a dust solution which has a region of underdensity (in a spatial hyperslice, not neccessarily related to the world lines of the dust particles, this region should be compact), possibly spherically symmetric although that is not generic, and outside agrees locally with some FRW dust solution. This exactly the situation discussed at length elsewhere in the BRS!

In

https://www.physicsforums.com/showthread.php?t=438007

the OP is caught up in the issue of multiple distinct operatationally distinct notions of "distance in the large", hence "velocity in the large". This is why warp drive metrics do not contradict the principle that at each event the tangent space is Lorentzian. But a large body of work since Alcubierre's papers shows that warp drives are almost certainly not physically realizable, and moreoever, if they were, so would be "time machines" and other outlandish devices. There is no completely solid disproof, and you can never tell what the future might bring, but right now it seems that there is no point on say spending large sums on looking for ways to make warp drives, because right now theory suggests strongly that it simply cannot be done.

 

[Chris Hillman]

Surface of Last Scattering; 2+1 Gravity; Bode's Numerology; Isolated Objects

In "Dark Matter or Dark Mass?"

www.physicsforums.com/showthread.php?p=2952416#post2952416

the OP asks about the surface of last scattering. He wants to know why the CMBR appears to come from every direction and from a certain epoch (in FRW cosmology, a certain constant "cosmological time" hyperslice). I wonder whether putting up a figure might help? See below for a suggestion.

If the figure isn't clear, make a model!

• buy a box of paper drinking straws (paper straws will buckle more readily than plastic ones),
• say they are 20 cm long; bend all the straws at 15 cm,
• get a piece of cardboard, and draw two equal intersecting circles each of radius [itex]15/\sqrt{2}[/itex] cm on the cardboard,
• pierce a piece of cardboard at some points each circle,
• push the straws through the cardboard, and gather the ones piercing each circle to make two cone shaped configuration (glue them at the apex with Elmer's glue or tie them at the apex with laundry ties, or something like that, but make sure that all the straws should everywhere make slope 1 wrt the cardboard),
• from the apex of each "cone", you can hang a differently colored straw, cut so that it doesn't reach the surface of last scattering, or hang a short strand of colored yarn; these represent the world lines of the two galaxies (these world lines should be orthogonal to the surface of last scattering, or nearly so, and since the galaxies formed long after the epoch of last scattering, they shouldn't reach to the cardboard),
• randomly bend each straw at a few points below the cardboard in random directions but still keeping slope 1 wrt the cardboard (that is, below the cardboard, each straw should each be bent several times in random directions, but making slope 1 wrt the cardboard, while above the cardboard, all the straws should be straight and make slope 1 wrt the cardboard).

This is very crude schematic model of an FRW model, represented in a conformal chart; the cardboard models the surface of last scattering, and the straw bending below this surface suggests the scattering. The model shows how two observers each measure radiation coming from all directions from events at the same epoch (the hypersurface corresponding to the surface of last scattering).

In "BTZ black hole"

www.physicsforums.com/showthread.php?t=441565

the OP asks about an exact solution modeling a Kerr analog in 2+1 gtr. But the analogy is not very close since in 2+1 dimensions, the Weyl tensor vanishes identically, so gravitation is not a long range force. That means that in model "2-stars", the matter filled interior (think of a 2-hemispherical cap) is curved but the exterior is locally flat (think of a conical frustrum).

Oh, noooo! In "Bode's Law"

www.physicsforums.com/showthread.php?t=441275

the OP inquires whether the notorious so-called "Bode's Law" (an infamous item of approximate numerology) is somehow validated by data on extrasolar planets. The answer is that Bode proposed a small integer relationship between the mean radii of the major planets, which is certainly not true (except very very approximately) in our solar system and even less so in others. So that would be "no, but hardly worth dignifying with an answer".

But there is a kernel of truth in the implicit observation that there is much which no one yet knows about the formation of solar systems. In particular, the above theory starts with a more or less fully formed solar system in which some near integer approximate relations happen to exist at least momentarily, and (with good sucess) tries to say whether these coincidental relations will be quickly destroyed by various perturbations, or will be preserved and even refined.

Someone mentioned tidal locking of the rotational periods of certain moons of Jupiter. The point that wasn't brought out clearly is that the theory of dynamical systems shows why looking at periods is preferable to looking at lengths if you want to look for small integer approximate relations in specific solar systems. As DH didn't quite say, many aspects of dynamical systems relevant to solar system dynamics are now well understood; in particular, there is good understanding of why some near integer relations are unstable (so that the orbits evolve to disrupt these near-relations) while others are stable (so that the system preserves them and may even make them better approximations over time). A good key phrase is KAM theory; I can provide citations to expositions aimed at mathematicians who are not specialists.

Coming back to looking for small integer ratios, the Greeks invented the very nice theory of simple continued fractions precisely to efficiently find good approximations by small integer ratios when they exist. This applies to anything, so it is in effect a machinery for doing numerology. Of course, in "applications" this is, in general, a mathematical analog of a parlor trick with cards: an artfully constructed illusion. Entertainment, not science.

In "Rod shortening of General Relativity"

www.physicsforums.com/showthread.php?t=441522

the OP asked for a "formula for rod shortening", apparently thinking of some alleged spatial analog of gravitational red shift for the gravitational field (possibly) nonrotating isolated objects. Ben Crowell pointed out that the OP failed to specify what spacetime he had in mind--- my guess is that the OP may have been trying to ask for a general "rod-shortening" formula for asymptotically flat Weyl vacuum solutions in the weak-field approximation (since the OP mentioned "potential", which makes sense, sort of, for weak-field approximations to Weyl vacuums)

User:yuiop assumed the OP was asking only about the Schwarzschild vacuum, and replied:

the simplest equation is:
[tex]
dL = \frac{dr}{\sqrt{1-2m/r}}
[/tex]
in units of G = c =1, where dL is the length of a short rod according to a local observer and dr is the length of the rod according to the Schwarzschild observer at infinity.

Because yuiop has not specified a measurement procedure to be used by a (static?) observer near spatial infinity, this doesn't make sense as stated. His claim can be fixed up, but the required procedure seems rather artificial to me:

The metric tensor, represented in Schwarzschild exterior chart, is
[tex]
ds^2 = -(1-2m/r) \, dt^2 + \frac{dr^2}{1-2m/r} + r^2 \, d\Omega^2,
\; \; r > 2m
[/tex]
If t increases but radius and angles remain constant, r=r0, r0>2m, this specializes to
[tex]
ds = \sqrt{1-2m/r_0} \, dt
[/tex]
which gives the redshift for a signal sent by a static observer at r=r0, r0> 2m, to an observer at r=infty. If we hold t and angles constant but increase radius from r=r0 to r=r0+dr, we obtain instead
[tex]
ds = \frac{dr}{\sqrt{1-2m/r}}
[/tex]
On the face of things, this expression simply gives the radial scale factor near r=r0 for the Schwarzschild radial coordinate, i.e. it describes a characteristic of the coordinate chart, not the geometry.

It is true that (unlike most alternative radial coordinates), the Schwarzschild radial coordinate has some geometric significance. In particular

• the area of the nested two-spheres implied by the spherical symmetry of the spacetime is proportional to r^2 as r varies, i.e. r is the "areal radius" of these spheres,
• 1/r0^2 is the constant Gaussian curvature of the sphere at r=r0,
• 1/r is the optical expansion of the principle outgoing null congruence (which has spherically expanding wavefronts),

However, one must do more work to explain a physical measurement procedure which explains what yuiop means by "measures at infinity".

The metric tensor for Weyl vacuums, in the Weyl canonical chart, is
[tex]
ds^2 = -\exp(2u) \, dt^2 + \exp(2v-2u) \, (dz^2+\rho^2) + \exp(-2u) \, \rho^2 \, d\phi^2
[/tex]
where u,v depend only on z,rho, where u is axisymmetric harmonic, [itex]u_{zz}+u_{\rho \rho}+u_{\rho}/\rho=0[/itex], and where v is determined from u by quadrature. To first order in u, v must be a constant. For an isolated object, u,v must tend to zero as [itex]r = \sqrt{z^2+\rho^2}[/itex] grows, so v must be zero and then
[tex]
ds^2 \approx -(1+2u) \, dt^2 + (1-2u) \, (dz^2 + d\rho^2 + \rho^2 \, d\phi^2) = -(1+2u) \, dt^2 + (1-2u) \, (dr^2 + r^2 \, d\Omega^2 )
[/tex]
Because u is an asymptotically vanishing axisymmetric harmonic function which does not depend on t, it may be identified with the Newtonian gravitational potential of an appropriate isolated object. Thus, if t increases but the other coordinates remain constant, the line element specializes to
[tex]
ds \approx (1+u) \, dt
[/tex]
which gives the redshift for a signal sent by a static observer at r=r0 to an observer at r=infty. Redshift, since [itex]dt > ds[/itex].

If we hold t, \phi constant but increase [itex]r = \sqrt{z^2+\rho^2}[/itex] from r=r_0 to r=r_0+dr, the line element specializes to
[tex]
ds \approx (1-u) \, dr
[/tex]
which is probably what the OP was trying to ask for. But does it make sense to call [itex]dr < ds[/itex] "rod-shortening"? I can think of an interpretation, but it's not very straightforward.

For the weak-field approximation to the Schwarzschild vacuum, we have of course [itex]u = -m/\sqrt{z^2+\rho^2} = -m/r[/itex], the Newtonian potential for a spherically symmetric isolated massive object.

Figures:

• "Surface" of last scattering (schematic)
• Red-shift vs "rod-shortening" (?) in AF Weyl vacuums

 

[Chris Hillman]

BRS: Does EM radiation gravitate? (Yes)

In "Do photons create gravity?"

www.physicsforums.com/showthread.php?t=442266

Tantolos asks (duh!) "do photons create gravity?" I've seen so many threads with this title that it seems fair to call it a FAQ.

Here's my stab at a suggested stock answer:

Photons are a concept belonging to a QFT, whereas gtr is a classical field theory. So it makes little sense to ask about photons in gtr, because gtr don't know nuthin about quantum concepts. But it does make sense to ask: "according to gtr, does EM radiation contribute to the gravitational field?" The answer is "yes". In fact, according to gtr, all forms of mass-energy contribute to the gravitational field. (See "BRS: Massless Scalar Field Gravitationless Solutions" for an example showing that this does not imply that all forms of energy neccessarily "gravitate" in an intuitive sense, however.)

A particularly simple example are the plane waves associated with a null Killing vector field (the wave vector field) [itex]\vec{k}[/itex], in which wrt a suitable frame field the energy-momentum-stress tensor takes the form
[tex]
T^{ab} = \varepsilon \, k^a \, k^b
[/tex]
Both the Ricci and Weyl tensors are nonzero in such examples; the Riemann curvature tensor is built from these pieces, and in gtr it models the gravitational field. Since it is nonzero in an EM plane wave, plane waves are associated with a nonzero gravitational field. However, the gravitational field of EM waves we can create in the lab are much too small to measure.

In principle, when two laser beams pass nearby each other, the combined gravitational field of the EM field energy and momentum contained in the two waves should lense each laser beam. Again, this effect is much too small to measure.

 

[Chris Hillman]

BRS: Random Comments. Charts, singularities, lazy posters -> confusion

In "Eddington Finkelstein coordinates in the Schwarzschild spacetime",

www.physicsforums.com/showthread.php?t=444995

vitaniarain asks

do the Eddington-Finkelstein coordinates allow to cover the maximal analytic extension of the Schwarzschild spacetime? if not what region do they cover?

No. The ingoing chart covers a certain region (right exterior and future interior) of the full spacetime, and the outgoing chart covers another partially overlapping region (right exterior and past interior); see the BRS on Penrose-Carter conformal diagrams for details.

In

www.physicsforums.com/showthread.php?t=445513

jinbaw asks about some coordinate transformations of the Schwarzschild vacuum metric written in the usual chart on the exterior (except that he sets m=1/2). He transforms to two new charts:

• ingoing Eddington,
• "pre-Kruskal-Szekeres"

He observes that the second chart still has a coordinate singularity at r=2m. This is correct, and introducing the K-S factor removes it. See any gtr textbook which discusses the Kruskal-Szekeres chart.

The OP and DaleSpam use "singularity" to refer to the "coordinate singularity" at r=2m. In his response, bcrowell confusingly uses "singularity" to refer to "curvature singularity". This is sure to cause confusion, and the issue comes up constantly. The only solution, IMO, is to write out "coordinate singularity" and "curvature singularity". Even better, one can refer to "strong scalar spacelike singularity", meaning a curvature scalar (e.g. Kretschamann scalar) blows up and the singularity is strong in the sense that essentially any observer approaching it will experience destructive spaghettification in finite proper time. Some "weak null singularities" which occur in certain exact gravitational plane-wave solutions do not have this property; some observers will experience curvatures which diverge too rapidly, as it were, to tear/crush their bodies (think expansion tensor).

BTW, to amplify what Ben said, Penrose pointed out that pp-waves have the property that all their curvature invariants (even ones formed using scalar invariants built using covariant derivatives of the curvature tensor) vanish identically. However, their curvature tensors almost never vanish. This is roughly analogous to the fact that in Lorentzian geometry, a nonzero vector may have zero "squared length" (namely, the null vectors have this property).

In "Shear stress in Energy-momentum Stress Tensor"

www.physicsforums.com/showthread.php?t=444006

Q-reeus asks about the significance of off-diagonal terms in the stress tensor.

In fact, his question really does not refer to spacetime or gtr at all, but to the 3x3 stress tensor in continuum field theory in ordinary euclidean space. In fact, to any symmetric tensor field.

As usual, it helps greatly to use an orthogonal frame field (placa a frame---three mutually orthogonal unit vectors--- at each point, with this frame field smoothly varying from point to point). The principal axis theorem states that any symmetric matrix can be brought to diagonal form by an orthogonal transformation, i.e. by simply rotating the frame. So, in the stress tensor, can any off-diagonal terms always be transformed away?

The answer is that by rotating the original frame field appropriately at each point, one can obtain a new frame field which is aligned with the principle axes, so that no off-diagonal terms appear when the symmetric tensor is expressed in this adapted frame field. However, in elasticity problems, the adapted frame will in general not align with the surface of an object, so we cannot eliminate surface shear stresses, in general, by changing to a frame field adapted to the principle axes at each point of our stress tensor field.

See my old PF thread, "What is the Theory of Elasticity?", for more about the stress tensor, shear stresses, etc.


Pervect's Post#2 mentions the Komar integrals, but IMO this is not what is confusing the OP.

So one can argue that "gravitational energy" does NOT act as a source of gravity. On the other hand, the Einstein field equations are non-linear; this implies that gravitational waves interact with each other (unlike light waves in Maxwell's (linear) theory). So one can argue that "gravitational energy" IS a source of gravity."

Well it can't be both at the same time surely.

It is merely the imprecision of natural language which leads to the incorrect perception of a "paradox" here. Roughly speaking, in gtr, the gravitational field (Riemann curvature) certainly carries energy and momentum, and this certainly gravitates (affects the Riemann curvature), but the effects are not "ultralocal" in the terminology of Visser, so the contribution of the gravitational field itself is not represented in the stress-momentum-energy tensor.

why should there be any such contested topics after 95 years of GR?

There are hotly contested topics in non-relativistic field theory!

"Why haven't mathematicians found the general solution of the Navier-Stokes equations, after more than a century?" Also, "why can't physicists decide whether Mach principles make any sense?" Et cetera.

Some questions are just plain hard to definitely resolve, that's why.

I haven't read all of "Einstein Field Equations?"

www.physicsforums.com/showthread.php?t=431843

but I get the impression that the OP is making the mistake of worrying about philosophical problems before understanding the geometric intuition. Also, this thread illustrates the other two among the three most common sources of perennial but avoidable confusion due entirely to posters adopting lazy bad writing habits!

To repeat:

• "flat" can mean "(locally) Ricci flat", "(locally) Riemann flat", "(locally) conformally flat", "(locally) flat spatial hyperslices", etc.,
• "Schwarzschild interior solution" can mean "interior region of the Schwarzschild vacuum solution" or "Schwarzschild "incompressible" perfect fluid solution",
• "singularity" can mean "curvature singularity" or "coordinate singularity" (or some other things like "shell-crossing singularity")

and if you don't write out which you mean, you'll confuse others and probably yourself.

Peter Donis says

For instance in the Schwarzschild solution, which is one of the simplest solutions, we could 'push' all the curvature into the 'time' dimension.

Presumably referring to the fact that in the ingoing Painleve chart, the constant Painleve time charts are locally isometric to E^3. However, "curved time dimension" makes no sense and I recommend against trying to think of things like this!

I don't know for sure if, mathematically, we can always find an embedding in a higher-dimensional *Euclidean* manifold.

If you allow indefinite signatures, any Lorentzian manifold can be locally embedded in a fairly small flat space and globally in a really huge one. If you search for embeddings in Ricci flat spaces (with appropriate signature), you need even fewer extra dimensions than for euclidean dimensions. There are known results concerning how many extra dimensions you need for various families of exact solutions.

can we only in case of static curved spacetimes push all the curvature into the time dimension? I think the answer is yes, could someone confirm this is the case for the Schwarzschild interior solution?

That doesn't really make sense, but FWIW,

• there are plenty of dynamical solutions, e.g. FRW models with E^3 slices, which allow spatially flat hyperslices; there are also static solutions which allow such hyperslices, such as as Schwarzschild vacuum (in the exterior; inside, we still admit such slices but the spacetime is dynamical there),
• the Schwarzschild fluid admits spatial hyperslices orthogonal to the world lines of the fluid elements, which have constant positive curvature (locally isometric to S^3).

Figure:

• It is impossible to eliminate shear stresses by using a frame adapted to principle axes at each point

 

[Chris Hillman]

Radiation; plus, Let me out! (of the Universe)

In
"A question on Gravity Waves and Gravity Radiation"

https://www.physicsforums.com/showthread.php?t=446961

Tanelorn asked

Does Relativity estimate or predict the frequency of the gravitational radiation?
Can we also estimate the amount of gravitational radiation being emitted and thus the amount present at a gravity wave detector here on earth?
Are there any other causes which might explain the loss of orbital energy?

and got an excellent reply from Janus.

To elaborate slightly: a useful rule of thumb is that a inertial observer distant from an isolated system measures gravitational radiation whose properties are determined by the motion of the system projected onto a 2-plane orthogonal to the line of sight. Thus, in the case of an isolated binary system, a distant observer aligned with the axis measures circularly polarized radiation at frequency [itex]\omega[/itex], because the projected motion looks like a rotating barbell, while a distant observer in the plane of the orbit measures linearly polarized radiation at frequency [itex]2 \, \omega[/itex] because the projected motion looks like a barbell extending and compressing, as it were. (See the figure below.) At intermediate angles, a mixture of the two frequencies will be observed.

Here, only the two endpoints of the barbell are massive, and the amplitude of the radiation is determined from the second time derivative of the traceless quadrupole moment of the system. Even though the barbell is not changing shape (ignoring the very slow decay of the orbit!), because it is rotating the distribution of mass-energy and momentum is changing wrt an nonrotating inertial frame, and in particular, the quadrupole moment of the stress-monentum-energy tensor is changing wrt time. The details can be found in Schutz, A First Course in General Relativity.

Then Tanelorn asked a followup question

So gravity waves are nothing more than variation with time of the static gravitational field?

This sounds like a variation of the issue worrying Ben Crowell. I urge responders to choose their words carefully to avoid "exciting the Van Flandern kookmode" I'd say something like this:

In the linearized approximation to the EFE, gravitational radiation emitted by an isolated gravitating system is identified as time variations in the Riemann tensor field (fourth rank) which

• propagates as a wave,
• is transverse to the direction of propagation,
• propagates through vacuum at the speed of light,
• when the gravitational field (Riemann tensor) is decomposed into a rapidly varying radiative and a slowly varying portion (the Coulomb tidal field), the radiative component decays like 1/r whereas the Coulomb component decays like 1/r^3,
• when the gravitational field (Riemann tensor) is decomposed into three pieces (second rank 3-dimensional) wrt an observers world line (Bel decomposition), the electroriemann and magnetoriemann pieces of the radiative part of the gravitational field have comparable magnitude (when expressed relativistic geometric units); this is usually not true for the Coulomb component ( in a vacuum region, we can forget about the third piece, the toporiemann piece),
• at large r, the radiation dominates, and then the principal Lorentz invariants of the field both vanish
  [tex]
  R_{abcd} \, R^{abcd} = R_{abcd} {{}^\ast\!R}^{abcd} = 0
  [/tex]
• heuristically, the radiative component would correspond in a QFT to spin-two massless exchange particle, the "graviton", but this turns out to be naive and no complete quantum theory of gravitation is yet known.

For comparison, in Maxwell's theory of EM, EM radiation emitted by an isolated charged system is identified as variations in the EM field tensor field (second rank) which

• propagates as a wave,
• is transverse to the direction of propagation,
• propagates through vacuum at the speed of light,
• when the EM field tensor is decomposed into a rapidly varying radiative and a slowly varying portion, the radiative component decays like 1/r whereas the Coulomb component decays like 1/r^2,
• when the EM tensor is decomposed into two vector fields (first rank 3-dimensional) wrt an observer's world line into electric and magnetic vectors, the radiative portions have comparable magnitude and properties; this is usually not so for the Coulomb component,
• at large r, the radiation dominates, and then the principal Lorentz invariants of the field both vanish
  [tex]
  F_{ab} \, F^{ab} = F_{ab} {{}^\ast\!F}^{ab} = 0
  [/tex]
• heuristically, the radiative component would correspond in a QFT to spin-one massless exchange particle, the "photon", and this is fully realized in QED.

MTW offer a very clear discussion of the effect of linearly and circularly polarized gravitational radiation on a cloud of intially comoving test particles.

In classical gravitation theories other than gtr, gravitational radiation is usually predicted, but may have properties and effects on test particles which differ significantly from the gtr predictions, e.g. might include longitudinal components.

The description of radiation in gtr is somewhat oversimplified: in the full, nonlinear EFE, radiation is a bit more complicated than just described, but this isn't expected to be relevant to understanding gravitational wave detectors near Earth, or even to change current expectations about sources of radiation.

I understand that gravity wave detectors have been built deep underground to prove gravity waves exist. Would we expect to be able to detect this level of gravitational radiation here on Earth with the sensitivity of our detectors and with the level of noise and interference here and elsewhere?

Not deep underground. The on-line resources in the BRS thread "Resources for SA/Ms" will answer the second question.

In "Google Street View Camera Vehicles Collected WI FI data"

https://www.physicsforums.com/showthread.php?t=442640

edward writes

Google claims it was totally inadvertent that they collected wi fi data using their street view camera vehicles. Personally I can't bring myself to believe that...This was first revealed May but for some reason is just now hitting the fan again. It is totally unbelievable that they could have mistakenly done this world wide...But I still wonder why they did it. This was a world wide venture, and that means a lot of unintentional data was collected. It must have cost them a lot of money to collect information that they claim that they will now delete.

What, you thought I was actually going to comment?! I advise one and all to avoid using "friendship" features at social networking sites, but any curious SA/M knows what to do if they want the answer to Edward's question: shoot me an encrypted PM. (See the BRS thread "PKI Cryptosystems for SA/Ms: A Tutorial", which should have been titled "Personal Cryptography: a Tutorial for SA/Ms", but too late to change it now.)

In "The future of Cosmology"

https://www.physicsforums.com/showthread.php?t=446222

Tanelorn got some fine resposes from marcus and twofish-quant, but remarked

it would be very good indeed to understand our universe as well as possible before we ourselves are forced to leave it.

I wonder: what precisely is his plan for leaving the universe? As regular readers know, I am unhappy with The State of Things, so I think I'd like to hitch a ride if he's offering

Figure:

• Gravitational radiation from a binary system (schematic)

 

[Chris Hillman]

BRS: energy-momentum complexes, rotating star models, symmetries

Re "How does empty space curve?"

www.physicsforums.com/showthread.php?t=305652

unless I am missing some subtelty here spacetime still doesn't curve except in the presence of some stress-energy.

That's the right spirit, I think, but remember that in gtr, gravitational energy/momentum is not represented in the stress-energy tensor. So in a vacuum region (no nongravitational energy/momentum) we have zero Ricci curvature but nonzero Weyl curvature (associated with the gravitational field itself)

Einstein's equations can be written in the equivalent (Maxwell-Like) form

Any equation involving (one of many distinct) "gravitational-energy complexes" (which are pseudotensors) is not a true tensorial equation, which means for example that in some charts the "gravitational-energy" will vanish in a given small region while in others it will not. That kind of thing can be problematic, and despite a resurgence of interest in the past five years or so, IMO it is still fair to say that energy-momentum pseudotensors don't really provide much help in resolving the tricky issue of how to represent gravitational energy/momentum in gtr.

Is there any reason to believe that the resulting boundary conditions would represent any "massless" physical situation? In other words, is there any reason to believe that you could physically have a Schwarzschild spacetime without the presence of a mass?

I think robphy was describing the idea that in a stellar model consisting of the world-tube of a ball filled with perfect fluid (say) matched across the surface of the "star" to a vacuum solution (if the ball is static this will be a portion of the Schwarzschild vacuum exterior region), we can isolate any vacuum neighborhood and consider that a "local vacuum solution". (Local in the sense of local neighborhood, as is standard in mathematics and hibrow physics.) In such a local vacuum solution, the presense of a source somewhere outside the domain covered is often implicit in some more or less murky fashion (but inferring the presence and location of a static spherically symmetric source is about as easy as such inferences get in gtr, I think). I think robphy was also referring to an elaboration in which one tries to match various local solutions to create solutions with strange global properties. There are quite a few examples in the arXiv (not easy to find, perhaps).

Regarding the quote by Geroch, I'd need to see more context to say more, but it is good to know that there are general theorems concerning asymptotically flat vacuum solutions and notions such as ADM and Bondi energy/momenta which give well-defined, general, and useful notions of the mass-energy and momentum of "isolated systems". But these don't apply if we toss in a bit of Lambda, so there is much work yet to be done.

Many of the newbie comments, e.g. by "Feullieton", are useless unless the posters clarify what they mean by "exist" (in Nature? in theoretical models? models in what theory?) and so forth. Basically, I think some of the newbies want to discuss the philosophy of physics (and the philosophy of manifold theory and differential geometry) but haven't yet recognized this.

In "empirical test of Einstein's famous goof"

www.physicsforums.com/showthread.php?t=448241

can we have the equation for the clock at the pole and the equator using the Kerr metric?

Note: if we can't do it please say so, but please no cop outs like "we don't need to", "we ignore rotation because the rotation is slow", we want to do GR here.

No doubt exact solutions representing vacuums outside rotating fluid balls exist, and there is good reason to think that they closely resemble the Kerr vacuum, but such have not been found. The only perfect fluid solution with symmetries resmembling Kerr is the Wahlquist fluid, which is physically unacceptable. In the mathematical sense, models obtainined by matching a realistic rotating perfect fluid ball to an exterior Kerr-like (but not quite Kerr) vacuum region certainly exist, but have not been written down (yet), and may well be impossible to write down in closed form, although nothing definitive appears to have been proven yet.

However, for the vacuum outside the Earth, existing and well-understood approximation methods are perfectly adequate; look for papers by Neil Ashby and others in the arXiv and Living Reviews offering brief descriptions of Post-Newtonian formalism models.

On the more elementary side, there are separate weak-field and slow-rotation-axisymmetric-but-possibly-strong-field approximations which can apply.

As a rule of thumb, introducing rotation often seems to make everything much more difficult in gtr. Experts have some insight into the reasons why but I don't know how to explain these insights in simple terms. But I'll offer this: in general, gravitational fields produced by isolated rotating sources have the property that physically interesting timelike geodesic congruences (world lines of a family of inertial observers) typically have vorticity and thus lack orthogonal spatial hyperslices. This effectively forces more nonzero metric functions depending on more variables, which makes exact solutions much harder to find by elementary means.

Almost all methods of finding exact solutions of (nonlinear) (systems of) PDEs involve exploiting symmetry in the sense of Lie's theory of the symmetries of differential equations (which is the historical origin of Lie theory as in Lie groups and Lie algebras). Relevant pairs of buzzwords include

• independent and dependent variables,
• external and internal symmetries,
• base and fiber spaces

In Lie's theory, a transformation which "preserves the form" of a PDE gives a symmetry; the symmetries to be exploited (if possible) are not limited to metrical symmetries of the underlying spacetime (usually, the base space) but may also include symmetries involving the field variables. For example, when we say that Maxwell's theory of EM is "conformally invariant", we are referring to certain symmetries which have the nature of conformal transformations which when written out very concretely involve both the EM field components and the spacetime coordinates.

I never seem to have the energy to try to provide a painless introduction to Lie's theory, but there are a number of excellent textbooks by authors such as Brian J. Cantwell, Hans Stephani, and Peter W. Olver. Of these, the shortest may be the one by Stephani. Note that Lie's ideas work out differently for systems of ODEs and for systems of PDEs, so the theory falls into two complementary halves. For example, as a rule, to solve a system of PDEs one exploits symmetry to reduce the number of variables; to solve a system of ODEs, one exploits symmetry to reduce the order of the equations. A special case of the "point symmetries" studied by Lie, the "variational symmetries" studied by Noether, is also very helpful, since these involve symmetries of a Lagrangian formulation and lead directly to a "canonical" energy-momentum tensor and to "conserved" fluxes. A generalization leads to connections with methods used in the theory of solitons to construct "solitonic" exact solutions to certain types of wave equations. Interestingly enough, following Chern and others, these ideas can be expressed using curvature (in the abstract mathematical sense).

Coming back to the original topic, if a problem offers insufficiently many external and internal symmetries, closed form exact solutions may be impossible to find. Then one turns to Sobolev spaces and general theory which can establish existence, uniqueness, and some generic properties of solutions not known in closed form. See Robinsion, Introduction to Infinite-Dimensional Dynamical Systems, Cambridge University Press (maybe not the most apt textbook in this context, but it should certainly help convey the flavor).

In "Group of rigid rotations of cube"

www.physicsforums.com/showthread.php?t=447340

Clearly the group has 24 elements by argument any of 6 faces can be up, and then cube can assume 4 different positions for each upwards face.

the group of proper symmetries of the cube is isomorphic to [itex]S_4[/itex] (order 24); the full symmetry group is the order 48 supergroup [itex]C_2 \wr S_3[/itex]; see for example Coxeter, Regular Polytopes, Dover reprint. The subgroup lattice of S_4 is given in a previous BRS post; GAP painlessly computes the subgroup lattice of [itex]C_2 \wr S_3[/itex] which I can give in similar format if desired (31 conjugacy classes of proper nonidentity subgroups).

This is related to the unfinished BRS on the Rubik cube, in a rather general way, via the information theory which in some sense unifies Shannon's information theory and classical Galois theory. Recall that in that theory, the fundamental objects of study are an action by a group and the corresponding lattice of pointwise stabilizer subgroups, where considering various induced actions, combining actions in various ways, &c., ultimately blurs the distinction between pointwise and setwise stabilizers. This is important because most elementary discussions concern "setwise stabilizers" in some action, here the action by S_4 on the faces (apparently).

My book describes the rotations as follows:
3 subgroups of order 4 created by rotation about line passing through center of two faces.
4 subgroups of order 3 created by "taking hold of a pair of diagonally opposite vertices and rotating through the three possible positions, corresponding to the three edges emanating from each vertex."

My trouble lies with the second description, that is, I haven't the slightest idea of what it is saying. Any help?

(What book?...grrr...)

One can consider the action by S_4 (or the supergroup) on faces, edges, vertices, the four vertex-vertex diagonals, the three face-face axes, &c, and on sets of these. Considering various actions by a group is often an easy and efficient way to begin enumerating conjugacy classes of subgroups. In particular:

• In the action on the three face-face axes, the stabilizer subgroup of an axis is C_4, and the action is transitive (any of the three axes can be moved to any other) so these must give a conjugacy class of three subgroups isomorphic to C_4.
• In the action on the four vertex-vertex diagonals, the stabilizer of each diagonal is C_3, and the action is transitive (any of the four diagonals can be moved to any other) so theses must give a conjugacy class of four subgrups isomorphic to C_3.

In the action by S_4 on faces, pairs of opposite faces move in lockstep--- that is, the kinematic closure of one face is the pair consisting of that face and the opposite face, i.e. these two faces share the same stabilizer subgroup. Similarly for pairs of opposite vertices.

Also, any general comments on visualizing symmetry groups would be appreciated, I trouble going beyond dihedral group of order 4.

Maybe the problem is nonabelian groups? S/he can try a book by (I think) R. P. Burns which offers an excellent "workbook" type approach to learning about finite groups. The Schaum Outlines book on group theory by Baumslag and Chandler is also quite readable.

In "quaternions and metric of the 3-sphere"

www.physicsforums.com/showthread.php?t=448298

("well known" ) the angle psi parameterizing the Hopf tori in the Hopf chart or toral chart on S^3 has nothing to do with the latitude angle in the polar spherical chart, so it should not be surprising that these angles have distinct ranges. One way to understand the range is to consider what happens as you slowly increase psi from 0 to pi/2. Another is to draw a tetrahedron in which a pair of opposite edges represent the two degenerate Hopf tori (two Hopf circles). We can imagine deforming the 3-sphere so that all the curvature is concentrated in these two great circles; just identify faces of the tetrahedron appropriately. Then consider how slices "parallel" to the pair of opposite edges (they form a family of parallel rectangles degenerating to the two edges) evolve as you move from one edge to the opposite edge.

Penrose's book The Road to Reality has a very nice picture of the Hopf circles which may suggest how to form the one parameter family of Hopf tori; the parameter is the angle psi the OP is worried about.

It may also help to observe that in a tubular neighborhood of one of the degenerate Hopf tori, the metric closely resembles ordinary cylindrical coordinates, locally in sense of open neighborhood; globally one shoulld imagine cutting the nested cylinders and identifying the circular ends to form nested tori. This gives a metric which is locally exactly the usual cylindrical chart on E^3; the Hopf chart on S^3 is similar, but in the sense of Riemannian geometry, locally (and even globally) gives 3-sphere geometry.

HTH.

Re "What is a Regular Transition Matrix?"

www.physicsforums.com/showthread.php?t=352587

some useful buzzwords are "Frobenius-Perron theory", "ergodic decomposition", "invariant measure", and a very nice undergraduate level introduction to the application to finite Markov chains is in the textbook by Snell et al., Finite Mathematical Structures. There also plenty of other books which give readable introductions.

BTW, "transition matrix" is used in several fields of mathematics to mean several rather distinct notions; the OP should have stated s/he was asking about the usage in the theory of finite Markov chains.

 

[Chris Hillman]

BRS: of balls and bubbles; plus, foundations

In "How does GR slow a homogeneous universe?"

www.physicsforums.com/showthread.php?t=448669

mysearch claims

Basically, there seems to be an assumption that after the Big Bang, the initial expansion of the universe was slowed by gravity. Many sources appear to feel that this is so self-evident that no further explanation is usually given other than a possible passing reference to GR.

I guess s/he is reading mostly popular sources, although s/he does cite one arXiv eprint, because of course this is a serious mischaracterization.

the basic GR premise that supports this conclusion.

I think pervect already had the same idea: s/he should learn about the Raychaudhuri equation, by preference by reading good textbooks rather than asking in PF! The Baez & Dolan expository paper is a good place to start, but a serious student should also study textbooks, IMO.

I think mysearch is struggling to express his desire to formulate and explore some competing models in the framework of gtr, based upon his verbal formulations. Unfortunately s/he runs into serious trouble immediately

The gravitational effects are assumed to align to the logic of Newton’s Shells... The force on an object m at radius=r>R, i.e. outside this volume, is subject to the normal inverse square law 1/r2 based on its distance r from the centre of the homogeneous volume.

I don't see much hope of making sense of this in the context of gtr!

S/he sketches his first model as envisioning:

a large spherical volume of homogeneous density, radius=R, exists within an infinite and absolute vacuum. This homogeneous volume has an effective mass and a centre of gravity.

This appears to mean something like a homogeneous dust ball surrounded by an asymptotically flat vacuum region. But then of course according to either gtr or Newton's theory, such a dust ball cannot remain static but must collapse. In context, presumably s/he meant that the dust ball is initially expanding, and then the expansion will be slowed by the gravitational self-attraction of the ball, in either gtr or Newton's theory. Assuming this interpretation, a nice simple gtr model meeting his verbal requirements would be the time reversal of the Oppenheimer-Snyder dust ball. This consists of an expanding dust ball (homogeneous time-varying density, zero pressure perfect fluid) matched across an expanding spherical surface to an exterior vacuum region, which is a portion of the Schwarzschild vacuum exterior with the appropriate mass parameter. The world lines of the dust particles form a uniformly expanding geodesic congruence (the ones near the surface of the ball are comoving with the surface), and this congruence has zero vorticity, so spatial hyperslices exist and turn out to be locally isometric to E^3 (vanishing three dimensional Riemann tensor).

S/he sketches his second model as envisioning:

Also assumes a homogeneous density, but now its volume conceptually extends to infinity.

This is could be compatible with an even larger array of gtr models; one fairly simple possibility would be a vacuum bubble inside an expanding FRW dust with E^3 hyperslices.

Since s/he says s/he is interested in better understanding gtr rather than shooting down modern cosmology, an even better model might be a hybrid consisting of an expanding shell of dust matched inside to an expanding vacuum region (portion of Minkowski vacuum) and outside to an asympotitically flat vacuum region (portion of Schwarzschild vacuum exterior region).

S/he asks about "a centre of gravity" but I don't think this really makes sense in gtr, because of the mathematics of curved manifolds leads to

• the notorious difficulty of defining sensible notions of "distance in the large"
• the notorious difficulty in averaging almost anything in any coherent sensible way

Because of the homogeneity s/he requires, the local versus global distinction is also relevant. Clearly there is no local (in sense of local neighborhood) "center of gravity" inside any FRW dust region! I suppose one could try to argue from the obvious nested spherical shells in our "dust ball at a time" that there is a global center. Note that one could choose any point inside the dust ball and make another family of distinct nested spherical shells; this would not include the surface of the ball, but this would need to be pointed out in order to identify a unique family of nested spherical shells.

An accurate big bang theory would have to explain why these burst started and stopped for several periods without matter.

I hope that won't pass without correction because this poster could learn something here: the standard hot Big Bang theory does not attempt to explain what happened before a time certain; its success consists of success in explaining (much) of what happened after that time.

Does GR radically disagree with this simplistic assessment of the underlying physics?

The conceptual foundation of gtr is radically from that of Newtonian gravitation, so the only possible short answer, I think, is "yes". The conceptual differences have technical consequences which cannot be ignored: the treatment of "conservation of energy", thermodynamics, and other core topics is significantly different in crucial ways.

To forestall the question: "why then does Newtonian gravity agree so closely with gtr?", one answer would be "in general, it agrees closely only in the weak-field slow motion limit; in addition, certain very simple and highly simplistic models may bear some points of naive agreement because there are only so many simple formulas".

Example: sometimes people ask why both Newtonian gravity and gtr give m/r^3 for the tidal accelerations outside a nonrotating isolated object of mass m. Well, that "r" is problematic; in Newtonian gravity there is no doubt what it means but the meaning has to carefully explained in gtr. So to some extent points of agreement can be illusory, or at least, there is almost always more to the story than simply stating an alleged "unambiguous agreement betweeen the predictions of N.g. and gtr".

Suddently s/he brings up dark energy, which looks rather like an ambush. If s/he really wants to understand gtr better, s/he should begin by studying a gtr textbook and in particular the best understood models. Leave speculations about dark energy out of it until one knows enough to begin to understand how such ideas fit into the big picture.

Re

www.physicsforums.com/showthread.php?t=448681

there has been a lot of study of what one might mean by "total energy of the universe". It turns out to be far from easy to define a useful general notion although there are obvious choices in certain restricted examples, including the situations Hawking has in mind (I guess, since the OP didn't cite or quote enough to be sure). It would be fair to say that the majority of researchers do not accept Hawking's occasional insistence on limiting oneself to the restricted situations he has in mind. If my guess is correct about what Hawking was referring to, I don't think the question of whether dark energy exists and if so what is properties are is relevant to the issue of whether the restrictions Hawking needs should be imposed. In fact, if I guessed right about what Hawking was discussing, the issue long predates the discoveries which have led to the inferences that

• dark matter possibly exists,
• dark energy possibly exists.

Re "Is mathematics a science?"

www.physicsforums.com/showthread.php?t=447994

I would say pretty much what HallsofIvy said, maybe a bit more:

• the defining characteristic of science is the scientific method,
• the defining characteristic of the scientific method is the comparision of experimental/observational data with quantitative theoretical predictions,
• mathematics can be defined as "the art of precise reasoning about simple phenomena without getting confused" (by the ambiguities of nonquantitative language, for example); as such, it provides the necessary foundation for everything in science,
• to be sure, mathematics is ultimately more about precisely defining and understanding abstract structures than about simple arithmetic, but ultimately, the mathematical ideas most likely to be used in science will involve real or complex numbers in some way,
• to be sure, mathematics is not only the most powerful and practical tool in the intellectual's arsenal, but is possessed of great beauty, at least as perceived by those sufficiently capable of abstraction,
• in mathematics as in any highly intellectual field, there is ample opportunity for individuals to stamp their personal style on a body of work; in particular, some arguments are widely agreed to be more beautiful than others,
• in particular, statistics (a highly quantitative field, even when dealing with "categorical data") is clearly mathematical in language and content, and all experimenters must and do use statistics to interpret the meaning of their results,
• in the philosophy of statistics, the question "what is a probability, that we should be mindful of it?" has been characterized as the greatest unsolved problem in math/stat/sci, a point which was emphasized by one of the very greatest mathematicians of the last century, Andrei Kolmogorov (who early in the century put the theory of probability on a theoretically sound foundation, measure theory, but this is not the same thing as answering the question just described!).

MATHEMATICS IS NOT ABOUT REALITY!

Is Shayan quoting this alleged viewpoint in order to repudiate it? I can't tell.

Mathematicians only care about their axioms. As long as everything is consistent, then it's good.

I don't know what "Shayan" means by "reality" but I do know that since Newton, many, even most, of the mathematical problems which have been regarded by mathematicians as the most important problems, have been inspired by scientific, engineering or otherwise practical problems involving "the real world" in various ways. Further, many mathematicians, even most, are inspired by the prospect of comparing theory with experiment. Certainly that is true of everyone who functions as an applied mathematician (physicists, computer scientists, economists, even political scientists).

Mathematicians don't care about the realistic applications of complex numbers...

Really? S/he should search John Baez's UCR website. (JB is a mathematician by training.)

And people solved that polynomials because they were like games,

Really? There is a kernel of truth in that regarding Cardano and his contemporaries, but micromass should compare Newton's writings about polynomials (including solving them--- much more than just "Newton's method"). Newton himself emphasized applications of his work, and this is typical of the modern viewpoint in mathematics.

Also, ditto Gerald Edgar and Tom Gilroy, I think.

While no-one has yet mentioned the views of Hardy, I think they are implicit in several of the more passionate but less well-informed comments. The fact is, I think it is fair to say that the vast majority of mathematicians believe that Hardy's views have been discredited. Indeed, one remarkable phenomenon in the last 50 years has been the virtual fusion of some rather "pure" mathematics with some of the most popular lines of investigation in mathematical physics. It is particularly ironic that number theory has turned out to be so practical, even critical for our modern technoworld. This refusion of mathematics and physics has compelled a return to the mainstream view in Newton's day, which did not clearly distinguish between these subjects. (But "natural science" in the Newtonian sense has not been what modern practioners mean by "science" for two centuries at least.)

In "lie subgroup"

www.physicsforums.com/showthread.php?t=448557

the pullback of a closed set under a continuous map (its components are the given real-valued functions) is topologically closed, so the subgroup is a closed subgroup of a Lie group, and thus a Lie subgroup.

 

[Chris Hillman]

BRS: Vaidya-Tikekar ssspf

Re "Tikekar superdense stars and interior metrics"

www.physicsforums.com/showthread.php?t=448324

FunkyDwarf asks about a particular static spherically symmetric solution (ssspf) in gtr, the Vaidya-Tikekar ssspf (1982), referring to the original paper (which I have read) and two more recent ones (which I have not read).

Some general remarks might help:

• all ssspf solutions are known in more or less closed form,
• they can be nicely expressed in various "canonical" forms including
  • Wyman-Lake form (by far the oldest)
  • BVW form
  • Martin-Visser form
  • Rahman-Visser form (Shahinur Rahman, not Sabbir);
  some of this nice work is quite recent; see eprints coauthored by Visser in the arXiv,
• the Martin-Visser form admits a remarkable internal symmetry which enables one, given a central density and pressure, to choose a new central pressure while keeping the old density; this "pressure change transformation" (a point symmetry in the sense of Lie) gives new (geometrically and physically distinct) solution, so from anyone solution in MV form one immediately obtains infinitely many others in this way,
• regardless of canonical forms, all ssspfs can be written using Schwarzschild like coordinates, or "isotropic" coordinates, or in various other ways, but those are the two most popular and you can probably guess what they are!; below I'll write r for the Schwarzschild radial coordinate,
• all good ssspf solutions can be matched across the surface (r value where pressure vanishes) to an exterior vacuum which is a portion of the Schwarzschild vacuum with the appropriate mass parameter,
• all ssspf solutions in gtr must obey the Buchdahl limit: the surface must be at r_s > 9/8 2m,
• all ssspf solutions have the property that the congruence of world lines of fluid elements are nongeodesic but form a vorticity-free congruence, and the timelike unit vectors are parallel to a timelike Killing vector (hence static spacetime, since there exists a vorticity-free timelike Killing vector field)
• however you write your ssspf Ansatz, physically there are two variables (density and pressure) so geometrically there are two metric functions which depend only on r; one will generally be completely determined from the other by solving a linear ODE, and the "master" function will generally be determined by solving a second order ODE, so we should expect two free parameters,
• wrt a frame comoving with the fluid particles, the Einstein tensor is automatically diagonal and G^(33) = G^(44) is automatic, so the only condition is G^(22)=G^(33); this gives a second order ODE for your metric function,
• the orthogonal hyperslices always resemble S^3 at the center r=0; graphically, if you plot the components of the three-dimensional Riemann tensor wrt the obvious frame field, r_(2323) = r_(2424) falls off more rapidly than r_(3434) as you head outwards from r=r0,
• graphically, good ssspf solutions generally have positive pressure and density, pressure falling to zero at the surface where the density will usually be positive, i.e. G^(22) = G^(33) = G^(44) is everywhere non-negative and less than G^(11) and falls to zero at the surface,
• most closed form ssspf solutions will be written using two parameters, as already noted; in principle the metric functions can be rewritten so that the parameters are central density and central pressure, and the solution may look much simpler when written this way, or much more complicated; typically the surface radius r_s will be a function of central density and pressure; sometimes a third parameter appears which simply sets a "standard radius" for computing the relative gravitational time dilation of observers riding with fluid elements,
• plugging in arbitrary values for the parameters may very well lead to negative density and/or negative pressure, which must usually be rejected if we are making a stellar model!,
• most ssspf solutions are not relativistic polytropes and most are not consistent with thermodynamical expectations (don't have an obvious notion of surface temperature, don't have a physically sensible equation of state)

"Good" rules out cases which violate energy conditions and some exceptional cases in which there is not surface at any finite r value. Even after restricting to "good" solutions, there is the problem of finding parameter values which give reasonable values of central density/pressure and surface radius for a given type of star; if there is no equation of state in view (which is usually the case), there is generally no reason to think that any values will give impressive models, so serious models are generally constructed numerically as described in MTW.

The Vaidya-Tikehar ssspf conforms to these expectations but is quite a bit more complicated than many other ssspf solutions such as the Tolman IV ssspf.

(For values of the central density and pressure corresponding to reasonable crude guesses for neutron stars, the Tolman IV solution actually does give values for surface radius which are reasonable for a nuetron star, and it has an equation of state, but a rather wacky one, and AFAIK the Tolman IV model is mainly of pedagogical value, and the other exact ssspf solutions known in simple closed form are AFAIK no better than Tolman IV, although some may give better approximations of other types of stars--- IIRC, the Tolman IV solution doesn't seem to work very well for ordinary stars, which is rather interesting in itself.)

In the original paper, the authors

• propose the desideratum that the three-dimensional Riemann tensor of the orthogonal hyperslices should have a particular form; in this form, the parameter satisfies K < 1 and the case K=1 gives E^3 slices while K=0 gives S^3 slices, with the other cases deformed three-spheres as described above (including the fact that the geometry approaches S^3 geometry as r->0+),
• write down the Schwarzschild chart for their ssspf, with one undetermined function of r,
• transform variables and solve the condition G^(22) = G^(33) for their unknown metric function \nu,
• specialize to the case K=-2, in which the slices have particularly simple three-dimensional Riemann tensor

FunkyDwarf thinks he spotted an error but I don't see the problem and the K=-2 solution given by the authors is certainly an exact ssspf.

By choosing values for their parameters one can find solutions which obey the energy conditions, e.g. R=1, A=3, B=5 works with r_s ~ 0.504. Plugging in numbers might show these values are physically out of range for a neutron star.

There is nothing that I see in Vaidya and Tikekar 1982 which implies that their ssspf neccesarily models "superdense stars", whatever that means (neutron stars?); they simply produced yet another exact solution of this kind. Some of the more recent arXiv eprints give this solution in the BVW form, as I recall.

Can't resist touting the many virtues of the new canonical forms mentioned above. Just as an example, the Martin-Visser form is (in Schwarzschild chart)
[tex]
ds^2 =
-\exp \left( -2 \int_r^\infty g(\bar{r}) d\bar{r} \right) \; dt^2
\; + \; \frac{dr^2}{1-2m \, r^2}
\; + \; r^2 \, d\Omega^2
[/tex]
where m is a function of r satisfying
[tex]
m^\prime =
\frac{-2r}{1+r \, g} \; (g^\prime + g^2) \; m
+ \frac{(g/r)^\prime/r + g^2/r}{1+r \, g}
[/tex]
Here, the ingenious idea of Martin and Visser was to use as metric function
[tex]
m = M/r^3
[/tex]
where M is also a function of r, giving the total mass inside that r, so that as r approaches r_s, M approaches the mass parameter used in the Schwarzschild exterior. It turns out that this metric function m is a very clever choice.

We can plug in
[tex]
G^{11} = 8 \pi \, \epsilon, \; \;
G^{22} = G^{33} = G^{44} = 8\pi \, p
[/tex]
and eliminate g and m. We find
[tex]
g = \frac{-p^\prime}{p + \epsilon}, \; \;
m = \frac{-p^\prime/r - 4 \pi \, p \,( \epsilon + p)}
{\epsilon + p - 2 r \, p^\prime}
[/tex]
where the mass-energy density \epsilon satisfies an Abel equation
[tex]
\epsilon^\prime =
A \, \epsilon^3 + B \, \epsilon^2 + C \, \epsilon + D
[/tex]
where the coefficients A,B,C,D depend upon the pressure. Different choices of solution for epsilon in terms of p, up to two constants, gives different families of ssspf solutions, e.g. the Vaidya-Tikekar family. Finally, choosing central values for \epsilon and p determines a specific solution which can used as a model of a nonrotating isolated static fluid ball.

Here, a "good" solution should satisfy
[tex]
\epsilon > 0, \; \;
p > 0, \; \;
p^\prime < 0, \; \;
p + \epsilon > -2 r \, p^\prime > 0
[/tex]

You can plug in the polytrope conditions (oddly, haven't yet seen this done in the literature)
[tex]
\mu = \mu_0 \, (T/T_0)^n, \; \;
p = p_0 \, (T/T_0)^{n+1}, \; \;
\epsilon = \mu + n \, p
[/tex]
where \mu is the mass-density, p is the pressure, and \epsilon is the mass-energy density (including the mass-energy due to nonzero temperature of the matter). Here, n is a constant, the adiabatic index. Notice that
[tex]
M^\prime = 4 \pi \, r^2 \, \mu
[/tex]
Then we have
[tex]
g = \frac{r \, \left( m + 4 \pi \, p_0 \, (T/T_0)^{n+1} \right) }
{1-2m \,r^2}
[/tex]
This is a little more elaborate than a just plain ssspf because we have another variable, the temperature, and we determine everything in terms of the central density, pressure, and temperature.

Now you obtain some interesting and amusing expressions for various quantities. For example:

• the gravitational acceleration of the fluid element at a given r value is:
  [tex]
  \nabla_{\vec{e}_1} \vec{e}_1 =
  \frac{m r \, (1 + 4 \pi \, p)}{\sqrt{1-2m \, r^2}} \; \vec{e}_2
  [/tex]
• the tidal tensor is given by
  [tex]
  \begin{array}{rcl}
  E_{22} & = & -2m + 4 \pi \, (\epsilon + p)
  = -2m + 4 \pi \, (T/T_0)^n \;
  \large( \mu_0 + (n+1) \, p_0 \, (T/T_0) \large) \\
  E_{33} = E_{44} & = & m + 4 \pi \, p =
  m + 4 \pi \, p_o (T/T_0)^{n+1}
  \end{array}
  [/tex]
  which shows how the stresses inside the fluid depend on the temperature at each point,
• the three-dimensional Riemann tensor of the "constant time" spatial hyperslices is given by
  [tex]
  r_{2323} = r_{2424} = -m + 4 \pi \, \epsilon, \; \;
  r_{3434} = 2m
  [/tex]

As we approach the center r=0, the acceleration vanishes and

• the tidal tensor approaches
  [tex]
  E_{22} = E_{33} = E_{44} = \frac{4 \pi}{3} \, (\epsilon_0 + 3 p_0)
  [/tex]
• the Riemann tensor of the hyperslices approaches
  [tex]
  r_{2323} = r_{2424} = r_{3434} = \frac{8\pi}{3} \, \epsilon_0
  [/tex]

Just one problem, as you may have already noticed: the polytrope assumptions ensure that either there is no surface (where sphere r=r_s where p(r_s) =0)! So the polytrope assumption isn't neccessarily what we want.

 

[Chris Hillman]

BRS: stress-energy tensor, Regge-Wheeler, Dirac, & quartic eqns,

God save the C U P

(CUP = Cambridge University Press)

You know how the U.S. Congress opens each session? Even though most of the members are probably stone-cold atheists in their innermost cynical hearts? (And as any Freudian can see, have apparently not quite separated from either the God Father or the Mother Country.) In similar faux-pious spirit, I should probably open each BRS post with this incantation.

Someone recently advised me to develop the habit of enumerating my potentially offensive remarks, and I see I have already Potentially Offended:

• the faithful
• Freudian psychiatrists
• the Congress
• the mob
• the English royal family
• Frenchmen who think Frenchmen should sing in French
• the NSA
• God Himself, assuming a notoriously lacking existence theorem.

And I haven't even gotten started in this post!

Fear of politically-motivated retribution aside, what prompts me to such sentiment? Well, all the truly useful math and physics books come from CUP. Well, not quite all, but all the ones anyone but Croesus can afford. Their London Mathematical Society Student Texts (LMSST) series is particularly excellent in terms of contemporary value. And fans of differential geometry will be particularly delighted by their recent reprint of William L. Burke's Applied Differential Geometry, which I urge every SA/M to cite in PF whenever someone asks "what the bleep is a (one-form) (tensor), anyway?"

Some Dover reprints, including some of their new Phoenix series, are also really great books, but alas I have to say that on balance they still tend more toward books which are not as relevant today, although there are some brilliant exceptions such as Flanders's classic on differential forms. I plan to mention some more below.

It's rather staggering that I began making less than laudatory remarks about Dover back when none but the odd visionary envisioned the possible Death of the Book in our lifetimes, but sadly, I now find that I must reverse myself and beg SA/Ms to impoverish themselves by buying Dover, simply in a last ditch attempt to save the book itself. One of the oldest, and still the best, form of information storage and retrieval.

Don't even get me started about such troubling issues with e-books and browsing-in-the-cloud as the question of who owns an e-book (hint: not you!) and a variety of (you guessed it) privacy and computer security issues. Let's just say that I think SA/Ms should avoid reflexively citing Google books at every opportunity, because its possible to give a coherent argument that this is contrary to the medium term interests of scholarship itself, and thus, science itself.

And now, courtesy of the Department of the Awkward Segue:

Discuss: the sudden uptick in sophisticated questions may be due to the physorg prize recently awarded to PF.

Re "Stress-energy tensor"

www.physicsforums.com/showthread.php?t=449064

wrt a frame field adapted to the EM or KG field, and denoting by [itex]\epsilon[/itex] the energy density:

• the stress-energy tensor of a non-null EM field takes the form:
  [tex]
  T^{ab} =
  \epsilon \;
  \left[ \begin{array}{cccc}
  1 & 0 & 0 & 0 \\
  0 & -1 & 0 & 0 \\
  0 & 0 & 1 & 0 \\
  0 & 0 & 0 & 1 \\
  \end{array} \right]
  [/tex]
  this applies to the magnetic field of a bar magnet, and if you like the "field-lines" picture, then intuitively speaking, "the magnetic field lines repel each other but also try to contract along their own length"; the field seeks an equilibrium configuration balancing these desiderata; the desire of each field line to contract along the spatial direction aligned with the field line, while repelling all neighboring field lines, is clearly visible in the (-1,1,1) structure seen in the spatial components on the diagonal,
• the stress energy tensor of a null EM field (vanishing principle Lorentz invariants; EM radiation) takes the form:
  [tex]
  T^{ab} =
  \epsilon \;
  \left[ \begin{array}{cccc}
  1 & \pm 1 & 0 & 0 \\
  \pm 1 & 1 & 0 & 0 \\
  0 & 0 & 0 & 0 \\
  0 & 0 & 0 & 0 \\
  \end{array} \right]
  [/tex]
• a massless Klein-Gordon scalar field typically has spacelike gradient in some "static region" and timelike gradient in some "dynamic region", and
  • in a region where the scalar field has timelike gradient, the stress-energy tensor takes the "stiff-fluid" form:
    [tex]
    T^{ab} =
    \epsilon \;
    \left[ \begin{array}{cccc}
    1 & 0 & 0 & 0 \\
    0 & 1 & 0 & 0 \\
    0 & 0 & 1 & 0 \\
    0 & 0 & 0 & 1 \\
    \end{array} \right]
    [/tex]
  • in a region where the scalar field has spacelike gradient, the stress-energy tensor takes the form:
    [tex]
    T^{ab} =
    \epsilon \;
    \left[ \begin{array}{cccc}
    1 & 0 & 0 & 0 \\
    0 & 1 & 0 & 0 \\
    0 & 0 & -1 & 0 \\
    0 & 0 & 0 & -1 \\
    \end{array} \right]
    [/tex]
  • on the boundary between two such regions, where the scalar field has null gradient, the stress-energy tensor takes the same form as a null EM field.
  Well-known static examples of mcmsf solutions include the Janis-Newman-Winacour mcsmf and the Ellis-Bronnikov (Morris-Thorne) mcsmf, in which the scalar field has a spacelike gradient. The Roberts mcmsf has a static exterior region where the scalar field has spacelike gradient and past interior and future interior regions which are dynamic, where the scalar field has timelike gradient.

Recalling that to take the trace, we first form [itex]{T^a}_b[/itex] and then contract, the EM field always makes a traceless contribution to the stress-energy tensor, while the massless KG field makes a contribution with nonzero trace except where the gradient is null.

To avoid possible misunderstanding: choose any event and boost/rotate the frame there. In the new frame, the energy-momentum tensor will most likely not assume the above forms, which are only valid for suitably adapted frame fields. But scalar invariants of the EM tensor will of course be the same no matter what frame field you use.

If you use a coordinate basis rather than a frame field (ONB in the language of MTW), then you are unlikely to find any chart in which the stress-energy tensor looks as simple as above, unless you are working with Minkowski spacetime (i.e. str, not gtr).

FWIW, the field equations are

• for EM, the Maxwell equations written in formalism suitable for curved spacetimes, e.g. using differential forms (plus the Hodge star):
  [tex]
  dF = 0, \; \; d{{}^\ast\!F} = 4 \pi \, J
  [/tex]
• for massless KG, the curved spacetime wave equation
  [tex]
  \Box \phi = 0
  [/tex]

In "Regge Wheeler Equation"

www.physicsforums.com/showthread.php?t=449121

When we perturb schwarzschild metric with linear perturbation we get Regge-Wheeler equation. Which is schrodinger equation for spin 2 particles. Gravitational waves also have spin 2. Is there a connection?

Yes, the dynamic perturbations will describe gravitational radiation propagating near an otherwise static spherically symmetric gravitational field in a vacuum region (i.e. Schwarzschild vacuum exterior), and the QFT inspired shorthand slogan for the tensorial nature of gravitational radiation in gtr (strictly: weak-field gtr) is "spin-two". For more detail the OP should see chapter 4 in Chandrasekhar, Mathematical Theory of Black Holes.

Re "Dirac brackets and gauge in special relativity"

www.physicsforums.com/showthread.php?t=448829

the discussion in Lawrie, Unified Grand Tour of Theoretical Physics, should be perfect for the OP.

Re "Solving for g_φφ=0 in charged/rotating BHs"

www.physicsforums.com/showthread.php?t=449018

stevebd wants to solve for r the equation
[tex]
\frac{R^4}{\Delta}=a^2 \sin^2 \theta, \; \;
\hbox{where}
R=\sqrt(r^2+a^2), \; \;
\Delta=R^2-2Mr+Q^2
[/tex]
He didn't say that he is looking at the Kerr metric written in some chart (presumably ingoing Eddington or ingoing Painleve--- if he wants a good answer s/he should write out the line element intended so that we at least know what "r" might be!). Fortunately, I can confidently guess that he is trying to find the condition that the coordinate vector [itex]\partial_\phi[/itex] be null. Then the obvious circles are closed null curves, and such do indeed exist in the "deep interior" of the Kerr vacuum.

(But not to worry because there are independent good reasons to think realistic models of black hole interiors as treated in gtr do not look that the Kerr vacuum in the "deep interior", even though the exterior should closely resemble Kerr vacuum!).

isn't it a straightforward quadratic equation in R^2?

I think I know what Tim was thinking, and for a moment I made the same mistake, but no, because plugging
[tex]
R=\sqrt(r^2+a^2), \; \;
\Delta=R^2-2Mr+Q^2
[/tex]
into
[tex]
R^4 = \Delta \, a^2 \sin^2 \theta
[/tex]
gives
[tex]
(r^2+a^2)^2=a^2 \, \sin(\theta)^2 \, (Q^2-2Mr+r^2+a^2)
[/tex]
Thus Maxima:

(%i1) subst(R^2-2*M*r+Q^2, Delta, R^4=a^2*sin(theta)^2*Delta);
(%o1) R^4=a^2*sin(theta)^2*(R^2+Q^2-2*r*M)
(%i2) subst(sqrt(r^2+a^2),R,%);
(%o2) (r^2+a^2)^2=a^2*sin(theta)^2*(Q^2-2*r*M+r^2+a^2)
(%i16) expand(%);
(%o3) r^4+2*a^2*r^2+a^4=a^2*sin(theta)^2*Q^2-2*a^2*r*sin(theta)^2*M+a^2*r^2*sin(theta)^2+a^4*sin(theta)^2
(%i3) factor(%);
(%o3) (r^2+a^2)^2=a^2*sin(theta)^2*(Q^2-2*r*M+r^2+a^2)

So the OP is looking for real roots in an appropriate range for a fourth order polynomial. The first thing is to check how many there are, and he can do that using Sturm chains. Note this requires choosing numerical values for the other parameters. Then he can apply the formula for the roots of a quartic to find the roots, then he can choose the particular root he needs. The answer will probably take about a page to write if he writes small. But he can use perturbation theory to find a useful approximation. Even better, he can use perturbation theory from the start and seek an approximate but memorable answer rather than an exact but useless since over complex answer.

There are many wonderful books which offer brief but useful introductions to the elements of perturbation theory, including:

• Wilf, Mathematics for the Physical Sciences, 1962, available as Dover reprint, stylish and in good taste in terms of what the author chooses to discuss (one notational quirk: [itex]c/a+b[/itex] for [itex]c/(a+b)[/itex] appears in-line, a not uncommon notation before 1920 or so, but rather odd in a 1962 book and sure to confuse modern students),
• Richard Bellman, Perturbation Techniques, 1966, available as Dover reprint; even more stylish!,
• Simmond and Mann, A First Look at Perturbation Theory, 1986, Dover reprint; not as stylish but it has a chapter on polynomials which will get the OP where he wants to go,
• Richards, Advanced Mathematical Methods with Maple, CUP; one of the very best math methods books, and if you use Maple, definitely the one to obtain,
• E. J. Hinch, Perturbation Methods, CUP, excellent for DEs.

All but the last book discuss analytic (i.e. "given by a symbolic formula", not "real/complex analysis") approximations to roots of polynomials, and all discuss numerous applications to ODEs. There not as much overlap as you might guess due to the richness of the subject, however.

Wilf's book also discusses Sturm chains and many other useful things; I have used Sturm chains in some of my old posts to locate real roots of (for example) the "effective potential" for the Schwarzschild-de Sitter lambdavacuum. Sturm's technique is quite useful and well worth learning.

Dover books: I don't think they'll help anyone learn superstring theory, but perturbation theory is never going to go out of style, so Dover books on perturbation theory are not likely to become irrelevant any time soon.

 

[Chris Hillman]

BRS: multiple confusions about FRW models; plus, Maxwell's mechanical model

Re "Conditions for spacetime to have flat spatial slices"

www.physicsforums.com/showthread.php?t=446589

this thread certainly seems to have been going in circles since JDoolin got involved, and I confess I lack the energy to try to do more than skim it. In the sequel, I think my comments may partially duplicate things George Jones, Ben Crowell, Lut Mentz, and some others have already said to JDoolin, but most are I think new.

Originally, I think Peter Donis was trying to ask something like this: when does a spacetime admit a family of spatial hyperslices which are all locally isometric to E^3? Or even: when does a (vacuum) (perfect fluid) model in gtr admit an irrotational timelike congruence whose orthogonal spatial hyperslices are locally isometric to E^3? If so, there are good partial answers in the research literature; see "the exact solutions book" coauthored by Stephani for starters.

Due to differences between Lorentzian and Riemannian geometry, it is not possible to diagonalize every symmetric matrix using Lorentz transformations (in the tangent space to any point, in a Lorentzian manifold) [itex]S \rightarrow \Lambda^t \, S \, \Lambda[/itex], although it is possible to diagonalize every symmetric matrix using orthogonal transformations (in the tangent space to any point, in a Riemannian manifold) [itex]S \rightarrow O^t \, S \, O[/itex]. See for example the book by Barrett O'Neill.

Then, JDoolin started talking about lotsa stuff which seems to arise from various misconceptions about curved manifolds in general and FRW models in particular. It is probably no coincidence that he hints that he is a devoted fan of the non-standard (and possibly even incorrect) approach of Lewis Epstein. (I recall once looking at the book in question but can't recall anything now.)

Just one example indicating serious confusion:

I'm a little troubled that the Robertson Walker chart is either mapping coordinate time or proper time, depending on who gives me an answer.

I have no idea what he might mean by that, but it would make no sense if he were using "mapping", "coordinate", or "proper time" in their standard senses.

You're sometimes saying that the vertical coordinate in the Robertson-Walker diagram represents the proper time of particles. Other times, you're acting like it is the actual time passed by the central observer...The only place where those two definitions can be shared is along the single line representing the worldline of the "stationary" particle.

Well, proper time measured by an observer between two events on his world line is the interval integrated along his world line between those two events.

Lut Mentz mentioned a (valid) coframe field which defines the FRW dust with E^3 hyperslices orthogonal to the world lines of the dust particles, in which differences in the t coordinate does give proper time intervals as measured by any observer riding on a dust particle. So

What Mentz114 is calling the Painleve chart for the FRW spacetime is a different coordinate system used to describe that spacetime, in which the metric looks quite different than it does in the Robertson-Walker coordinate system. In this coordinate system, the "time" coordinate t does *not* directly represent the proper time of "comoving" observers (at least, I don't think it does based on looking at the metric--Mentz114, please correct me if I'm wrong).

Lut is correct; Peter is not. A Painleve type chart is distinguished by the existence of an irrotational timelike geodesic congruence whose orthogonal hyperslices are "nice", even locally flat (locally isometric to E^3), such that differences in the time coordinate corresponds to proper time intervals as measured by any of a certain family of inertial observers--- the ones whose world lines are the integral curves of the irrotational timelike geodesic congruence just mentioned. IOW, the t=t_0 hyperslices are the orthogonal hyperslices of our irrotational timelike geodesic congruence, and these slices are locally flat (or, in a generalized notion of Painleve chart, otherwise "nice").

It has to be proper time that he's talking about, because, he then proceeds to do a Galilean Transformation on the diagram.

Part of the problem seems to be that JDoolin doesn't yet understand that "proper time" makes no sense unless referred to a timelike congruence of world lines. No doubt he is thinking of the proper time measured by observers comoving with the dust particles, but even so, by omitting the qualifiers I suspect he is confusing himself.

proper time (OF A WORLDLINE) is an invariant quantity, but proper time is NOT A COORDINATE. Coordinates are contravariant; not invariant.

Aha, this is clearly a specific confusion. There are certainly plenty of coordinate systems such that differences in time coordinate correspond to proper time interval measured by an observer riding on one integral curve of a certain timelike congruence (not neccessarly a geodesic congruence). Then, coordinate time intervals certainly do correspond to proper time intervals as measured by observers having the specified world lines. So JDoolin just confirmed my guess about one of his underlying confusions: a clear example where sloppy writing permitted sloppy thinking, which prevented his making progress.

A coordinate is simply a strictly increasing (real valued scalar) function defined on some open neighborhood U of some manifold. That is, a function z such that dz is nonzero everywhere on U. If we have another such function y, and if the two-form [itex]dy \wedge dz[/itex] is everywhere nonzero on U, then our two coordinates form a "partial net" such that the integral curves of [itex]\partial_y, \, \partial_z[/itex] are never tangent in U. (See the nice discussion in Hilbert and Cohn-Vossen, Geometry and the Imagination.) This is the generic situtation, in a sufficiently small U. Continuing, we can add more coordinates until we have a local coordinate chart on U, or some smaller neighborhood contained in U. Then the strictly increasing property means that the n-tuple of values of the coordinates uniquely labels each event in U. That's all there is to it.

The specific example I used, that of FRW spacetime, *does* have the property that a single coordinate patch can be used to cover the entire spacetime

True.

The Effect of the Lorentz Transformations are essentially directly proportianal to distance in space and time. i.e. If you Lorentz Transform an event that is 2 light years away, the effect will be roughly twice as much as if you Lorentz Transform an event that is 1 light-year away...If you do a Lorentz Transform on an event that is a billion light years away, the effect is roughly a billion times as much as if you do an LT on an event that is 1 light year away.

This strongly suggests to me that another fundamental problem is very likely that JDoolin has never mastered the geometry of linear transformations. He seems to vaguely grasp part of the meaning of "linearity" but he clearly does not understand that the Lorentz group consists of many linear transformations which have rather distinct geometric properties, e.g. rotations versus boosts versus loxodromic transformations.

Check out the discussion page for the Milne Model, because there are some things there that came from the actual book. When I tried to put actual quotes from Milne in the main article, they were removed.

Oh gosh. Well, not to defend anything which may or may not go on in WP (so far we have only JDoolin's side of this story), but as a rule, encyclopedia articles not about "the history of X" are not concerned with what historical figure F said about X 100 years ago, but about anything in modern textbooks attributed to F (because derived from what F actually said) which may be relevant to modern understanding, taking account of the big picture.

In general, in my experience, mathematically weak students (often autodidacts) often decide that "reading the masters" will make up for studying modern textbooks. But when Chandrasekhar and others urge us to read the masters, they really mean that the most mathematically capable students may not require spoon feeding from modern textbooks, but by studying the old masters and occasionally attempting exercises in modern textbooks as a reality check, may efficiently reinvent anything they need to reach the current frontiers. I would modify that slightly: postdocs with time and effort can benefit from reading the masters, but ambitious Ph.D. students need to reach the frontiers ASAP and they'd be well advised to stick to the textbooks and (when they know enough) the research literature.

Time and again I see well-intentioned autodidacts go down this "Great Books of Science" path, which IMO limits them to pseudo-intellectualism, which is pretty sad, since none of this stuff is so very hard if you approach it in the right manner.

Also, not to denigrate Milne, who was certainly a leading astronomer in his day who made important contributions, but his writings on gtr-related stuff are nowhere near as important or relevant as the books by MTW and Chandrasekhar, so anyone wishing to "read the masters" should at the very least know who the masters have been!

Pardon me, but does the current model really "FIT" that well? We have no real explanation for inflation. We have no dark energy. We have no dark matter. We have a theory that is inconsistent with quantum mechanics. But we have an equation that matches up really well.

Well, this is a rather childish view. Anyone who knows anything about real science knows that when we approach the frontiers of science, there are always far more questions than answers. What should impress outside observers are the facts that

• cosmologists have a theory which explains so much with so little,
• cosmologists clearly recognize a large number of issues where they know they can't yet say very much with very much confidence.

The thing is, I can show you a conformal mapping between the Milne model and the Robertson Walker Metric, which preserves the speed of light and has exactly the same events, if you're interested. All the same variables are there. All the same events are there, except the singularity is transformed into a lightcone in Milne's version, and it's turned into a plane in the "comoving matter" version.

Not implausible since FRW dusts and Minkowski spacetime ("Milne model") are both conformally flat, so conformal transformations certainly exist. But what on Earth does he think this proves? Maybe this?

In the Friedmann-Walker diagram, the light "from the big bang" crosses every single worldline. But in the "comoving particles" diagram, the light just passes a finite number of worldlines.

Oh wow, my gosh, he really is confused! I don't know what charts/frame fields he means by "Friedmann-Walker diagram" or "comoving particles diagram", but I don't have to, in order to know he has made some kind of serious error.

Unless, just possibly, he doesn't realize that the FRW dust with E^3 hyperslices (as in the coframe Lut Mentz wrote down) and the FRW dust with S^3 hyperslices are not locally isometric and thus not physically equivalent, and the second claim refers to an FRW dust with S^3 hyperslices, while the first refers to an FRW dust with E^3 hyperslices. If so, part of his confusion must involve some misinterpretation of a conformal mapping between two such distinct dust models, both conformally flat but with nonzero Ricci tensors and with different global (and local!) properties.

It's frustrating to see someone so confused, and possibly so confused by reading one bad book (at least, it was obviously bad for JDoolin to read that book), but from the posts by JDoolin I've seen so far, I think we can give him benefit of the doubt by assuming he is honestly curious and simply confused by his reading, not one of these idgits who have set out to blow down modern cosmology well before they have a clue what this subject is all about.

In "Special relativity adandons Maxwell's mechanical interpretation of EM?"

www.physicsforums.com/showthread.php?t=449268

there is a fine old Science article from c. 1979 which very clearly discusses Maxwell's mechanical model. Every five years I need to cite it and lately, find I can't remember author or title, which makes it difficult to find the citation. Sigh... Another good cite here would be Feynman's brilliant discussion of how EM waves propogate, in terms of the partial derivatives appearing in Maxwell's equations written out in conventional vector calculus style.

Re

www.physicsforums.com/showthread.php?t=449248

the perfect book for him would be Geroch, Mathematical Physics, written from the categorical point of view throughout. Also highly recommended: Lawvere and Schanuel, Conceptual Mathematics (much more sophisticated than you'd guess from the early chapters).

Re

www.physicsforums.com/showthread.php?t=448388

looks like the intersection of four conics on CP^4, so one can use Groebner basis methods to look for a solution, or at least for information about solutions. One can use Schubert calculus (cohomology of certain Grassmannians) to compute the expected number of solutions. If he wants real solutions rather than complex ones (as I suspect he does), things will probably be a lot more complicated, unless he gets lucky.

Re "Determining the Distribution of a Statistic"

[PLAIN]https://www.physicsforums.com/showthread.php?t=447116[/PLAIN]

Kendall, A Course in the Geometry of n Dimensions would be perfect for the OP. E.g., Kendall derives the distribution of chi-square from n-dimensional euclidean geometry. Most of the other distributions studied by people like Fisher can also be so derived, and Kendall does so. Great book for those interested in understanding the unity of mathematics!