Weinberg LN in QM (Section 3.5): Momentum operator

[jouvelot]

Hi everyone,

Weinberg uses spatial translation invariance to derive the momentum operator. But the way he does it puzzles me. Here is an excerpt of the book.

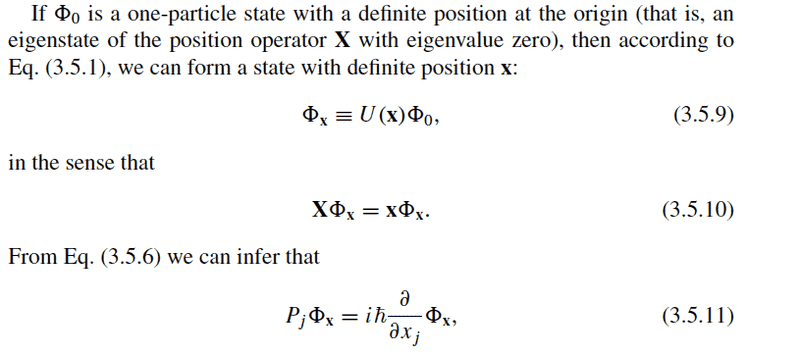
Equation 3.5.1 is the definition of the unitary operator ##U(x)## for translation invariance:
$$U^{-1}(x)XU(x) = X+x,$$ with ##-P/\hbar## as translation generator, while Equation 3.5.6 defines the commutator:
$$[X_i,P_j] = i\hbar\delta_{ij}.$$ What I don't get is how one can "infer" from this equation the momentum operator in Equation 3.5.11, in particular the usual partial derivative.

Note that there is Equation 3.5.8, which states that ##U(x) = exp(-iP.x/\hbar)##, and from which I could fathom Equation 3.5.11, however. Is (3.5.6) just a bogus reference (even though it appears in both the 2013 and 2015 editions of the book)?

Thanks in advance to anyone who can help.

Pierre

 

[dextercioby]

I don't question the physical insight of the Nobel Prize winner Steven Weinberg in writing textbooks (this QM one, by the way, is highly respected in the community), but I only sadly remark that the excerpt you quoted contains an array of mathematically ill-defined statements and equations whose debugging using also formal calculus I do not encourage, but hardly tolerate.

If Weinberg used proper mathematics, then 3.6 leading to 3.11 is the highly non-trivial result of Dixmier's theorem, a reformulation of Stone-von Neumann's theorem in terms of coordinates and momenta rather than Weyl's unitaries.

 

[vanhees71]

Well, Weinberg does physics not formal mathematics. I think this book is one of the best on QM written in the recent years. I think all of Weinberg's textbooks are masterpieces in clarity and style. Of course, he's a theoretical physicist and not a mathematician dealing with the exact formulation of QM as a mathematical theory.

I've Weinberg's book not with me over the weekend. So I provide my own derivation. The idea is the definition of the observables from group theory, based on the symmetry of the model. In this case we deal with the fundamental space-time symmetries and here even only with translation invariance. To keep the notation simple, I consider one-dimensional motion. The generalization to 3D is straight forward. In non-relatistic QM you can start with a minimal model, only invoking the translation group, assuming the existence of a position operator for a particle (strictly speaking the logic is to construct all ray representations of the Galileo group and then construct the appropriate position operators from it, but that's not necessary here). I also set ##\hbar=1## (natural units).

So we start with the Heisenberg algebra, defining momentum as the generator of spatial translations:
$$[\hat{x},\hat{p}]=\mathrm{i} \hat{1}.$$
Now we assume the existence of a (generalized) position eigenstate with eigenvalue ##0##, ##|x=0 \rangle## (I also use the Dirac notation; I don't know, why Weinberg doesn't like Dirac and find the Dirac notation much clearer, because you have a clear indication which kind of quantity you deal with). Now, if ##\hat{p}## generates spatial translations we should have
$$|x \rangle=\exp(-\mathrm{i} \hat{p} x)|x=0 \rangle.$$
To prove this we use the commutation relation in exponentiated form, i.e., we consider the operator-valued function
$$\hat{X}(\alpha)=\exp(\mathrm{i} \hat{p} \alpha) \hat{x} \exp(-\mathrm{i} \hat{p} \alpha).$$
We can easily derive a differential equation for this function by taking the derivative
$$\frac{\mathrm{d}}{\mathrm{d} \alpha} \hat{X}(\alpha)=\exp(\mathrm{i} \hat{p} \alpha) \mathrm{i} [\hat{p},\hat{x}]\exp(-\mathrm{i} \hat{p} \alpha)=\hat{1}.$$
Since ##\hat{X}(\alpha=0)=\hat{x}## we have
$$\hat{X}(\alpha)=\hat{x}+\alpha \hat{1}$$
and thus
$$\hat{x} \exp(-\mathrm{i} \hat{p} x)|x=0 \rangle = \exp(-\mathrm{i} \hat{p} x) \hat{X}(x)|x=0 \rangle = x \exp(-\mathrm{i} \hat{p} x)|x=0 \rangle,$$
i.e.
$$|x \rangle=\exp(-\mathrm{i} \hat{p} x)|x =0 \rangle$$
is a generalized eigenvector of ##\hat{x}## with eigenvalue ##x##. The spectrum of ##\hat{x}## is thus the entire real axis.

Now we can easily calculate the position representation of the momentum eigenstates, for which one can derive in the very same way as for ##\hat{x}## the spectrum to be also the entire real axis using that ##-\hat{x}## is the generator for momentum translations again using the Heisenberg commutation relation:
$$u_p(x)=\langle x|p \rangle=\langle x=0|\exp(+\mathrm{i} \hat{p} x|p \rangle = \exp(\mathrm{i} \hat{p} x) \langle x=0|p \rangle.$$
Now we want to normalize this to a ##\delta## distribution,
$$\langle p|p' \rangle=\delta(p-p') \; \Rightarrow \; \langle x=0|p \rangle=\frac{1}{\sqrt{2 \pi}},$$
so that
$$u_p(x)=\langle x|p \rangle=\frac{1}{\sqrt{2 \pi}} \exp(\mathrm{i} p x).$$
Now for a Hilbert-space vector we have for the wave function in position representation ##\psi(x)=\langle x|\psi \rangle##
$$\hat{p} \psi(x):=\langle x|\hat{p} \psi \rangle = \int_{\mathbb{R}} \mathrm{d} p p u_p(x) \langle p|\psi \rangle=-\mathrm{i} \partial_x \int_{\mathbb{R}} \mathrm{d} p u_p(x) \langle p|\psi \rangle=-\mathrm{i} \partial_x \psi(x).$$
All this is of course only a physicist's formal derivation. To prove all this in a mathematical rigorous way needs an entire book on Hilbert space theory/functional analysis.

 

[jouvelot]

Dear dextercioby,

Thanks a lot for your interesting comment: I'll look to this Dixmier's theorem online for hints then.

On a grander scheme of things, which "cleaner" QM book would you advise? QM-wise, I only read before the QM book by Landau and Lifshitz, before moving on to QED and QFT.

 

[jouvelot]

Dear vanhees71,

Thanks a lot for the detailed derivation, the first half of which is obtained in Weinberg's book as the limit of iterated infinitesimal translation operators; but your's is neat too.

The second part or your message provides another path to the derivation of the operator momentum in the space domain than the one he suggests, I think, although your's is quite clear (thanks). In fact, the next few paragraphs in the text derive the properties such as normalization to a delta distribution for states with definite momentum that you, in your message, assumed to obtain the momentum operator representation. He is going in the reverse direction, as far as I understand.

Thanks a lot for your help :)

 

[dextercioby]

Dear dextercioby,

Thanks a lot for your interesting comment: I'll look to this Dixmier's theorem online for hints then.

On a grander scheme of things, which "cleaner" QM book would you advise? QM-wise, I only read before the QM book by Landau and Lifshitz, before moving on to QED and QFT.

A. Galindo and P. Pascual's two volume text on quantum mechanics is the equivalent (from the respect for mathematics point of view) of Wald's book on General Relativity. For a middle level (no functional analysis used) text, you can stick with Weinberg without a problem, though.

 

[jouvelot]

Dear dextercioby,

Thanks for the references to the book and also to Dixmier and Stone-von Neumann theorems, which, from what I saw on the Internet, are far from trival; the "inference" mentioned by Weinger is not that obvious apparently ;)

 

[jouvelot]

Now for a Hilbert-space vector we have for the wave function in position representation ##\psi(x)=\langle x|\psi \rangle##
$$\hat{p} \psi(x):=\langle x|\hat{p} \psi \rangle = \int_{\mathbb{R}} \mathrm{d} p p u_p(x) \langle p|\psi \rangle=-\mathrm{i} \partial_x \int_{\mathbb{R}} \mathrm{d} p u_p(x) \langle p|\psi \rangle=-\mathrm{i} \partial_x \psi(x).$$
All this is of course only a physicist's formal derivation. To prove all this in a mathematical rigorous way needs an entire book on Hilbert space theory/functional analysis.

BTW, your derivation confirms that there is also a sign typo in Equation (3.5.11), contradicting a footnote in Weinberg's text in Section 3.1 defining the operator momentum as ##-i\hbar\nabla##.

 

[vanhees71]

Maybe it's not a typo. I've a bit of a problem with Weinberg's unusual notation. I always have to check the meaning of his symbols. The refusal to use the ingenious notation by Dirac is the only thing I don't like with his otherwise brilliant book on QM. I guess Weinbergs ##\Phi_x## stands for ##|x \rangle## (again going back to the 1D case for laziness, of course everything is straightforward to extend to 3D position and momentum vectors/operators), because then starting with
$$|x \rangle =\exp(-\mathrm{i} \hat{p} x)|x=0 \rangle$$
you get
$$\partial_x |x \rangle=-\mathrm{i} \hat{p} \exp(-\mathrm{i} \hat{p} x)|x=0 \rangle = -\mathrm{i} \hat{p} |x \rangle$$
or
$$\hat{p} |x \rangle = \mathrm{i} \partial_x |x \rangle.$$
Now for the wave function ##\psi(x)=\langle x|\psi \rangle## you need the Hermitean conjugate of the previous Eq., i.e., using ##\hat{p}^{\dagger}=\hat{p}##,
$$\langle x |\hat{p} =-\mathrm{i} \partial_x \langle x|.$$
Now multiplying ##|\psi \rangle## from the right again leads to
$$\hat{p} \psi(x)=\langle x|\hat{p} \psi \rangle=-\mathrm{i} \partial_x \langle x|\psi \rangle = - \mathrm{i} \partial_x \psi(x).$$
Note that I use ##\hat{p}## here in different meanings. On the left-hand side of the equation it's an operator in the function-Hilbert space of square integrable functions, ##L^2##, (position-space representation), while when applied in the next step to ##|\psi \rangle## it's an abstract operator in the abstract Hilbert space ##\mathcal{H}##, which can be seen as the equivalence class of all possible realization of the separable Hilbert space, which is unique in the sense that each separable Hilbert space is by definition equivalent to ##\ell^2##, the Hilbert space of square summable sequences.

 

[jouvelot]

I had gotten the first part, but didn't make the subtle distinction you explicit in the second part and was thus confused by the apparently contradictory footnote and unusual sign (not mentioning the "inference" step I was alluding to at the start of the thread, which made me doubt everything). Indeed, using the same notation in two different spaces doesn't help at first; the devil is in the details ;)

Thanks a great lot for the clarification :)

 

[vanhees71]

Indeed, perhaps one should distinguish the operators in a given representation (here the position representation) from the operator in the same formalism somehow, perhaps like
$$\tilde{p} \psi(x)=\langle x|\hat{p} \psi \rangle.$$

 

[jouvelot]

Well, as a computer scientist, I'm used to overloading operators... but it clearly shows that it's a dangerous tool to use, in computer science as in physics

Thanks a lot.