Understanding Line Integrals: Scalar vs Vector Fields

[cshum00]

Well i know that the line integral is

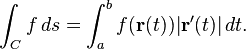given a scalar function f. equation1

But the line integral is also

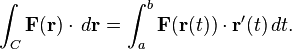given a vector field F. equation2

So, given scalar function f and taking the gradient vector of it in order to turn it into a vector field F. Why is equation1 not equal to equation2?

 

[HallsofIvy]

It's not clear to me what you are asking. If [itex]F(r)= \nabla f[/itex] then it is certainly true that [itex]\int_C \nabla F\cdot dr= \int_a^b \nabla F(r)\cdot r'(t)dt[/itex]. But I don't know what you mean by "equation1 equal to equation2".

 

[cshum00]

Given [itex] \nabla f = F(r)[/itex]
[itex]\int_a^b f(r(t)) |r'(t)|dt \neq \int_a^b F(r(t)) \cdot r'(t)dt[/itex]
Why?

 

[HallsofIvy]

Given [itex] \nabla f = F(r)[/itex]
[itex]\int_a^b f(r(t)) |r'(t)|dt \neq \int_a^b F(r(t)) \cdot r'(t)dt[/itex]
Why?

Why should [itex]\int_a^b f(r(t)) |r'(t)|dt \neq \int_a^b \nabla f(r(t)) \cdot r'(t)dt[/itex]?

 

[cshum00]

Well, let's try some example:

Let [itex]f(x,y) = xy[/itex]
[itex]F=\nabla f(x,y)=<y,x>[/itex]

Curve C => [itex]y=x, 0<x<1[/itex]
Parametrize [itex]r(t)=<t,t>, 0<t<1[/itex]
[itex]r'(t)=<1,1>[/itex]
[itex]|r'(t)|=\sqrt2[/itex]

[itex]f(r(t))=t^2[/itex]
[itex]F(r(t))=<t,t>[/itex]

If [itex]\int_a^b f(r(t))|r'(t)|dt = \int_a^b F(r(t)) \cdot r'(t)dt[/itex]
[itex]\int_0^1 t^2 \sqrt2dt = \int_0^1 <t,t> \cdot <1,1>dt[/itex]
[itex](\sqrt2/3) t^3 |_0^1 = \int_0^1 2t dt[/itex]
[itex]\sqrt2/3 = t^2 |_0^1[/itex]
[itex]\sqrt2/3 \neq 1[/itex]

 

[cshum00]

The only reason i have been asking this:
[itex]\int_a^b f(r(t)) |r'(t)|dt \neq \int_a^b \nabla f(r(t)) \cdot r'(t)dt[/itex]
is because the formula given on the book say that they should be equal. However, as i try many examples; i never get the same answer. Here is some general proof i have been trying but never got far.

Let [itex]f(x,y)=f(x,y)[/itex]
[itex]F = \nabla f(x,y)=<f_x, f_y>[/itex]

Let Parametrization of Curve C:
[itex]r(t) = <g(t), h(t)>[/itex]
[itex]r'(t) = <g'(t), h'(t)>[/itex]
[itex]|r'(t)| = [g'(t)^2 + h'^2(t)]^\frac{1}{2}[/itex]

[itex]\int_a^b f(r(t)) |r'(t)|dt = \int_a^b \nabla f(r(t)) \cdot r'(t)dt[/itex]
[itex]\int_a^b f(g(t), h(t))[g'(t)^2 + h'(t)^2]^\frac{1}{2}dt = \int_a^b <f(_x, f_y> \cdot <g'(t), h'(t)>dt[/itex]
[itex]\int_a^b f(g(t), h(t))[g'(t)^2 + h'(t)^2]^\frac{1}{2}dt = \int_a^b g'(t)f_x(g(t), h(t))+ h'(t)f_y(g(t), h(t))dt[/itex]Since limits and variable of integration are the same then
[itex]f(g(t), h(t))[g'(t)^2 + h'(t)^2]^\frac{1}{2} = g'(t)f_x(g(t), h(t)) + h'(t)f_y(g(t), h(t))[/itex]
But since i don't have any idea of what is going on inside those partial derivatives, i don't have a clue how to proceed.

Edit: In addition, the right hand side kind of looks like the product rule of a derivative.