Laplace equation on a trapezoid

[MatPhy]

Hello everybody!

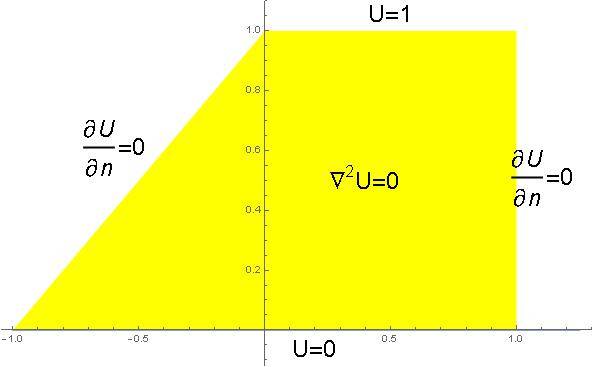
I know how to solve Laplace equation on a square or a rectangle.

Is there any easy way to find an analytical solution of Laplace equation on a trapezoid (see picture).

Thank you.

 

[pasmith]

Solve it in the triangle using polar coordinates with origin at (-1,0), and in the square in cartesian coordinates. Patch the two together by requiring continuity on (0,0) to (0,1).

 

[MatPhy]

Pasmith, thank you for the answer. But maybe it is possible to solve this in this way:

 

[pasmith]

I don't think that allows you to determine [itex]f[/itex] uniquely.

I did find the solution [tex]
u(x,y) = \begin{cases} y, & 0 \leq x \leq 1, 0 \leq y \leq 1, \\ (x + 1)y, & -1 \leq x < 0, 0 \leq y \leq 1 + x \end{cases}[/tex] by considering solutions of the form [itex]u(x,y) = yf(x)[/itex], motivated by the condition on [itex]y = 0[/itex]. That gave me [itex]u(x,y) = Axy+ By[/itex]. Allowing for different values of [itex]A[/itex] and [itex]B[/itex] on either side of [itex]x = 0[/itex] gives four unknowns, and using these it proved possible to satisfy the remaining boundary conditions and the condition of continuity at [itex]x = 0[/itex].

 

[MatPhy]

Pasmith, thank you for the answer.

But in my opinion we should also consider this relation:

[tex]{\partial u_1(x,y) \over \partial x} = {\partial u_2(y) \over \partial x}[/tex] at [tex]x=0 \quad \text{,}[/tex]

where
[tex]u_1(x,y) = (x+1)y[/tex] on subdomain [tex]-1 \leq x < 0, 0 \leq y \leq 1 + x \quad \text{and}[/tex]
[tex]u_2(y) = y[/tex] on subdomain [tex]0 \leq x \leq 1, 0 \leq y \leq 1 \quad \text{.}[/tex]

But [tex]{\partial u_2(y) \over \partial x} = 0[/tex]

and

[tex]{\partial u_1(x,y) \over \partial x} = y \neq 0 \quad \text{.}[/tex]

 

[pasmith]

You can't require continuity of both [itex]u[/itex] and [itex]\partial u/\partial x[/itex] at [itex]x = 0[/itex]; all you can do is require continuity of a linear combination of [itex]u[/itex] and [itex]\partial u/\partial x[/itex].

 

[MatPhy]

Well numerical solution gave different result. Solution for U(x,y) at [tex]0 \leq y \leq 1 \quad \text{and} \quad x=0[/tex] is

 

[Anna2016_2]

Does an analytical solution exists for the same problem but when switching between Neumann and Dirichlet boundary conditions? That is if we set no flux (Neumann) boundary conditions along the bases of the trapezoid, and the same Dirichlet boundary conditions as prescribed above, along the two other sides of the trapezoid?

Thank you!