Lagrangian function of a double undamped pendulum

[PaBlo14101066]

I must find the Lagrangian for an undamped pendulum using the diagram showed below, I've no idea what to do with the second angle φ2 because is measured from the line that joins the two pivot points.

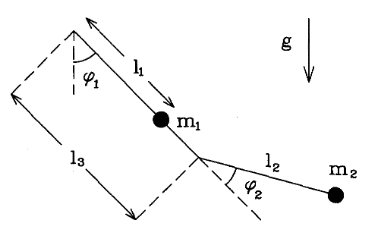
The ecuations I must obtain are as follows

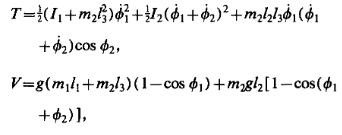
I get so many different things but I can't reach the desired result

 

[BvU]

I've no idea what to do

If all else fails, you could try $$T = {1\over 2} m_1\Bigl(\dot x_1^2+\dot y_1^2\Bigr) + {1\over 2} m_2\Bigl(\dot x_2^2+\dot y_2^2\Bigr) $$ but of course you already know how the subscript 1 term ends up, so the work is in the ##m_2## term.

Idem ##V##.

 

[PaBlo14101066]

If all else fails, you could try $$T = {1\over 2} m_1\Bigl(\dot x_1^2+\dot y_1^2\Bigr) + {1\over 2} m_2\Bigl(\dot x_2^2+\dot y_2^2\Bigr) $$ but of course you already know how the subscript 1 term ends up, so the work is in the ##m_2## term.

Idem ##V##.

Yes, I know, and also there must be a rotational kinetic energy, I made the algebra considering that φ2 is measured from the vertical and obtained

But even though I consider $$\phi_2=\phi_1+\phi'$$ I don't get to the equations of the second image

 

[etotheipi]

Don't you get, in terms of ##\hat{x}## and ##\hat{y}##,$$\dot{\mathbf{r}}_1 = l_1 \dot{\varphi}_1 \begin{pmatrix} \cos{\varphi_1} \\ \sin{\varphi_1} \end{pmatrix}$$and$$\dot{\mathbf{r}}_2 = l_3 \dot{\varphi}_1 \begin{pmatrix} \cos{\varphi_1} \\ \sin{\varphi_1} \end{pmatrix}

+ l_2 (\dot{\varphi}_1 + \dot{\varphi}_2) \begin{pmatrix} \cos{(\varphi_1 + \varphi_2)} \\ \sin{(\varphi_1 + \varphi_2)} \end{pmatrix}

$$Now you can find ##\dot{\mathbf{r}}_1 \cdot \dot{\mathbf{r}}_1## and ##\dot{\mathbf{r}}_2 \cdot \dot{\mathbf{r}}_2##. Does that work?

 

[PaBlo14101066]

Don't you get, in terms of ##\hat{x}## and ##\hat{y}##,$$\dot{\mathbf{r}}_1 = l_1 \dot{\varphi}_1 \begin{pmatrix} \cos{\varphi_1} \\ \sin{\varphi_1} \end{pmatrix}$$and$$\dot{\mathbf{r}}_2 = l_3 \dot{\varphi}_1 \begin{pmatrix} \cos{\varphi_1} \\ \sin{\varphi_1} \end{pmatrix}

+ l_2 (\dot{\varphi}_1 + \dot{\varphi}_2) \begin{pmatrix} \cos{(\varphi_1 + \varphi_2)} \\ \sin{(\varphi_1 + \varphi_2)} \end{pmatrix}

$$Now you can find ##\dot{\mathbf{r}}_1 \cdot \dot{\mathbf{r}}_1## and ##\dot{\mathbf{r}}_2 \cdot \dot{\mathbf{r}}_2##. Does that work?

I think that's it, I would have to do the algebra, but as far as it looks, looks fine

 

[etotheipi]

I think that's it, I would have to do the algebra, but as far as it looks, looks fine

Yes, I believe it does indeed get what you're after. The only fiddly part of the calculation is$$
\begin{align*}
\begin{pmatrix} \cos{\varphi_1} \\ \sin{\varphi_1} \end{pmatrix} \cdot \begin{pmatrix} \cos{(\varphi_1 + \varphi_2)} \\ \sin{(\varphi_1 + \varphi_2)} \end{pmatrix} &=

\cos{\varphi_1} \left( \cos{\varphi_1} \cos{\varphi_2} - \sin{\varphi_1} \sin{\varphi_2}\right) + \sin{\varphi_1} \left( \sin{\varphi_1} \cos{\varphi_2} + \cos{\varphi_1} \sin{\varphi_2}\right)

\\
&= \cos{\varphi_2} \left( \cos^2{\varphi_1} + \sin^2{\varphi_1} \right) \\

&= \cos{\varphi_2}

\end{align*}
$$but the rest should fall out okay.

 

[PaBlo14101066]

Yes, I believe it does indeed get what you're after. The only fiddly part of the calculation is$$
\begin{align*}
\begin{pmatrix} \cos{\varphi_1} \\ \sin{\varphi_1} \end{pmatrix} \cdot \begin{pmatrix} \cos{(\varphi_1 + \varphi_2)} \\ \sin{(\varphi_1 + \varphi_2)} \end{pmatrix} &=

\cos{\varphi_1} \left( \cos{\varphi_1} \cos{\varphi_2} - \sin{\varphi_1} \sin{\varphi_2}\right) + \sin{\varphi_1} \left( \sin{\varphi_1} \cos{\varphi_2} + \cos{\varphi_1} \sin{\varphi_2}\right)

\\
&= \cos{\varphi_2} \left( \cos^2{\varphi_1} + \sin^2{\varphi_1} \right) \\

&= \cos{\varphi_2}

\end{align*}
$$but the rest should fall out okay.

Yes, that's it. Thank you very much. You've earned heaven (whatever heaven is to you) :)