- #1
MarkFL
Gold Member
MHB
- 13,288
- 12
Here are the questions:
I have posted a link there to this topic so the OP can see my work.
Calculus word problems?
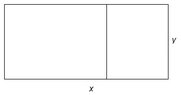
1. A rancher has 200 ft of fencing with which to enclose two adjacent rectangular corrals, as shown. What dimensions should be used so that the enclosed area will be a maximum?
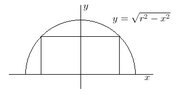
2.A rectangle is inscribed inside a semi-circle with intercepts at (-8,0), (0,8), and (8,0). Find a function that models the area of the rectangle in terms of half of the base, x, of the rectangle, as shown in the figure below. Determine what x-value will produce an area of 30 square units.
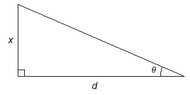
3. A camera is mounted at a point 300 ft from the base of a rocket launching pad. The rocket rises vertically when launched. Express the distance, x, traveled by the rocket as a function of the camera elevation angle, theta. Find the distance traveled by the rocket when the angle of elevation is 20 degrees.
PLEASE EXPLAIN
I have posted a link there to this topic so the OP can see my work.