Just a question about the tangent basis

[etotheipi]

I was reading these notes and then on page 23 I saw something a bit weird. Back in this thread I learned that ##\{ \partial_i \}## form a basis of ##T_p M##, and that a tangent vector can be written ##X = X^i \partial_i##, and it's not too difficult to show that components transform like ##\bar{X}^j = \frac{\partial \bar{x}^j}{\partial x^i} X^i##. But this guy says that

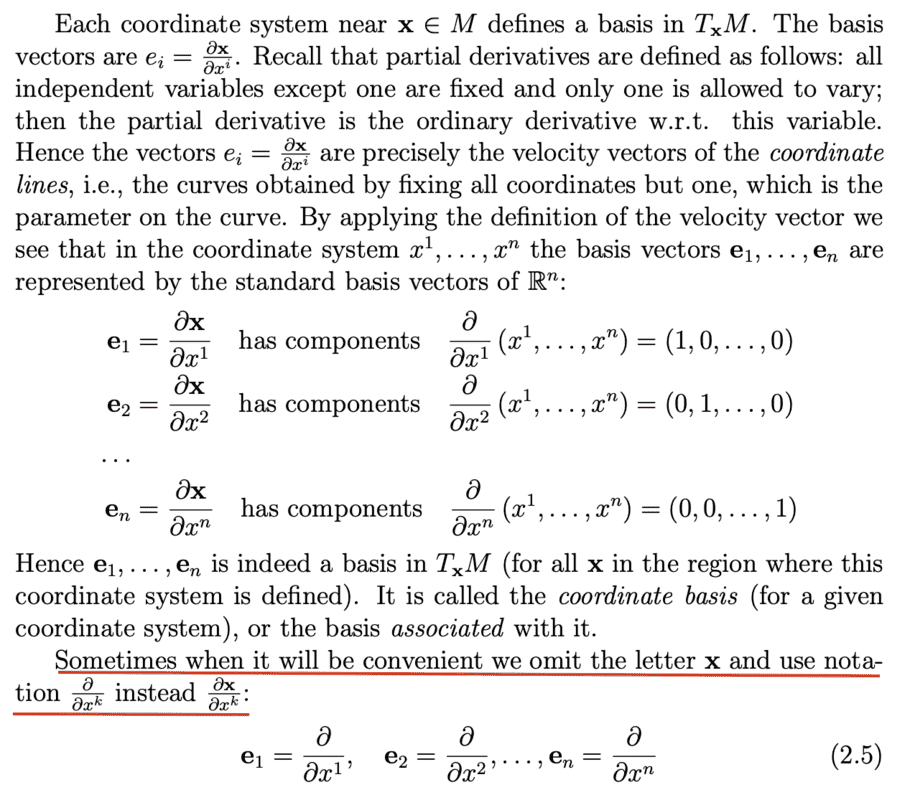
The most alarming part of that is that he seems to suggest that ##\frac{\partial}{\partial x^i}## is just shorthand/notation for ##\frac{\partial \vec{x}}{\partial x^i}##. That seems quite wrong, since I thought the operators ##\frac{\partial}{\partial x^i}## are basis vectors already, in their own right. And ##\partial_i## and ##\partial_i \vec{x}## are completely different objects. Plus, the base space is not even necessarily a vector space, in which case I thought the position vector ##\vec{x}## is not even defined!

So I wondered if this section is wrong, or if I am missing something . Thanks!

 

[PeroK]

I think that ##\vec x = (x^1, x^2, \dots , x^n)## is slightly loose notation, as this is more the coordinates than a vector. But, I'd say ##\frac{\partial}{\partial x^1}## is definitely shorthand for fixing all other coordinates and letting only ##x^1## vary.

The transition to treating ##\frac{\partial}{\partial x^1}## as a vector, however you define it, is by identifying a basis vector with this and then the key ingredient is to define an inner product by making the inner product of your basis vectors dependent on the metric.

Effectively what you have done - when all is said and done - is simply defined a tangent space with an appropriate inner product. How precisely you relate that back to the manifold is not actually that important.

PS I mean the precise justification is not important. You obviously have to get precisely the right inner product on your tangent space.

 

[PeterDonis]

I thought the operators ##\frac{\partial}{\partial x^i}## are basis vectors already, in their own right.

They are if you use the one-to-one correspondence between vectors and directional derivative operators, and treat ##\frac{\partial}{\partial x^i}## as the directional derivative operator along the "coordinate grid" curve on which only ##x^i## changes and all other coordinates are constant. I think that's what this author is referring to when he says that ##\frac{\partial}{\partial x^i}## are "the velocity vectors of the coordinate lines". I agree he is fudging somewhat when he appears to treat the n-tuple of coordinates as a vector.

 

[etotheipi]

Ah, okay, I think I get it. He's writing coordinate basis of ##T_p \mathbb{R}^n##. I think I was getting confused because in a Euclidean space, where a position vector makes sense, sometimes it's written$$\vec{E}_a = \frac{\partial \vec{x}}{\partial y^a} = \frac{\partial x^i}{\partial y^a} \vec{e}_i$$where the ##\vec{e}_i## are a Cartesian set. But that's a different usage of the symbol ##\vec{x}## to what he's doing here. Thanks!

 

[etotheipi]

Funnily enough, I found another version of these same notes, and they've gotten rid of the confusing shorthand:

https://personalpages.manchester.ac.uk/staff/theodore.voronov/Teaching/Differentiable%20Manifolds/2018-2019/4-tangent-vectors.pdf

This is a lot better, because ##\frac{\partial \mathbf{x}}{\partial x^i}## is a tuple in ##\mathbb{R}^n##. The weird shorthand ##\frac{\partial}{\partial x^i}## would have you believe that it's actually a derivative, or an operator, which is a bit silly.

Anyway, I think I'm done for today. Thanks for helping!

 

[vanhees71]

Ah, okay, I think I get it. He's writing coordinate basis of ##T_p \mathbb{R}^n##. I think I was getting confused because in a Euclidean space, where a position vector makes sense, sometimes it's written$$\vec{E}_a = \frac{\partial \vec{x}}{\partial y^a} = \frac{\partial x^i}{\partial y^a} \vec{e}_i$$where the ##\vec{e}_i## are a Cartesian set. But that's a different usage of the symbol ##\vec{x}## to what he's doing here. Thanks!

I wouldn't use ##\vec{x}## as a notation for ##x^{\mu}##, because in GR these are not components of a vector but just the coordinates (of a local chart).

 

[PeroK]

Ah, okay, I think I get it. He's writing coordinate basis of ##T_p \mathbb{R}^n##. I think I was getting confused because in a Euclidean space, where a position vector makes sense, sometimes it's written$$\vec{E}_a = \frac{\partial \vec{x}}{\partial y^a} = \frac{\partial x^i}{\partial y^a} \vec{e}_i$$where the ##\vec{e}_i## are a Cartesian set. But that's a different usage of the symbol ##\vec{x}## to what he's doing here. Thanks!

I'm working through Gravity and Geometry by Sean Carroll. Here is my summary of the tangent space as he presents it.

Consider the set of all parametrised curves thru a point ##p## in a manifold, ##M##. Note that:

• Each curve itself is a geometric object, which will be used to define/identify a direction at ##p##; and,
• Each specific parameterisation of the curve is associated with a magnitude of rate of change of the curve at ##p##
• Together these will be associated with a local vector at ##p##.

Note: as ##M## is a manifold, we have ##p \in U##, for some open set ##U## and at least one coordinate chart (a 1-1 mapping of ##U## into ##\mathbb{R}^n##). We may use this mapping to associate functions and derivatives of real-valued functions on ##U## with functions on ##\mathbb{R}^n##. It would get cluttered to include all these mappings, so we assume that everything works via mappings backwards and forwards from ##M## to ##\mathbb{R}^n## where required.

Suppose we have a curve, ##C##, parametrised by ##\lambda## and a smooth real-valued function ##f## on ##M##. We can define a (total) derivative of ##f## with respect to this curve by:
$$\frac{df}{d\lambda} = \frac{\partial f}{\partial x^{\mu}} \frac{dx^{\mu}}{d \lambda}$$
Note that this is coordinate independent (easy to check) and hence well defined.

Now, we consider the set ##F## of all smooth real-valued functions on ##U##. And we see that ##C[\lambda]## is a linear operator/functional on ##F##:
$$C[\lambda](f) = \frac{df}{d\lambda}$$
Moreover, the set of all parameterised curves is a vector space of linear operators on ##F##. This is fairly easy to check.

The tangent space ##T_p## is then associated with this space of linear operators ##C[\lambda]##.

Next, given a coordinate chart, we want to establish the coordinate basis for ##T_p##. First, note that the partial derivatives ##\partial_{\mu} = \frac{\partial }{\partial x^{\mu}}## are themselves in ##T_p##. It's easy to find a simple parametrisation of a curve for which ##C[\lambda](f) = \partial_{\mu}f##. Not surprisingly, these operators will be our coordinate basis. Then any vector ##V \in T_p## has the representation in the coordinate basis:
$$C[\lambda] = V = V^{\mu}e_{\mu} = \frac{dx^{\mu}}{d \lambda}\partial_{\mu}$$
In summary:

• Parameterised curves in ##M## are associated with linear operators (on the space of smooth real-valued functions on ##M##) are associated with vectors in the tangent space
• Given any coordinate chart, the coordinate partial derivatives form a basis (of linear operators); and,
• The components are the derivatives of the coordinates with respect to the specific parameter

If we tried to dot the mathematical i's and cross the t's, we'd get bogged down in mappings going back and forth. In any case, Carroll presents a solid mathematical foundation for the tangent space.

Note: the ##C[\lambda]## is my notation.