- #1
anemone
Gold Member
MHB
POTW Director
- 3,883
- 115
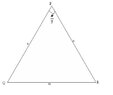
Given a triangle $PQR$ where $QR=m$, $PQ=PR=n$ and $\angle P=\dfrac{\pi}{7}$.
View attachment 2522
Show that $m^4-3m^2n^2-mn^3+n^4=0$.
View attachment 2522
Show that $m^4-3m^2n^2-mn^3+n^4=0$.