- #1
Happiness
- 679
- 30
Why are the solutions satisfying ##\psi(x+l)=\lambda\,\psi(x)## (4.191) the only physically admissible solutions? (##l## is the period of the periodic potential.)
We may argue that the probability of finding an electron at ##x##, ##|\psi(x)|^2##, must be the same at any indistinguishable position:
##|\psi(x+l)|^2=|\psi(x)|^2##
This implies
##\psi(x+l)=\lambda\,\psi(x)##
##|\lambda|^2=1##
##\lambda=e^{iKl}##, which is the same as (4.196)
It seems that we can get (4.196) in a shorter way this way as compared to how it is done below by using the characteristic equation of matrix ##a## and the Wronskian determinant.
So it seems that the book justifies (4.191) in a different way from the argument using probability.
EDIT: I realize the probability argument does not justify the use of (4.191) either. Rather, it is justified by symmetry: Since the potential is periodic, the expectation values of all dynamical variables must be identical in every period. The only way this can happen is when (4.191) and (4.196) are true.
Still, it's unclear why the book uses such a long way to derive (4.196), without justifying the use of (4.191).
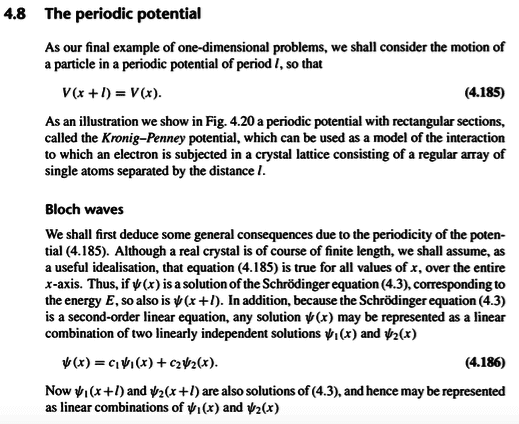
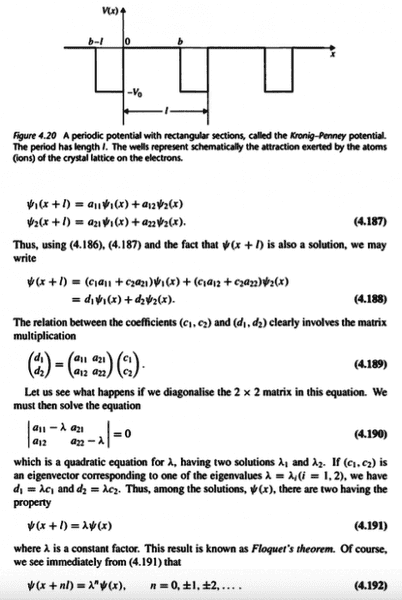

We may argue that the probability of finding an electron at ##x##, ##|\psi(x)|^2##, must be the same at any indistinguishable position:
##|\psi(x+l)|^2=|\psi(x)|^2##
This implies
##\psi(x+l)=\lambda\,\psi(x)##
##|\lambda|^2=1##
##\lambda=e^{iKl}##, which is the same as (4.196)
It seems that we can get (4.196) in a shorter way this way as compared to how it is done below by using the characteristic equation of matrix ##a## and the Wronskian determinant.
So it seems that the book justifies (4.191) in a different way from the argument using probability.
EDIT: I realize the probability argument does not justify the use of (4.191) either. Rather, it is justified by symmetry: Since the potential is periodic, the expectation values of all dynamical variables must be identical in every period. The only way this can happen is when (4.191) and (4.196) are true.
Still, it's unclear why the book uses such a long way to derive (4.196), without justifying the use of (4.191).
Last edited: