- #1
Happiness
- 679
- 30
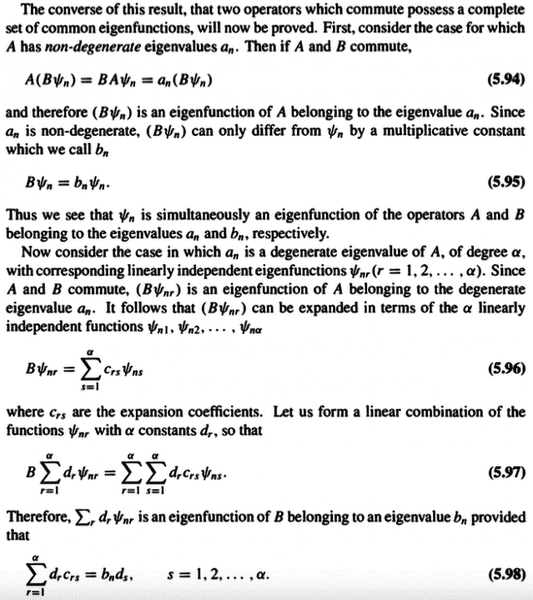
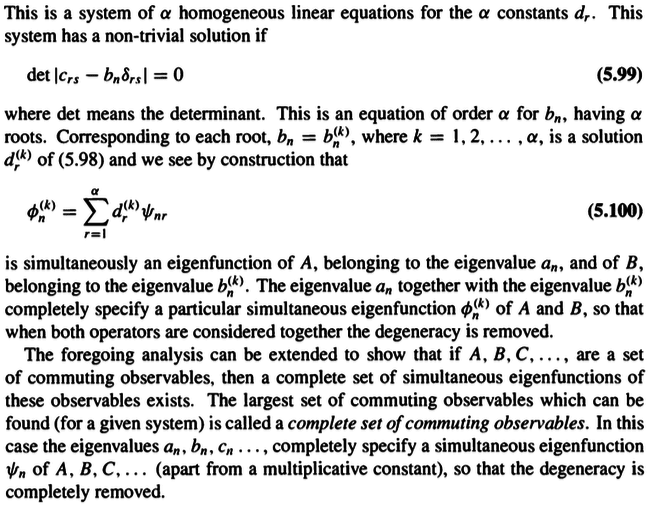
The following is a proof that two commuting operators ##A## and ##B## possesses a complete set of common eigenfunctions. The issue I have with the proof is the claim that the eigenvalue ##a_n## together with the eigenvalue ##b_n## completely specify a particular simultaneous eigenfunction ##\phi_n^{(k)}## of ##A## and ##B##, so that when both operators are considered together the degeneracy is completely removed.
(5.99) may not have ##\alpha## distinct roots. If so, ##a_n## together with ##b_n## do not completely specify a particular simultaneous eigenfunction, since an eigenvalue ##b_n^{(k)}## may have more than one solution ##d_r^{(k)}## corresponding to it.
Furthermore, the last paragraph mentions that the degeneracy is completely removed when the largest set of commuting observables are considered. This implies that if ##A## and ##B## do not form the largest set of commuting observables, the degeneracy may not be not completely removed. So I believe the claim is not proved.
(5.99) may not have ##\alpha## distinct roots. If so, ##a_n## together with ##b_n## do not completely specify a particular simultaneous eigenfunction, since an eigenvalue ##b_n^{(k)}## may have more than one solution ##d_r^{(k)}## corresponding to it.
Furthermore, the last paragraph mentions that the degeneracy is completely removed when the largest set of commuting observables are considered. This implies that if ##A## and ##B## do not form the largest set of commuting observables, the degeneracy may not be not completely removed. So I believe the claim is not proved.