Computations with Tangent Vectors and Pushforwards - Lee

[Math Amateur]

I am reading John M. Lee's book: Introduction to Smooth Manifolds ...

I am focused on Chapter 3: Tangent Vectors ...

I need some help in fully understanding Lee's conversation on computations with tangent vectors and pushforwards ... in particular I need clarification on the nature of the 'vectors' [itex]\partial / \partial x_i |_p [/itex] ... ...

The relevant conversation in Lee is as follows:

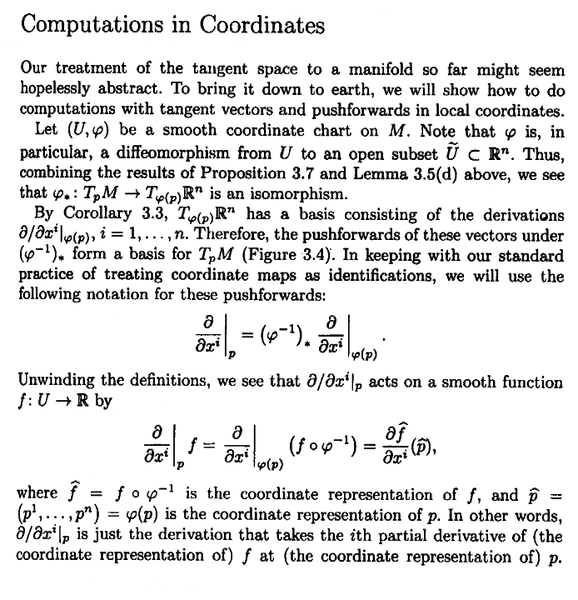
In the above text from Lee we read the following:

" .. ... The vectors [itex]\partial / \partial x_i |_p [/itex] are called the coordinate vectors at [itex]p[/itex] associated with a given coordinate system ... ... "

My question is as follows:

How or in what sense are the [itex]\partial / \partial x_i |_p [/itex] vectors ... they are certainly not objects with a magnitude and direction ... they seem to me to be maps or operators ... ...

Indeed they are defined by Lee as follows:

[itex]\frac{ \partial }{ \partial x^i } |_p = ( \phi^{-1}_* ) \frac{ \partial }{ \partial x^i } |_{\phi(p)} [/itex]Thus, the [itex]\frac{ \partial }{ \partial x^i } |_p[/itex] are mappings ... put in a smooth function [itex]f[/itex] and get out a real number ...

So ... how, or in what sense are these objects vectors ...

Hope someone can clarify this issue ...

Peter

 

[Brian T]

Hi Peter,
To understand how the objects ##\frac{\partial}{\partial x^i}## are vectors, consider the following:
Take a function ##f## defined in a neighbor of ##p \in M##, and a curve ##\alpha(t)## which passes through ##p## at ##\alpha(0)##. The time derivative of ##f## on the path is given by the chain rule, explicitly
$$ \frac{d(f \circ \alpha)}{dt} |_{t=0} = \frac{dx^i}{dt}\frac{\partial f}{\partial x^i} |_{p} $$
From this, we see that different velocities will give different time derivatives of f (the components ##\frac{dx^i}{dt}## will be different). Consequently, we can say that the vectors are equivalent to these directional derivatives on functions, i.e. these derivatives form an equivalence class for the vectors themselves. Also, these derivatives satisfy the properties of a vector space. If you go back to a manifold in an ambient space, say 2d surfaces in ##\mathbb{R}^3##, this definition fits since for a surface parametrized by ##\vec{x}(u,v)##, the tangent vectors ##\vec{x}_u## and ##\vec{x}_v## satisfy ##\vec{x}_u[f] = \partial_uf## and ##\vec{x}_v[f] = \partial_vf##. This is a common theme in geometry to define objects in a way that agrees with our intuition from Euclidian space but also allows us to generalize to non-Euclidian spaces.
For an arbitrary vector acting on a function, we have
$$X[f] = X^i \frac{\partial f}{\partial x^i} $$
This gives the expression for X written in the coordinate basis ##\frac{\partial}{\partial x^i}##:
$$X = X^i \frac{\partial}{\partial x^i}$$
We can then define the tangent plane at this point ##T_p M## as the set of all velocity vectors at that point for all possible curves passing through ##p##. When you're dealing with vectors on a manifold, one cannot specify a "direction" in the Euclidian sense by referencing some ambient space; rather, we say that the vector pointing in a direction (say the ##u## direction) takes the ##u## derivative of a function. Also, these vectors do have a magnitude if we are working with a Riemannian manifold, where the components of the metric are given by
##g_{i j} = < \frac{\partial}{\partial x^i} , \frac{\partial}{\partial x^j}> ##

 

[Math Amateur]

Hi Peter,
To understand how the objects ##\frac{\partial}{\partial x^i}## are vectors, consider the following:
Take a function ##f## defined in a neighbor of ##p \in M##, and a curve ##\alpha(t)## which passes through ##p## at ##\alpha(0)##. The time derivative of ##f## on the path is given by the chain rule, explicitly
$$ \frac{d(f \circ \alpha)}{dt} |_{t=0} = \frac{dx^i}{dt}\frac{\partial f}{\partial x^i} |_{p} $$
From this, we see that different velocities will give different time derivatives of f (the components ##\frac{dx^i}{dt}## will be different). Consequently, we can say that the vectors are equivalent to these directional derivatives on functions, i.e. these derivatives form an equivalence class for the vectors themselves. Also, these derivatives satisfy the properties of a vector space. If you go back to a manifold in an ambient space, say 2d surfaces in ##\mathbb{R}^3##, this definition fits since for a surface parametrized by ##\vec{x}(u,v)##, the tangent vectors ##\vec{x}_u## and ##\vec{x}_v## satisfy ##\vec{x}_u[f] = \partial_uf## and ##\vec{x}_v[f] = \partial_vf##. This is a common theme in geometry to define objects in a way that agrees with our intuition from Euclidian space but also allows us to generalize to non-Euclidian spaces.
For an arbitrary vector acting on a function, we have
$$X[f] = X^i \frac{\partial f}{\partial x^i} $$
This gives the expression for X written in the coordinate basis ##\frac{\partial}{\partial x^i}##:
$$X = X^i \frac{\partial}{\partial x^i}$$
We can then define the tangent plane at this point ##T_p M## as the set of all velocity vectors at that point for all possible curves passing through ##p##. When you're dealing with vectors on a manifold, one cannot specify a "direction" in the Euclidian sense by referencing some ambient space; rather, we say that the vector pointing in a direction (say the ##u## direction) takes the ##u## derivative of a function. Also, these vectors do have a magnitude if we are working with a Riemannian manifold, where the components of the metric are given by
##g_{i j} = < \frac{\partial}{\partial x^i} , \frac{\partial}{\partial x^j}> ##

Thanks Brian ... appreciate your help ...

Still reflecting on what you have said ...

Peter

 

[Brian T]

Thanks Brian ... appreciate your help ...

Still reflecting on what you have said ...

Peter

No problem, let me know if you have any explicit questions. It took me awhile to get used to the concept of a vector as a derivative as well.

 

[Math Amateur]

Thanks Brian ... most reassuring ...

Peter