- #1
Alex126
- 84
- 5
Homework Statement
An object of mass m is rotating, hanging from a string of known length l. The string is attached to a pole, which rotates with a known angular velocity ω and forms a to-be-determined angle α with the string. Find α.
I think I have solved it (the numbers match the results of the book), but I could use a double-check from you veterans :D
Homework Equations
F = m*g for the weight force of an object.
Centripetal Force = m*ω2*r, with ω2*r = centripetal acceleration (a).
The Attempt at a Solution
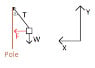
I drew the free-body diagram (imagine the object rotating "into" the screen):
T = tension
F = centripetal force
W = weight
So on the X axis and Y axis we have:
X axis:
Tx + F = 0
T*sin(α) + m*a = 0
Y axis:
Ty - W = 0
T*cos(α) - m*g = 0
=> T = mg/cos(α)
Inputting the T found on the Y axis into the X equation:
mg*sin(α)/cos(α) + ma = 0
g*sin(α)/cos(α) + a = 0
Since a = ω2*r, and the radius is also the length of the rope multiplied by the sine of α (the rope is the hypotenuse of a triangle; in the picture the rope would be aligned with the vector T, and the radius would be aligned with the force F), then a = ω2*l*sin(α)
So the equation becomes:
g*sin(α)/cos(α) + ω2*l*sin(α) = 0
sin(α) * (g/cos(α) + ω2*l) = 0
1. sin(α) = 0 => α = 0
2. g/cos(α) + ω2*l = 0
=> g + ω2*l*cos(α) = 0
=> cos(α) = -g/(ω2*l)
(Then input the numbers and calculate the arc-cos)
Are there any inaccuracies/mistakes, or is this how it's supposed to be done?
Also, with these results (α = 0; the other angle α is impossible because it gives a cos(α) < -1), I'm not sure how to interpret the solution. With α = 0, then r = 0, so a = 0, so F = 0. But with a centripetal force = 0, how can there be circular motion at all? The premise of the problem is that we have an angular velocity, so the object IS rotating, but then it turns out that the angle is zero, so it's "spinning on the center of the circle (and the circle is a single point, which in this case would be the pole)"). Is this still considered circular motion, even if r = 0, or does this have a different name?
Attachments
Last edited: