- #1
- 2,355
- 10
I. Overview
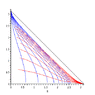
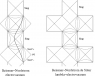
Penrose-Carter diagrams are one of the most useful techniques for visualizing the global causal structure of a Lorentzian manifold. Their most distinctive features are
Penrose diagrams are most often drawn for spacetimes which happen to be spherically symmetric, and are often drawn with the angular coordinates suppressed to make a two-dimensional diagram. But they can be constructed for cylindrical and planar symmetry and are equally valuable in those situations. In all cases, Penrose diagrams are generally the best way to immediately grasp the nature of global Killing flows (for example, how the global flow associated with "time translation" in the exterior of a black hole may appear as a spatial translation in an interior region).
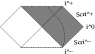
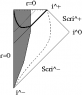
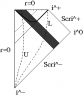
In the spherically symmetric case, Penrose diagrams clearly reveal physically important characteristics of the principle null geodesic congruences. And by studying Penrose diagrams of simple spherically symmetric gravitational collapse models we can gain valuabl e insight into such essential but subtle concepts as
More technical virtues--- whose value becomes apparently only after further explanation--- include the fact that one can integrate over a region of "future lightlike infinity" to compute such interesting quantities as the mass-energy lost from a dynamic isolated gravitating system due to gravitational radiation.
A little appreciated virtue is that Penrose diagrams are also very useful for understanding the relationship between various different classes of observers in a given spacetime model.
In this thread I plan to
My hope is that SA/Ms will painlessly become sufficiently familiar with the uses of Penrose diagrams to make their own and use them in threads in the public areas. With some figures and a bit of handholding, I think at least some readers in the public areas will be able to appreciate that these diagrams are a very efficient way of conveying information.
Penrose-Carter diagrams are one of the most useful techniques for visualizing the global causal structure of a Lorentzian manifold. Their most distinctive features are
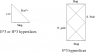
- such a diagram is actually a conformal coordinate chart, so (with angular dimension suppressed, to make a two-dimensional diagram) lines with slope +/-1 represent null geodesics,
- the domain of the chart includes the entire manifold (or almost all of it), so it is a global chart (or very nearly so),
- the domain is compact, so important geometric loci such as
- "the sphere at spatial infinity"
- future lightlike infinity
Penrose diagrams are most often drawn for spacetimes which happen to be spherically symmetric, and are often drawn with the angular coordinates suppressed to make a two-dimensional diagram. But they can be constructed for cylindrical and planar symmetry and are equally valuable in those situations. In all cases, Penrose diagrams are generally the best way to immediately grasp the nature of global Killing flows (for example, how the global flow associated with "time translation" in the exterior of a black hole may appear as a spatial translation in an interior region).
In the spherically symmetric case, Penrose diagrams clearly reveal physically important characteristics of the principle null geodesic congruences. And by studying Penrose diagrams of simple spherically symmetric gravitational collapse models we can gain valuabl e insight into such essential but subtle concepts as
- event horizons, their "teleological" nature, and the standard definition of "black hole",
- apparent horizons
- Cauchy horizons
- various types of cosmological horizons
- wormholes of various types
More technical virtues--- whose value becomes apparently only after further explanation--- include the fact that one can integrate over a region of "future lightlike infinity" to compute such interesting quantities as the mass-energy lost from a dynamic isolated gravitating system due to gravitational radiation.
A little appreciated virtue is that Penrose diagrams are also very useful for understanding the relationship between various different classes of observers in a given spacetime model.
In this thread I plan to
- provide a fairly detailed introduction to the Penrose diagram for Minkowski vacuum, with figures, including a discussion of how to "read" a typical Penrose diagram,
- a more sketchy, but illustrated, introduction to Penrose diagrams for various familiar black hole models: Schwarzschild vacuum, Reissner-Nordstrom electrovacuum, de Sitter lambdavacuum, Kerr vacuum,
- a sketchy illustrated survey of Penrose diagrams for related solutions, including Taub-NUT vacuum, Vaidya models, Maeda wormhole, (perhaps) some CPW models of gravitational collapse,
- a sketchy illustrated survey of Penrose diagrams for some cosmological models, including FRW dusts, the horizon problem, FRW radiation fluids, LTB dusts, Mcvittie spherically symmetric perfect fluid, Senovilla-Vera dust
- a sketchy survey of the Levi-Civita type AIII Petrov D vacuum (E^2 symmetric Kasner vs. Taub planar symmetric vacuum), the Levi-Civita type C Petrov D vacuum and (perhaps) a related solution, the Bonnor-Swaminarayan vacuum, plus transversable wormholes.
My hope is that SA/Ms will painlessly become sufficiently familiar with the uses of Penrose diagrams to make their own and use them in threads in the public areas. With some figures and a bit of handholding, I think at least some readers in the public areas will be able to appreciate that these diagrams are a very efficient way of conveying information.
Last edited: