- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading An Introduction to Rings and Modules With K-Theory in View by A.J. Berrick and M.E. Keating (B&K).
I need help to clarify a remark of B&K regarding ring homomorphisms from the zero or trivial ring ...
The relevant text from B&K reads as follows:

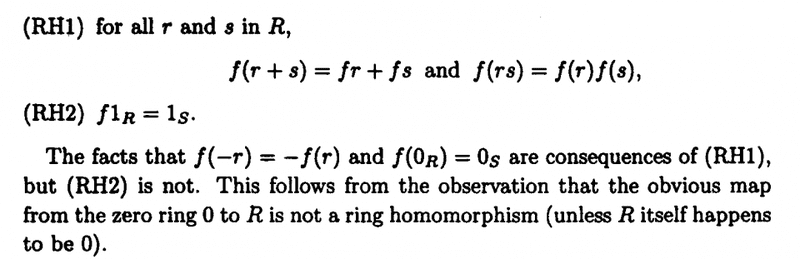
In the above text from B&K's book we read ...
"... ... This follows from the observation that the obvious map from the zero ring to ##R## is not a ring homomorphism (unless ##R## itself happens to be ##0##). ... ... "
I do not understand the above statement that the obvious map from the zero ring to ##R## is not a ring homomorphism (unless ##R## itself happens to be ##0##) ... ...What, indeed do B&K mean by the obvious map from the zero ring to ##R## ... ... ?It seems to me that the obvious map is a homomorphism ... ..Consider the rings ##T, R## where ##T## is the zero ring and ##R## is any arbitrary ring ... so ##T = \{ 0 \}## where ##0 = 1## ...
Then to me it seems that the "obvious" map is ##f( 0_T) = 0_R## ... ... which seems to me to be a ring homomorphism ...
... BUT ... this must be wrong ... but why ... ?
Can someone please clarify the above for me ...
Some help will be very much appreciated ...
Peter
I need help to clarify a remark of B&K regarding ring homomorphisms from the zero or trivial ring ...
The relevant text from B&K reads as follows:
In the above text from B&K's book we read ...
"... ... This follows from the observation that the obvious map from the zero ring to ##R## is not a ring homomorphism (unless ##R## itself happens to be ##0##). ... ... "
I do not understand the above statement that the obvious map from the zero ring to ##R## is not a ring homomorphism (unless ##R## itself happens to be ##0##) ... ...What, indeed do B&K mean by the obvious map from the zero ring to ##R## ... ... ?It seems to me that the obvious map is a homomorphism ... ..Consider the rings ##T, R## where ##T## is the zero ring and ##R## is any arbitrary ring ... so ##T = \{ 0 \}## where ##0 = 1## ...
Then to me it seems that the "obvious" map is ##f( 0_T) = 0_R## ... ... which seems to me to be a ring homomorphism ...
... BUT ... this must be wrong ... but why ... ?
Can someone please clarify the above for me ...
Some help will be very much appreciated ...
Peter