You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Recent content by mathhabibi
-
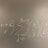
I Partial Differential Equation solved using Products
Is not a double variable product because for there to be only two terms either ##x=y+1## or ##y=x-1##. Good observation though.- mathhabibi
- Post #3
- Forum: General Math
-
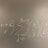
I Partial Differential Equation solved using Products
Using the concepts of Summability Calculus but generalized such that the lower bound for sums and products is also variable, we can prove that the solution to the following PDE: $$P^2\frac{\partial^2P}{\partial x\partial y}=(P^2+1)\frac{\partial P}{\partial x}\frac{\partial P}{\partial...- mathhabibi
- Thread
-
- Tags
- Differential equation Partial
- Replies: 2
- Forum: General Math
-
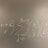
Help with the Separation of Variables and Integration
okay I started my tutoring session will be back later.- mathhabibi
- Post #14
- Forum: Calculus and Beyond Homework Help
-
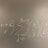
Help with the Separation of Variables and Integration
No problem. I am a bit sketchy with physics but is it true that ##F_n=m\frac{dv}{dt}##?- mathhabibi
- Post #12
- Forum: Calculus and Beyond Homework Help
-
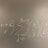
Help with the Separation of Variables and Integration
I'm not sure It will take me some time but from what I am seeing, after integrating you want to solve for ##x_f-x_i##, is that correct?- mathhabibi
- Post #9
- Forum: Calculus and Beyond Homework Help
-
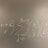
Help with the Separation of Variables and Integration
In the post you said you are solving for v. Be more clear next time. What even is ##\Delta x##?- mathhabibi
- Post #6
- Forum: Calculus and Beyond Homework Help
-
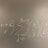
Help with the Separation of Variables and Integration
which is why you should integrate.- mathhabibi
- Post #5
- Forum: Calculus and Beyond Homework Help
-
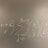
Help with the Separation of Variables and Integration
solve for dv/dx and integrate with respect to x.- mathhabibi
- Post #3
- Forum: Calculus and Beyond Homework Help
-
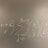
Evaluate the limit of this harmonic series as n tends to infinity
No. It's ##\ln(n)+\gamma## (where ##\gamma## is the Euler-Mascheroni constant). However, ##\ln n## is pretty close to the harmonic numbers so replacing the numbers with that function shouldn't change anything.- mathhabibi
- Post #18
- Forum: Calculus and Beyond Homework Help
-
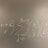
Evaluate the limit of this harmonic series as n tends to infinity
Take the natural log of both sides. Also this isn't the original limit as in the question.- mathhabibi
- Post #16
- Forum: Calculus and Beyond Homework Help
-
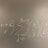
Evaluate the limit of this harmonic series as n tends to infinity
The limit is $$\lim_{n\rightarrow\infty}\left(\frac{H_n}{n^2}\right)^n=\lim_{n\rightarrow\infty}\frac{H^n_n}{n^{2n}}$$Where ##H_n## is the ##n##th harmonic number. The numerator grows much slower than the denominator and so the answer is just ##0##.- mathhabibi
- Post #14
- Forum: Calculus and Beyond Homework Help
-
I Requesting constructive criticism for my paper
I think I can still cite his notebooks somehow. You have contributed something, don't downplay what you've done :)- mathhabibi
- Post #38
- Forum: General Math
-
I Requesting constructive criticism for my paper
After taking in all your feedback, I submitted a new version of my paper, where instead the main result is called the simple result and is defined as a lemma, not a theorem. I also solved the "conjecture" in my paper and replaced it with a theorem, deriving an asymptotic for the roots of the...- mathhabibi
- Post #36
- Forum: General Math
-
I Alternating Harmonic Numbers are cool, spread the word!
There's a small typo in the OP but I couldn't change it. The reflection formula should be $$\bar{H}_x-\bar{H}_{2-x}=\pi\cot(\pi x)+\left(\frac1{2-x}-\frac1{1-x}-\frac1x\right)\cos(\pi x)$$But this doesn't change the proof of the conjecture.- mathhabibi
- Post #5
- Forum: General Math
-
I Alternating Harmonic Numbers are cool, spread the word!
I solved the conjecture. Take a look at the function ##\bar{H}_x-\bar{H}_{2-x}+\ln2##, which is just the RHS of the reflection formula added by ##\ln2##. As ##x\rightarrow-\infty## we have that ##\bar{H}_x-\bar{H}_{2-x}+\ln2\sim\bar{H}_x## and the RHS approaches ##\ln2+\pi\cot(\pi x)##. So we...- mathhabibi
- Post #4
- Forum: General Math