Prove forces of semiholonomic constraints do no virtual work

[Happiness]

I do not see how (2.34) shows that forces of semi-holonomic constraints do no work in the displacements ##\delta q_k## between the varied path and the actual path.

Starting from (2.31), I seem to be able to prove that such forces do do work, in contrary to what is claimed in the paragraph following (2.34).

Using ##Q^{(c)}## to denote the generalised force of constraint, (2.31) becomes

##\frac{d}{dt}\frac{\partial L}{\partial\dot{q_k}}-\frac{\partial L}{\partial q_k}=Q_k^{(c)}##

What follows is basically the reverse of the derivation of Lagrange's equation from D'Alembert's principle, as shown from (1.44) to (1.57).

Using ##L=T-V## and assuming ##V=V(q, t)##,

##\frac{d}{dt}\frac{\partial T}{\partial\dot{q_k}}-\frac{\partial T}{\partial q_k}+\frac{\partial V}{\partial q_k}-Q_k^{(c)}=0##

##\frac{d}{dt}\frac{\partial T}{\partial\dot{q_k}}-\frac{\partial T}{\partial q_k}-Q_k^{(a)}-Q_k^{(c)}=0##, where ##Q^{(a)}## is the generalised applied force.

##\Sigma_k\Big[\frac{d}{dt}\frac{\partial T}{\partial\dot{q_k}}-\frac{\partial T}{\partial q_k}-Q_k^{(a)}-Q_k^{(c)}\Big]\delta q_k=0##

##\Sigma_k\Big[\frac{d}{dt}[\frac{\partial}{\partial\dot{q_k}}(\Sigma_i\frac{1}{2}m_iv_i^2)]-\frac{\partial}{\partial q_k}(\Sigma_i\frac{1}{2}m_iv_i^2)-Q_k^{(a)}-Q_k^{(c)}\Big]\delta q_k=0##

##\Sigma_k\Big[\Sigma_i[\frac{d}{dt}(m_iv_i\cdot\frac{\partial v_i}{\partial\dot{q_k}})-m_iv_i\cdot\frac{\partial v_i}{\partial q_k}]-Q_k^{(a)}-Q_k^{(c)}\Big]\delta q_k=0##

##\Sigma_k\Big[\Sigma_i[\frac{d}{dt}(m_i\dot{r_i}\cdot\frac{\partial r_i}{\partial q_k})-m_i\dot{r_i}\cdot\frac{d}{dt}\frac{\partial r_i}{\partial q_k}]-Q_k^{(a)}-Q_k^{(c)}\Big]\delta q_k=0##

##\Sigma_k\Sigma_i\Big[(m_i\ddot{r_i}\cdot\frac{\partial r_i}{\partial q_k}-(F_i^{(a)}+F_i^{(c)})\cdot\frac{\partial r_i}{\partial q_k}\Big]\delta q_k=0##

##\Sigma_i\Big[m_i\ddot{r_i}\cdot\delta r_i-(F_i^{(a)}+F_i^{(c)})\cdot\delta r_i\Big]=0##

##\Sigma_i(F_i^{(a)}+F_i^{(c)}-\dot{p_i})\cdot\delta r_i=0##

If the virtual work done by ##F_i^{(c)}## is ##0##, we have

##\Sigma_i(F_i^{(a)}-\dot{p_i})\cdot\delta r_i=0##, which is (1.45)

Using the same steps as the derivation from (1.45) to (1.57), (that is, the reverse of what was done earlier) we get (1.57), which is different from (2.31).

We thus conclude that forces of semi-holonomic constraints must do work in virtual displacements.

What's wrong?

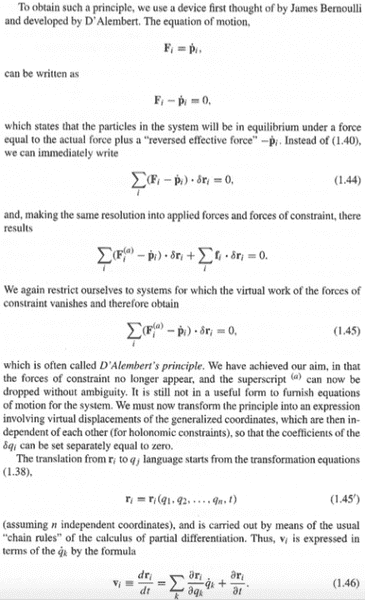
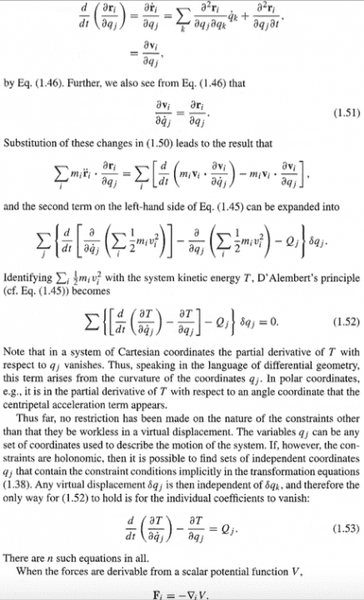
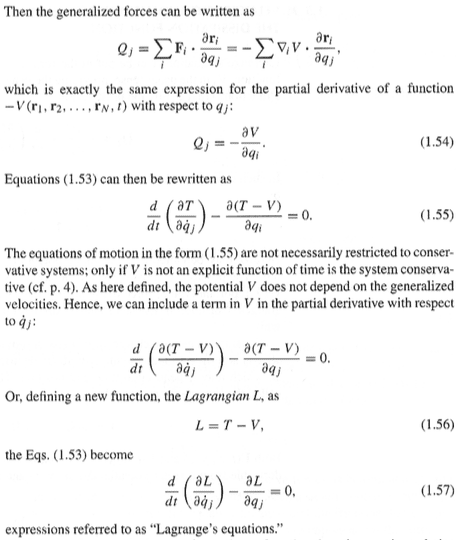
Below is the derivation of Lagrange's equation using D'Alembert's principle:

 

[vanhees71]

From which textbook did you get this? It should be very clear that the usual d'Alembert principle and what's presented there (nowadays known as "Vakonomic Dynamics") lead to different equations of motion, and the principle of vanishing virtual work for general (even for linearly) nonholonomic constraints is not fulfilled. There is some evidence that it does not lead to the correct description of simple model systems like a rolling ball on a rotating table or a skate running down an inclined plane:

http://dx.doi.org/10.1016/0020-7462(95)00024-0
https://www.researchgate.net/profile/Gaetano_Zampieri/publication/224039915_Nonholonomic_versus_Vakonomic_Dynamics/links/544fb9a50cf201441e934bcd.pdf

 

[Happiness]

From which textbook did you get this? It should be very clear that the usual d'Alembert principle and what's presented there (nowadays known as "Vakonomic Dynamics") lead to different equations of motion, and the principle of vanishing virtual work for general (even for linearly) nonholonomic constraints is not fulfilled. There is some evidence that it does not lead to the correct description of simple model systems like a rolling ball on a rotating table or a skate running down an inclined plane:

http://dx.doi.org/10.1016/0020-7462(95)00024-0
https://www.researchgate.net/profile/Gaetano_Zampieri/publication/224039915_Nonholonomic_versus_Vakonomic_Dynamics/links/544fb9a50cf201441e934bcd.pdf

It is from Classical Mechanics 3rd ed. by Goldstein, Poole & Safko.

I should have been clearer in my question. The book does not claim that all semi-holonomic constraint forces do no virtual work. What it says is that for semi-holonomic constraint forces that do no virtual work, d'Alembert principle and Hamilton's principle will give us the same equations of motion.

There is some evidence that it does not lead to the correct description of simple model systems like a rolling ball on a rotating table or a skate running down an inclined plane

Does the "it" here refer to "d'Alembert principle"? The reason it does not lead to the correct description is because the constraint forces in these system do not meet the requirement of d'Alembert principle, i.e., they do not have vanishing virtual work, or they meet the requirement and d'Alembert principle is wrong?

 

[Happiness]

##\Sigma_i(F_i^{(a)}+F_i^{(c)}-\dot{p_i})\cdot\delta r_i=0##

If the virtual work done by ##F_i^{(c)}## is ##0##, we have

##\Sigma_i(F_i^{(a)}-\dot{p_i})\cdot\delta r_i=0##, which is (1.45)

This step is wrong I believe.

Using ##Q^{(c)}## to denote the generalised force of constraint, (2.31) becomes

##\frac{d}{dt}\frac{\partial L}{\partial\dot{q_k}}-\frac{\partial L}{\partial q_k}=Q_k^{(c)}##

We should interpret ##Q_k^{(c)}## as the (generalised) applied forces needed to keep the motion of the system unchanged when the (generalised) constraint forces are removed. In replacing the constraint forces with applied forces that are the same in every way, we increase the degree of freedom that the system can move (because there are no longer any constraint forces to restrict the system). The reason the system's motion is unchanged even though it can now move in new degrees of freedom is because, with this re-interpretation, the applied forces change the Lagrangian of the system from ##L## to ##L+\lambda f##, where ##\lambda## is the undetermined Lagrange multiplier and ##f=0## is the equation of constraint.

Originally, with the constraint forces, the ##q_k## are not all independent (they are related by the equation of constraint). But with this re-interpretation, the ##q_k## are now all independent. This independence then allows us to get the equations of motion from Hamilton's principle.

The virtual work done by ##F_i^{(c)}## is ##0## means ##\Sigma_iF_i^{(c)}\cdot\delta r_i=0## is only true for virtual displacements ##\delta r_i## that are consistent with the constraint. But with this re-interpretation, the virtual displacements ##\delta r_i## no longer needs to be consistent with the constraint, since the re-interpreted system has no constraint. Hence, ##\Sigma_iF_i^{(c)}\cdot\delta r_i\neq0## in the above step.

But the constraint forces may have vanishing virtual work and the claim that in such cases, d'Alembert principle gives the same equation of motion as Hamilton's principle remains to be proved:

Let ##\delta s_i## be the virtual displacements that are consistent with the constraint, and ##\delta r_i## be (as before) the virtual displacements that no longer needs to be consistent with the constraint, i.e., the virtual displacements in the re-interpreted system.

Let ##f_i^{(c)}## be the constraint forces in the original system, and ##F_i^{(c)}## be the corresponding required applied forces in the re-interpreted system.

Show that ##\Sigma_i(F_i^{(a)}+F_i^{(c)}-\dot{p_i})\cdot\delta r_i=0## if and only if ##\Sigma_i\Big[(F_i^{(a)}-\dot{p_i})\cdot\delta s_i+f_i^{(c)}\cdot\delta s_i\Big]=0## or more simply ##\Sigma_if_i^{(c)}\cdot\delta s_i=0##.

 

[vanhees71]

I'm not a great fan of the d'Alembert principle. I prefer the Hamilton principle of least action, because it's more universal and clearer in its application (that's of course just a personal taste).

From the action-principle point of view nonholonomic constraints are a constraints of the "allowed" variations in the Hamilton principle rather than constraints of the manifold of physical configurations. That's why for holonomic constraints you can as well eliminate all constraints by introduction of appropriate generalized coordinates and use as many generalized coordinates as you have unconstrained degrees of freedom.

Nonholonomic constraints describe situations, where you have more degrees of freedom in the large than locally (infinitesimal). The paradigmatic example is a skate on an inclined plane. Macroscopically you have three degrees of freedom: Two coordinates ##(x,y)## of the center of mass of the skate on the inclined plane and the angle ##\phi## between the ##x##-axis (which we choose as the axis pointing downhill) and the skate. Microscopically it can only slide tangential to its direction, which leads to the constraint on the variations of the degrees of freedom
$$\delta f=\delta x \sin \phi-\delta y \cos \phi=0,$$
which says that locally the skate's center of mass can only slide in direction of the skate. The Lagrangian reads
$$L=\frac{m}{2}(\dot{x}^2+\dot{y}^2)+mgx.$$
The variational principle tells you to extremize the action, but you have to take into account the non-holonomic constraint, which you implement by introducing a Lagrange multiplier for the variation (which is the difference between this (imho correct) approach to the "vakonomic dynamics"), i.e., you make
$$\delta S=\int \mathrm{d} t (\delta L+\lambda \delta f) \stackrel{!}{=}0.$$
Generally you have (for the skleronomic case) constraints of the form (Einstein summation implied from here on)
$$\delta f_i=A_{ik}(q) \delta q_k.$$
This gives
$$\delta S=\int \mathrm{d} t (\delta L+\lambda_i \delta f_1)=0.$$
The equation of motion follows as usual
$$\delta S=\int \mathrm{d} t \delta q_k \left (\frac{\partial L}{\partial q_k} -\frac{\mathrm{d}}{\mathrm{d} t} +\lambda_i A_{ik} \right) \stackrel{!}{=}0.$$
Now, in the spirit of the Lagrange-multplier method, you can take the ##\delta q_k## as unconstrained and solve the equations of motion for the ##q_k## and ##\lambda_i##
$$\frac{\partial L}{\partial q_k} -\frac{\mathrm{d}}{\mathrm{d} t} +\lambda_i A_{ik} =0$$
with the constraints
$$A_{ik} \dot{q}_k=0.$$
In contradiction to these equations of motion the vaskonomic dynamics as described in Goldstein (I'm a bit surprised that this wrong idea is proposed in this textbook; is this a new edition?) lead in general to different equations of motion, namely (2.24) and (2.25). Only if the nonholonomic constraints are integrable, i.e., if
$$\frac{\partial A_{ik}}{\partial q_j}-\frac{\partial A_{ij}}{\partial q_k}=0$$
and thus can be reformulated as holonomic constraints (at least in principle).

In the case of the skate, the vaskonomic equations lead to quite weird results which most probably are not in accordance with experience. For further details, see

Lewis, Andrew D., and Richard M. Murray. "Variational principles for constrained systems: theory and experiment." International Journal of Non-Linear Mechanics 30.6 (1995): 793-815.
http://qspace.library.queensu.ca/dspace/bitstream/1974/36/1/1994g_letter.pdf

Zampieri, G. (2000). Nonholonomic versus vakonomic dynamics. Journal of Differential Equations, 163(2), 335-347.
https://www.researchgate.net/profile/Gaetano_Zampieri/publication/224039915_Nonholonomic_versus_Vakonomic_Dynamics/links/544fb9a50cf201441e934bcd.pdf

Flannery, M. R. (2005). The enigma of nonholonomic constraints. American Journal of Physics, 73(3), 265-272.
http://www.phys.ufl.edu/~maslov/classmech/flannery.pdf

 

[Happiness]

In contradiction to these equations of motion the vaskonomic dynamics as described in Goldstein (I'm a bit surprised that this wrong idea is proposed in this textbook; is this a new edition?) lead in general to different equations of motion, namely (2.24) and (2.25).

I believe there is no new edition after this one.

Does that mean d'Alembert principle and Hamilton's principle lead to (2.24) in the same form but differ in their expressions for ##Q_k## on the RHS?

The book claims that we can't apply Hamilton's principle to any kinds of semi-holonomic constraints, but only to those that satisfy the requirement of d'Alembert's principle, i.e., those that do no virtual work.

If Hamilton's principle is the right approach while d'Alembert's principle is the wrong one, when it comes to semi-holonomic constraints, isn't it strange that for Hamilton's principle to work, it has to satisfy the requirement of d'Alembert's principle? Suppose d'Alembert's principle is wrong, and the book's claim is true, then there must be a way for virtual work to enter Hamilton's principle, a way independent of d'Alembert's principle. How exactly does the requirement of no virtual work enter Hamilton's principle independently?

 

[vanhees71]

The d'Alembert principle for nonholonomic constraints leads to the correct equation (see the papers I cited, where you find empirical evidence for simple cases), while the vaskonomic equations don't. They lead to even paradoxical behavior. The two approaches are equivalent only for holonomic constraints, i.e., if and only if the nonholonomic constraints ##A_{k}(q) \delta q^k=0## are integrable. Locally (in simply connected regions of the configuration manifold) that's equivalent to the vanishing of the curl, i.e.,
$$\frac{\partial A_k}{\partial q^j}-\frac{\partial A_j}{\partial q^k}=0.$$

 

[Happiness]

Is the vakonomic method same as the method of Lagrange undetermined multiplier in the book? What's the reason the vakonomic method doesn't work in non-holonomic constraints? Is it because the method wrongly assumes that all critical points of an action are stationary points? Which implies that an action may have singular points, at which the action is not differentiable?

 

[vanhees71]

The reason is that, except for holonomic constraints, i.e., if the nonholonomic constraints given are in fact total differentials, you impose different constraints. In the d'Alembert principle you define the constraints in terms of virtual displacements. That's equivalent to define the constraints in terms of the variations, i.e., you constrain the allowed variations around the true trajectory. If you have proper nonholonomic constraints, these constraints cannot be substituted by defining submanifolds in configuration space. That makes sense physically, because in such cases you have less degrees of freedom in the infinitesimal neighborhood of any point of the trajectory than degrees of freedom in the large (think carefully about the example of the skate on a declined plane). In this case the constraints are in terms of the variation (i.e., the virtual displacement in d'Alembert's principle):
$$f_{\alpha}(t,q) \delta q^{\alpha}=0.$$
If ##f## is not explicitly time dependent the constraned is called skleronomic, otherwise rheonomic. There's no formal difference in the treatment in d'Alembert's or Hamilton's principle. You introduce Lagrange multipliers for the variations (see my posting above).

In case of the vakonomic equations you impose different constraints for the true trajectory, i.e., you write
$$f_{\alpha}(t,q) \dot{q}^{\alpha}=0$$
for the constraints. Thus you introduce the constraints in Hamilton's principle by introducing the corresponding Lagrange multipliers. As you can easily see by comparison, the resulting equations of motion differ from those of the d'Alembert principle in the expression for the constraint forces ##Q^{\alpha}##. They are equivalent if and only if the constraints are holonomic and skleronomic, i.e., if the differentials on the left-hand side of the constraints of the variation are a total differentials or the vakonomic constraints are total time derivatives of a function ##F(q)##:
$$\frac{\mathrm{d} F}{\mathrm{d} t}=\dot{q}^{\alpha} \frac{\partial F}{\partial q^{\alpha}}.$$

 

[Happiness]

The reason is that, except for holonomic constraints, i.e., if the nonholonomic constraints given are in fact total differentials, you impose different constraints. In the d'Alembert principle you define the constraints in terms of virtual displacements. That's equivalent to define the constraints in terms of the variations, i.e., you constrain the allowed variations around the true trajectory. If you have proper nonholonomic constraints, these constraints cannot be substituted by defining submanifolds in configuration space. That makes sense physically, because in such cases you have less degrees of freedom in the infinitesimal neighborhood of any point of the trajectory than degrees of freedom in the large (think carefully about the example of the skate on a declined plane). In this case the constraints are in terms of the variation (i.e., the virtual displacement in d'Alembert's principle):
$$f_{\alpha}(t,q) \delta q^{\alpha}=0.$$
If ##f## is not explicitly time dependent the constraned is called skleronomic, otherwise rheonomic. There's no formal difference in the treatment in d'Alembert's or Hamilton's principle. You introduce Lagrange multipliers for the variations (see my posting above).

In case of the vakonomic equations you impose different constraints for the true trajectory, i.e., you write
$$f_{\alpha}(t,q) \dot{q}^{\alpha}=0$$
for the constraints. Thus you introduce the constraints in Hamilton's principle by introducing the corresponding Lagrange multipliers. As you can easily see by comparison, the resulting equations of motion differ from those of the d'Alembert principle in the expression for the constraint forces ##Q^{\alpha}##. They are equivalent if and only if the constraints are holonomic and skleronomic, i.e., if the differentials on the left-hand side of the constraints of the variation are a total differentials or the vakonomic constraints are total time derivatives of a function ##F(q)##:
$$\frac{\mathrm{d} F}{\mathrm{d} t}=\dot{q}^{\alpha} \frac{\partial F}{\partial q^{\alpha}}.$$

I am lost. Do you have a simple definition of the vakonomic method?

I think those papers that you mentioned are too advanced for me at this moment. I don't understand the notation ##\delta q^{\alpha}##.

"There's no formal difference in the treatment in d'Alembert's or Hamilton's principle." Does this apply to all kinds of constraints, holonomic and non-holonomic?

 

[Happiness]

The following is from the last paper you mentioned:
Flannery, M. R. (2005). The enigma of nonholonomic constraints. American Journal of Physics, 73(3), 265-272.
http://www.phys.ufl.edu/~maslov/classmech/flannery.pdf

Comparing (21) with (16) for ##j=1,2,...,n-c##,

##\lambda_k\frac{\partial f_k}{\partial q_j}=0##

For simplicity, assume there is only one equation of constraint, i.e., ##c=1##.

##\lambda\frac{\partial f}{\partial q_j}=0##

##\lambda\neq0##, otherwise there is no force of constraint.

We must have ##\frac{\partial f}{\partial q_j}=0## for ##j=1,2,n-1##.

But this contradicts the assumption of ##f=f(q_1,q_2,...,q_n,t)=0## (3).

What's wrong?

EDIT: I think I found the mistake, ##\frac{\partial L}{\partial\dot{q_j}}##, ##\frac{\partial L}{\partial\dot{q_j}}## and ##Q_j^{NP}## in (16) are not the same as those in (21). And this is also the mistake in my original question! (2.31) and (1.57) are in fact consistent.

 

[PhDeezNutz]

Just to confirm. @vanhees71 is equation (2.25 ) in the OP wrong?

I am able to derive the same equation through means other than the one shown in the OP and I'm pretty convinced of its validity. I just wanted to confirm (or deny).