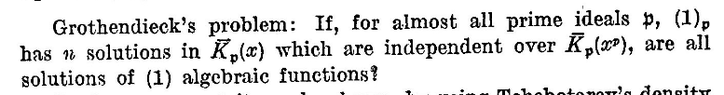- #1
mathmari
Gold Member
MHB
- 5,049
- 7
Hey! 
I am looking at the following:
View attachment 5008
View attachment 5009
View attachment 5010 View attachment 5011
View attachment 5012 I haven't really understood the proof...
Why do we consider the differential equation $y'=P(x)y$ ? (Wondering)
Why does the sentence: "If $(3)_{\mathfrak{p}}$ has a solution in $\overline{K}_{\mathfrak{p}}(x)$, then $(3)_{\mathfrak{p}}$ has also a solution $y_{\mathfrak{p}}$ in $\overline{K}_{\mathfrak{p}}[x]$." stand? (Wondering)
I am looking at the following:
View attachment 5008
View attachment 5009
View attachment 5010 View attachment 5011
View attachment 5012 I haven't really understood the proof...
Why do we consider the differential equation $y'=P(x)y$ ? (Wondering)
Why does the sentence: "If $(3)_{\mathfrak{p}}$ has a solution in $\overline{K}_{\mathfrak{p}}(x)$, then $(3)_{\mathfrak{p}}$ has also a solution $y_{\mathfrak{p}}$ in $\overline{K}_{\mathfrak{p}}[x]$." stand? (Wondering)