Nuclear Spin and Selection Rules

[Tainty]

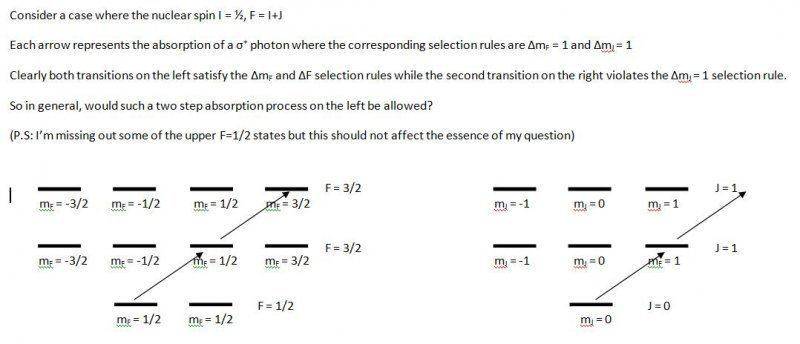
Suppose we define the total angular momentum as F = I+J where I is the nuclear spin angular momentum and J is the total electronic angular momentum. m_F and m_J are the respective magnetic quantum numbers.

The relevant F selection rules are delta_m_F = 0, 1 and -1, delta_F = 0, 1 and -1.
And similarly, the J selection rules are delta_m_J = 0, 1 and -1, delta_J = 0, 1 and -1.

My question is, when considering a transition involving nuclear spin, in addition to satisfying the delta_F and delta_m_F selection rules, do we have to also satisfy the delta_J and delta_m_J selection rules at the same time?

A specific example involving two successive transitions is attached. The transitions satisfy the delta_F and delta_m_F selection rules but violate the delta_m_J selection rules. Would such a two step process be allowed or forbidden? I'd appreciate any insight. Thank you!

 

[DrDu]

I don't think so. Nuclear and electronic absorption are due to coupling of the em field to different systems (nuclear vs. electronic). Coupling to the nuclear part will in general not change the electronic wavefunction and vice versa.

 

[DrClaude]

In the presence of the hyperfine interaction, ##M_J## is no longer a good quantum number, i.e., you can't use it to label an eigenstate of the Hamiltonian. Therefore, it is meaningless to discuss ##\Delta M_J##. For instance, in your diagram on the right, absorption of a ##\sigma^+## photon does not anymore correspond to a ##\Delta M_J = +1## transition: because of the hyperfine interaction, you will get a mixture of ##M_J##'s.

 

[DrDu]

But even the states resulting from hyperfine splitting can be written as superpositions of M_J eigenstates. These eigenstates then fulfill individually the selection rules.

 

[DrClaude]

But even the states resulting from hyperfine splitting can be written as superpositions of M_J eigenstates. These eigenstates then fulfill individually the selection rules.

Yes, but the atom will generally be found in a superposition of ##M_J##'s to start with.

To make things clearer: if the transition is allowed when considering ##\Delta M_F##, it has to be allowed when considering ##\Delta M_J##. Within the ##| J, M_J \rangle## states that make up the initial state ##| F, M_F \rangle##, and within the ##| J', M_J' \rangle## states that make up the final state ##| F', M_F' \rangle##, if the transition has ##\Delta M_F =0,\pm1##, then there is at least one pair of ##| J, M_J \rangle## and ##| J', M_J' \rangle## for which ##\Delta M_J=0,\pm1##.

 

[Tainty]

Thank you very much for those replies. Both of you hit the nail on the head in terms what I have been confused about.

I agree that if the transition is Δm_F allowed it should be automatically Δm_J allowed based on superposition even though J and m_J are strictly no longer good quantum numbers. But this appears to contradict the scenario in the diagram I earlier attached. For a system with nuclear spin, the left figure would be Δm_F (+1) allowed but not correspondingly (right figure) Δm_J allowed. Where is the flaw in my argument?

Thanks again!

 

[DrDu]

Let's consider something simple, like nuclear magnetic resonance. There J and M_J doesn't change as there is no electronic transition at all, and ##\Delta F=\Delta I## with selection rules only for F and I. What I meant before, is that the interaction is proportional to ##d\cdot E## for electronic transitions and ##I\cdot B## for nuclear transitions, each with it's own selection rules for J and I, respectively. A transition is due to either the first or the second term, but not both of them at the same time.

 

[DrClaude]

Lets consider the first absorption. The atom goes from ##|F=1/2, M_F=-1/2\rangle## to ##|F=3/2, M_F=1/2\rangle##. Using the notation ##|I,M_I,J,M_J\rangle##, we have
\begin{align*}
|F=1/2, M_F=-1/2\rangle &= |1/2, -1/2, 0 , 0\rangle \\
|F=3/2, M_F=1/2\rangle &= \frac{1}{\sqrt{3}} |1/2, -1/2, 1 , 1\rangle + \sqrt{\frac{2}{3}} |1/2, 1/2, 1 , 0\rangle
\end{align*}
which is allowed since we have ##\Delta M_J = +1## from ##|1/2, -1/2, 0 , 0\rangle## to ##|1/2, -1/2, 1 , 1\rangle##.

In the second step, we have
\begin{align*}
|F=3/2, M_F=1/2\rangle &= \frac{1}{\sqrt{3}} |1/2, -1/2, 1 , 1\rangle + \sqrt{\frac{2}{3}} |1/2, 1/2, 1 , 0\rangle \\
|F=3/2, M_F=3/2\rangle &= |1/2, 1/2, 1 , 1\rangle \\
\end{align*}
which is allowed since we have ##\Delta M_J = +1## from ##|1/2, 1/2, 1 , 0\rangle## to ##|1/2, 1/2, 1 , 1\rangle##.

What you have to consider is that while you are doing only ##\sigma^+## transitions, the atom will not end up in purely the ##|1/2, -1/2, 1 , 1\rangle## state, as it is coupled by hyperfine interaction to ##|1/2, 1/2, 1 , 0\rangle##. Even if you were to prepare the atom in the state ##|1/2, -1/2, 1 , 1\rangle##, after a certain time you will have a significant superposition of the two states.

 

[Tainty]

That makes a lot of sense! Thanks folks. I will try to digest and understand this further.

 

[Tainty]

After further thought, I realize I have some difficulty reconciling a few issues, particularly with regards to a physical understanding of the entire picture.

Firstly, I realize that in my initial query, I had omitted any mention of the m_I quantum number. Does its selection rules echo that of the m_J quantum number? (I am assuming that the answer to this is yes for the questions that follow, otherwise just ignore them.)

Secondly, I also realize that even though the m_J and m_I selection rules are automatically satisfied due to superposition, each eigenstate does not satisfy BOTH at the same time, which is what DrDu pointed out earlier. This perhaps has been the real source of 'discomfort' and confusion for me. In some sense, i have difficulty accepting the fact that the selection rules are truly satisfied. This problem (or rather confusion) is exacerbated when i consider a multiple step absorption process, where, as DrClaude shows in his response to my example, the eigenstate that satisfies Δm_J=+1 in the first step is NOT the eigenstate that satisfies Δm_J=+1 in the second step.

Could any of you assist in providing further physical insight? Thank you!

 

[DrDu]

Maybe you could explain in some more detail which kind of experiment or system you are referring too?

 

[Tainty]

Hi DrDu, I am just trying to test my understanding of this topic, which pertains to the broader field of spin polarization, through various thought experiments. These questions arise as I try to improve my fundamental understanding of the topic.

 

[DrDu]

Yes, but I think it would be appropriate to analyze a concrete example (of your choice).

 

[Tainty]

Ok, suppose we consider the example that DrClaude expanded upon.

Lets consider the first absorption. The atom goes from |F=1/2,MF=−1/2⟩|F=1/2, M_F=-1/2\rangle to |F=3/2,MF=1/2⟩|F=3/2, M_F=1/2\rangle. Using the notation |I,MI,J,MJ⟩|I,M_I,J,M_J\rangle, we have

|F=1/2,MF=−1/2⟩|F=3/2,MF=1/2⟩=|1/2,−1/2,0,0⟩=13√|1/2,−1/2,1,1⟩+23−−√|1/2,1/2,1,0⟩​

\begin{align*} |F=1/2, M_F=-1/2\rangle &= |1/2, -1/2, 0 , 0\rangle \\ |F=3/2, M_F=1/2\rangle &= \frac{1}{\sqrt{3}} |1/2, -1/2, 1 , 1\rangle + \sqrt{\frac{2}{3}} |1/2, 1/2, 1 , 0\rangle \end{align*}

 

[Tainty]

In the 2nd equation, the first term (eigenstate) on the RHS satisfies Δm_J=+1 but not Δm_I=+1 whereas the second term (eigenstate) on the RHS satisfies Δm_I=+1 but not Δm_J=+1. In other words, the selection rules for both m_J and m_I are not satisfied at the same time for each eigenstate. If this statement is true, what is the physical interpretation?

(P.S: Like I mentioned earlier, I'm assuming that the selection rules for m_I are the same as those for m_J.)

 

[DrClaude]

In the 2nd equation, the first term (eigenstate) on the RHS satisfies Δm_J=+1 but not Δm_I=+1 whereas the second term (eigenstate) on the RHS satisfies Δm_I=+1 but not Δm_J=+1. In other words, the selection rules for both m_J and m_I are not satisfied at the same time for each eigenstate. If this statement is true, what is the physical interpretation?

(P.S: Like I mentioned earlier, I'm assuming that the selection rules for m_I are the same as those for m_J.)

The selection rule comes from conservation of angular momentum. If both ##M_I## and ##M_J## were changing here, then angular momentum would not be conserved. Only one ##\Delta M## can have a value of +1.