- #1
bob900
- 40
- 0
I'm having trouble understanding the derivation of the the position operator eigenfunction in Griffiths' book :
How is it "nothing but the Dirac delta function"?? (which is not even a function).
Couldn't [itex]g_{y}(x)[/itex] simply be a function like (for any constant y)
[itex]g_{y}(x)[/itex] = 1 | x=y
[itex]g_{y}(x)[/itex] = 0 | elsewhere
Then we have, [itex]x * g_{y}(x) = y*g_{y}(x)[/itex], so it is indeed an eigenfunction of the x operator. And it happens to be a normal function. So why does he say it's 'nothing but Delta'?
griffiths said: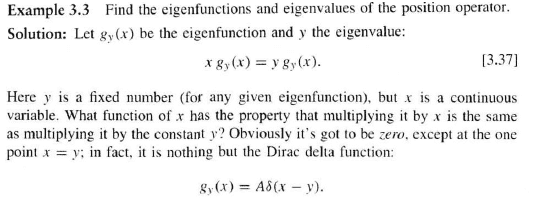
How is it "nothing but the Dirac delta function"?? (which is not even a function).
Couldn't [itex]g_{y}(x)[/itex] simply be a function like (for any constant y)
[itex]g_{y}(x)[/itex] = 1 | x=y
[itex]g_{y}(x)[/itex] = 0 | elsewhere
Then we have, [itex]x * g_{y}(x) = y*g_{y}(x)[/itex], so it is indeed an eigenfunction of the x operator. And it happens to be a normal function. So why does he say it's 'nothing but Delta'?