Frobenius Theorem - Bresar, Theorem 1.4 ....

[Math Amateur]

I am reading Matej Bresar's book, "Introduction to Noncommutative Algebra" and am currently focussed on Chapter 1: Finite Dimensional Division Algebras ... ...

I need help with some aspects of the proof of Theorem 1.4 ... ...

Theorem 1.4 reads as follows:

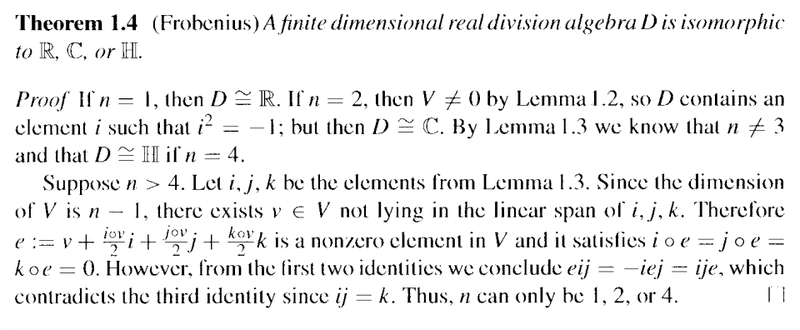
Questions 1(a) and 1(b)

In the above text by Matej Bresar we read the following:

" ... ... Suppose ##n \gt 4##. Let ##i, j, k## be the elements from Lemma 1.3.

Since the dimension of ##V## is ##n - 1##, there exists ##v \in V## not lying in the linear span of ##i, j, k##.

Therefore ##e := v + \frac{i \circ v}{2} i + \frac{j \circ v}{2} j + \frac{k \circ v}{2} k##

is a nonzero element in ##V## and it satisfies ##i \circ e = j \circ e = k \circ e = 0##... ... "My questions are as follows:

(1a) Can someone please explain exactly why ##e := v + \frac{i \circ v}{2} i + \frac{j \circ v}{2} j + \frac{k \circ v}{2} k## is a nonzero element in ##V##?

(1b) ... ... and further, can someone please show how ##e := v + \frac{i \circ v}{2} i + \frac{j \circ v}{2} j + \frac{k \circ v}{2} k## satisfies ##i \circ e = j \circ e = k \circ e = 0##?Question 2

In the above text by Matej Bresar we read the following:

" ... ... However, from the first two identities we conclude ##eij = -iej = ije##, which contradicts the third identity since ##ij = k## ... ... "I must confess Bresar has lost me here ... I'm not even sure what identities he is referring to ... but anyway, can someone please explain why/how we can conclude that ##eij = -iej = ije## and, further, how this contradicts ##ij = k##?
Hope someone can help ...

Help will be appreciated ... ...

PeterThe above post refers to Lemma 1.3.

Lemma 1.3 reads as follows:

=====================================================

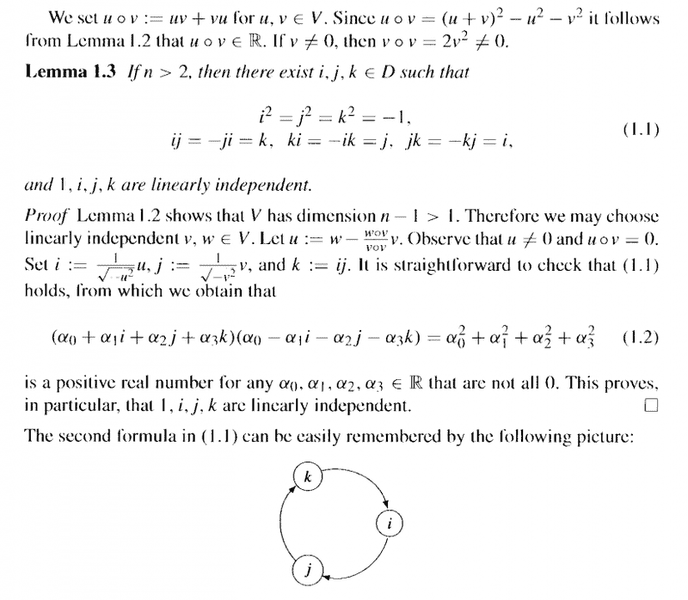
In order for readers of the above post to appreciate the context of the post I am providing pages 1-4 of Bresar ... as follows ...

 

[fresh_42]

If we assume ##e := v+\frac{i\circ v}{2}i+\frac{j\circ v}{2}j+\frac{k\circ v}{2}k=0## then ##v=-\frac{i\circ v}{2}i-\frac{j\circ v}{2}j-\frac{k\circ v}{2}k##. Now this would imply (in contrast to our assumption) that ##v \in lin_\mathbb{R}\{i,j,k\}## if the coefficients ##\frac{i\circ v}{2},\frac{j\circ v}{2},\frac{k\circ v}{2}## were all real. But this follows from the last two lines directly ahead of Lemma 1.3. where he shows that they have to be real.
(Hint: As a general rule: It's always helpful to ask "what if not" when one tries to understand a reasoning. I do this quasi permanently when I read a mathematical text. It's the most important question of all.)

What bothers me a lot more, is the theorem itself, because it only holds for associative division algebras ##D##, since the octonions build a non-associative division algebra of dimension ##8## over the reals. Thus somewhere in the entire process, there must be a hidden usage of associativity. Where? And why doesn't he mention this? Maybe it's within the line with ##eij##. But somewhere it has to be.

The algebra tower goes like this:
##\mathbb{Q}## - prime field of characteristic ##0##
##\mathbb{R}## - limits gained, field, topological closure of ##\mathbb{Q}##, ##\dim_\mathbb{R} = 1##
##\mathbb{C}## - solvability gained, algebraic closure of ##\mathbb{R}##, ##\dim_\mathbb{R} = 2##
##\mathbb{H}## - commutativity lost, skew-field, ##\dim_\mathbb{R} = 4##
##\mathbb{O}## - associativity lost, division ring, ##\dim_\mathbb{R} = 8##

 

[Math Amateur]

If we assume ##e := v+\frac{i\circ v}{2}i+\frac{j\circ v}{2}j+\frac{k\circ v}{2}k=0## then ##v=-\frac{i\circ v}{2}i-\frac{j\circ v}{2}j-\frac{k\circ v}{2}k##. Now this would imply (in contrast to our assumption) that ##v \in lin_\mathbb{R}\{i,j,k\}## if the coefficients ##\frac{i\circ v}{2},\frac{j\circ v}{2},\frac{k\circ v}{2}## were all real. But this follows from the last two lines directly ahead of Lemma 1.3. where he shows that they have to be real.
(Hint: As a general rule: It's always helpful to ask "what if not" when one tries to understand a reasoning. I do this quasi permanently when I read a mathematical text. It's the most important question of all.)

What bothers me a lot more, is the theorem itself, because it only holds for associative division algebras ##D##, since the octonions build a non-associative division algebra of dimension ##8## over the reals. Thus somewhere in the entire process, there must be a hidden usage of associativity. Where? And why doesn't he mention this? Maybe it's within the line with ##eij##. But somewhere it has to be.

The algebra tower goes like this:
##\mathbb{Q}## - prime field of characteristic ##0##
##\mathbb{R}## - limits gained, field, topological closure of ##\mathbb{Q}##, ##\dim_\mathbb{R} = 1##
##\mathbb{C}## - solvability gained, algebraic closure of ##\mathbb{R}##, ##\dim_\mathbb{R} = 2##
##\mathbb{H}## - commutativity lost, skew-field, ##\dim_\mathbb{R} = 4##
##\mathbb{O}## - associativity lost, division ring, ##\dim_\mathbb{R} = 8##

Thanks for the help fresh_42 ...

You write:

" ... ... If we assume ##e := v+\frac{i\circ v}{2}i+\frac{j\circ v}{2}j+\frac{k\circ v}{2}k=0## then ##v=-\frac{i\circ v}{2}i-\frac{j\circ v}{2}j-\frac{k\circ v}{2}k##. Now this would imply (in contrast to our assumption) that ##v \in lin_\mathbb{R}\{i,j,k\}## if the coefficients ##\frac{i\circ v}{2},\frac{j\circ v}{2},\frac{k\circ v}{2}## were all real. But this follows from the last two lines directly ahead of Lemma 1.3. where he shows that they have to be real. ... ... "

So ... this shows that v must be nonzero ... but I am still not sure why exactly ##e \in v## ... ... can you help ... ?Also ,,, I remain unsure on exactly why/how defining e as

##e := v+\frac{i\circ v}{2}i+\frac{j\circ v}{2}j+\frac{k\circ v}{2}##

implies that

##i \circ e = j \circ e = k \circ e = 0##?Can you help?

Peter

 

[fresh_42]

Thanks for the help fresh_42 ...

You write:

" ... ... If we assume ##e := v+\frac{i\circ v}{2}i+\frac{j\circ v}{2}j+\frac{k\circ v}{2}k=0## then ##v=-\frac{i\circ v}{2}i-\frac{j\circ v}{2}j-\frac{k\circ v}{2}k##. Now this would imply (in contrast to our assumption) that ##v \in lin_\mathbb{R}\{i,j,k\}## if the coefficients ##\frac{i\circ v}{2},\frac{j\circ v}{2},\frac{k\circ v}{2}## were all real. But this follows from the last two lines directly ahead of Lemma 1.3. where he shows that they have to be real. ... ... "

So ... this shows that v must be nonzero

It implies ##e \neq 0##. ##v\neq 0## is already guaranteed by the choice of ##v \notin lin_\mathbb{R}\{i,j,k\}##.

... but I am still not sure why exactly ##e \in v## ... ... can you help ... ?

Lemma 1.2.: ##V## is a linear subspace. So with ##v,i,j,k \in V## (##v## by assumption, ##i,j,k## by their definition), hence also ##e = v + c_1\cdot i +c_2 \cdot j +c_3 \cdot k \in V## holds.

Also ,,, I remain unsure on exactly why/how defining e as

##e := v+\frac{i\circ v}{2}i+\frac{j\circ v}{2}j+\frac{k\circ v}{2}##

implies that

##i \circ e = j \circ e = k \circ e = 0##?

We have to use the following rules here:

• ##u \circ (r \cdot w) = r \cdot (u \circ w)## for all ##r \in \mathbb{R}## and ##u,w \in V##
• The coefficients ##c_1,c_2,c_3## in the definition of ##e## are all real. (See the last two lines before Lemma 1.3.)
• Finally ##i \circ j = j \circ k = k \circ i = 0## by their definition and ##u \circ u = -2## for all ##u \in \{i,j,k\}##

Now
$$\begin{align*} i \circ e &= i \circ v + i \circ (c_1\cdot i) + i \circ (c_2\cdot j) + i \circ (c_3\cdot k)
\\ &= i \circ v + c_1 \cdot (i \circ i) + c_2 \cdot (i \circ j) + c_3 \cdot (i \circ k)
\\ &= i \circ v - 2 \cdot c_1
\\ &= iv+vi-2 \cdot (\frac{iv}{2}+\frac{vi}{2})
\\ &= 0\end{align*}$$
It's been now the second time Bresar has hidden the essential hint within these two lines ahead of Lemma 1.3. Would have been a lot easier to see, if he had numbered this as some Corollary. Anyway. I admit it's been a bit tricky to see, but I think it would have helped you a lot to "play" with those formulas on your own: for practice and for the feeling of success if you found out. This way, I thank you for giving me this feeling, but it would have been better placed on your side. I know it's frustrating to star at things with the "knowledge" in mind: 'This should be obvious, why can't I see?' - and be ensured I know this from an uncounted number of own experiences, however, I'm afraid it's part of the game.

 

[Math Amateur]

It implies ##e \neq 0##. ##v\neq 0## is already guaranteed by the choice of ##v \notin lin_\mathbb{R}\{i,j,k\}##.

Lemma 1.2.: ##V## is a linear subspace. So with ##v,i,j,k \in V## (##v## by assumption, ##i,j,k## by their definition), hence also ##e = v + c_1\cdot i +c_2 \cdot j +c_3 \cdot k \in V## holds.

We have to use the following rules here:

• ##u \circ (r \cdot w) = r \cdot (u \circ w)## for all ##r \in \mathbb{R}## and ##u,w \in V##
• The coefficients ##c_1,c_2,c_3## in the definition of ##e## are all real. (See the last two lines before Lemma 1.3.)
• Finally ##i \circ j = j \circ k = k \circ i = 0## by their definition and ##u \circ u = -2## for all ##u \in \{i,j,k\}##

Now
$$\begin{align*} i \circ e &= i \circ v + i \circ (c_1\cdot i) + i \circ (c_2\cdot j) + i \circ (c_3\cdot k)
\\ &= i \circ v + c_1 \cdot (i \circ i) + c_2 \cdot (i \circ j) + c_3 \cdot (i \circ k)
\\ &= i \circ v - 2 \cdot c_1
\\ &= iv+vi-2 \cdot (\frac{iv}{2}+\frac{vi}{2})
\\ &= 0\end{align*}$$
It's been now the second time Bresar has hidden the essential hint within these two lines ahead of Lemma 1.3. Would have been a lot easier to see, if he had numbered this as some Corollary. Anyway. I admit it's been a bit tricky to see, but I think it would have helped you a lot to "play" with those formulas on your own: for practice and for the feeling of success if you found out. This way, I thank you for giving me this feeling, but it would have been better placed on your side. I know it's frustrating to star at things with the "knowledge" in mind: 'This should be obvious, why can't I see?' - and be ensured I know this from an uncounted number of own experiences, however, I'm afraid it's part of the game.

Thanks so much for the assistance fresh_42 ... your post was extremely helpful ...

But I need some further help ...

You write:

" ... ... ##u \circ (r \cdot w) = r \cdot (u \circ w)## for all ##r \in \mathbb{R}## and ##u,w \in V## ... ..."

I am having trouble understanding why this is true ...

My thoughts are as follows:##u \circ (r \cdot w) = u (r \cdot w) + ( r \cdot w) u##

##= u( r \cdot w ) + r \cdot ( wu)## ... ... ... ... ... (1)

and

##r \cdot ( u \circ w ) = r \cdot ( uw + wu )##

##= r \cdot (uw ) + r \cdot (wu)## ... ... ... ... ... (2)Now, we require expression (1) to be equal to expression (2) ...

For this to be true we require ...

##u( r \cdot w ) = r \cdot (uw )## ... ... but ... ... why is this true ... the scalar ##r## cannot commute with vectors ##u## or ##w## ... ?Can you help?

Peter***EDIT***

SOLVED?

I have been doning some checking of the axioms for an associative algebra with identity such as a real division algebra D nd have found that a fundamental axiom involving multiplication of elements of D and multiplication by a scalar (i.e. a real ) is the following:

##r \cdot (uw) = ( r \cdot u) w = u ( r \cdot w )##

and this, I think, answers my problem in needing the equality

##u( r \cdot w ) = r \cdot (uw )## Is my reasoning correct?

Peter

 

[fresh_42]

Yes, this is correct. To be honest, I hadn't thought about it very much. Because we don't distinguish between left and right division algebras (rings), scalars (elements of ##\mathbb{R}##) can be applied from both sides with identical result (and ##\circ## is defined with ordinary multiplications).

(You're on a good track questioning everything! )

 

[Math Amateur]

Thanks for all your help fresh_42 ... it has assisted me a great deal in trying to understand division algebras ...

Peter