Depth of a basketball floating on water

[brotherbobby]

Homework Statement

A basketball of mass 600 g and radius 12 cm floats at rest on the surface of water. Calculate the depth ##\boldsymbol{d}## of the ball that's under the liquid surface.

Relevant Equations

(1) Law of floatation : The mass of a floating body is the mass of liquid displaced - ##m_B=\Delta m_L##.
(2) Equation of a spherical surface : ##x^2+y^2+z^2=a^2##, where ##a## is the radius.

Attempt : (Turns out, there is more mathematics in this problem than physics. The crucial part involves the use of vector calculus where one needs to find the volume of a region bounded at the top by a portion of a sphere. That is where am stuck.)

The mass of water displaced by the ball ##\Delta m = 0.6\;\text{kg}##. This amounts to the volume of liquid displaced : ##\Delta V_L = 600\;\text{cm}^3##. This is also the volume of the ball inside liquid : ##\Delta V_B = 600\;\text{cm}^3##.

##\text{The question is - how to relate this volume to the depth inside liquid?}##

I make a sketch of the problem situation. I need to find the depth inside water ##d=?##

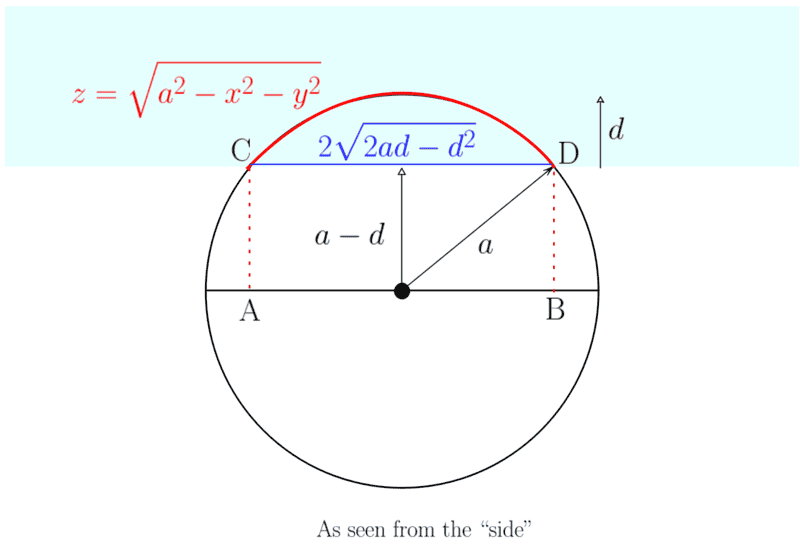If I "overturn" the basketball and looking from the "side", call its radius as ##a##, I would have an image like the one alongside. The red arc ##\color{red}{\stackrel{\large{\frown}}{CD}}## is the 2-D surface of the ball inside water whose ##(x,y,z)## coordinates at any point are related by ##z=\sqrt{a^2-x^2-y^2}##. The blue line ##\color{blue}{\overline{CD}}## marks the boundary of the region under water. Using the Pythagorean theorem, the length of the line ##{\color{blue}{\overline{CD}}}\text{AB}## is ##2\sqrt{2ad-d^2}##. We understand that this line ##{\color{blue}{\overline{CD}}}\text{AB}## is the diameter of the circle at the boundary of liquid.


Hence the volume of the region under water is the difference of the volume of region ##{\color{red}{\stackrel{\large{\frown}}{CD}}}\text{AB}## and ##{\color{blue}{\overline{CD}}}\text{AB}##.

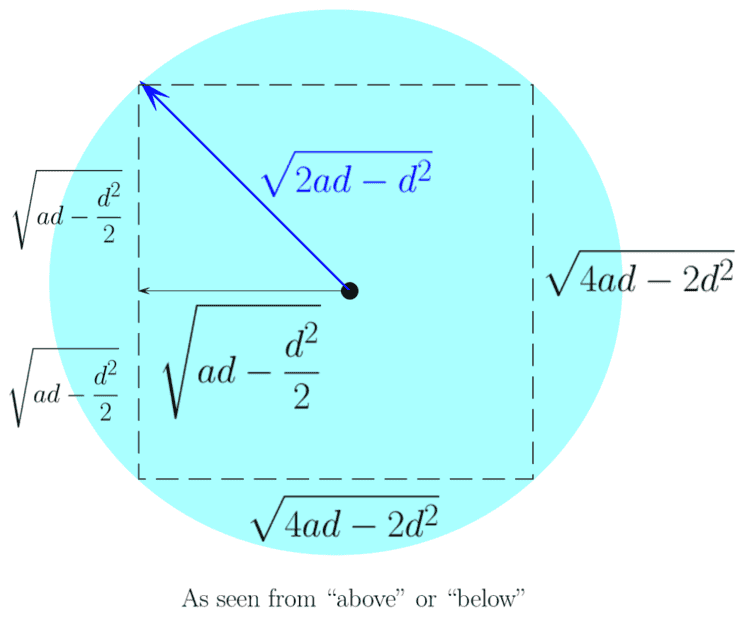The volume of the region ##{\color{blue}{\overline{CD}}}\text{AB}## is easy. It is a cuboid with a square on "top" of dimensions ##\sqrt{4ad-2d^2}##, calculated upon use of the Pythagorean theorem to find the side of a square given its radius (= ##\sqrt{2ad-d^2}##). The height of the cudoid (as seen from the "side") is ##(a-d)##. Hence the volume of the cuboid ##{\color{blue}{\overline{CD}}}\text{AB}## is : ##V_{\text{cuboid}}=2(2ad-d^2)(a-d)##.

But now to find the volume of the region ##{\color{red}{\stackrel{\large{\frown}}{CD}}}\text{AB}## whose upper serface is a portion of a sphere?

This is where am stuck. I am aware that the equation of the upper surface ##\color{red}{\stackrel{\large{\frown}}{CD}}## is ##\color{red}{z=\sqrt{a^2-x^2-y^2}}##. The lower surface of the same region is a square of dimensions ##\sqrt{4ad-2d^2}##. But how to find its volume?


Given my lack of progress on this crucial but elementary point, any hint would be welcome.

 

[haruspex]

Divide the submerged region into horizontal discs. If the ball has radius r and a disc thickness dx is distance x from the ball's centre, what, approximately, is its volume?

Btw, there is only one "a" in "flotation".

 

[brotherbobby]

Divide the submerged region into horizontal discs. If the ball has radius r and a disc thickness dx is distance x from the ball's centre, what, approximately, is its volume?

Btw, there is only one "a" in "flotation".

Yes, I can do the problem that way. I do it below roughly writing into ##\text{Sketchbook}^{\circledR}## and hope it's readable.

We end up with a cubic equation we have to solve to find ##d## : ##d^3-0.36d^2-5.73\times 10^{-4}=0##.

Since I don't know how to solve it, let me try online.

The answer comes out to be : ##\boxed{d = 4.2\;\text{cm}}##.

 

[brotherbobby]

The question is, can this be done in the way I wanted to do in post #1, taking the equation of a sphere and subtracting volumes of regions to find the volume enclosed.

 

[haruspex]

The question is, can this be done in the way I wanted to do in post #1, taking the equation of a sphere and subtracting volumes of regions to find the volume enclosed.

If one method leads to having to solve a cubic, and that cubic has no rational solution, then all solutions will lead to that.

 

[haruspex]

##d## : ##d^3-0.36d^2-5.73\times 10^{-4}=0##.

Sign error, but I'm guessing it’s just a typo.

 

[brotherbobby]

Sign error, but I'm guessing it’s just a typo.

Yes, it was a typo. The answer ##\boxed{d = 4.2\;\text{cm}}## correct.

If one method leads to having to solve a cubic, and that cubic has no rational solution, then all solutions will lead to that.

I agree, but I still want to solve it that way.

It's a bit like finding the volume of a sphere. You can break the sphere into concentric shells of radius ##x## and thickness ##{\rm{d}} x## : $$V = \int\limits_0^a 4\pi x^2 dx$$.
You can also use the equation of a sphere (##x^2+y^2+z^2=a^2##) and solve the triple integral finding volume : $$V=\int\limits_{-a}^{+a}dx \int\limits_{-\sqrt{a^2-x^2}}^{+\sqrt{a^2-x^2}}dy \int\limits_{-\sqrt{a^2-x^2-y^2}}^{+\sqrt{a^2-x^2-y^2}}dz$$
(Stuck though I am, I'd like to do it in the second way to see if I get the same answer)

 

[brotherbobby]

I am afraid to bring you back @haruspex to the (unsolved) problem above of finding the volume of the spherical cap , at least the way I'd want it. Forget the numerical details for now.

The goal : ##\textbf{To calculate the volume of the spherical dome using the method of calculus.}##

Now I succeeded in doing that using the method you said in post #2, using thin discs of thickness ##dx## at distances of (increasing) ##x## from the sphere's center. The answer came out to be ##\boxed{V = \dfrac{\pi d^2}{3}(\underline{3}a-d)}\qquad (1)##. I underline the 3 for a reason that will be apparent soon.

But why only the center? What if I wanted to choose a point at the base of the dome and move increasingly to its north pole?

Below is a sketch of what I have in mind.

Let's focus on the spherical cap. The volume of the thin disc is ##dV = \pi r^2(x) dx##, where the radius of the disc ##r(x)= \frac{b}{d}x##, using the method of similar triangles.

Thus the volume of the dome ##V= \dfrac{\pi b^2}{d^2}\int\limits_0^d x^2\;dx= \dfrac{\pi b^2}{d^2}\dfrac{d^3}{3}=\dfrac{\pi d}{3} (2ad-d^2)=\dfrac{\pi d^2}{3}(\underline{2}a-d)\qquad (2)##.

As you will note, the coefficient of a underlined in the brackets is 2 , different from the correct expression in (1) above which is 3.

Request : I'd be glad if you told me what's the error in my calculation.

 

[Hill]

what's the error in my calculation

This:

Because the triangles ##r/x## and ##b/d## are not similar.

 

[brotherbobby]

Because the triangles r/x and b/d are not similar.

Thank you. Sorry I got betrayed by the looks.