Algebraic Extensions - Dummit and Foote, Propn 11 and 12 ....

[Math Amateur]

I am reading Dummit and Foote, Chapter 13 - Field Theory.

I am currently studying Section 13.2 : Algebraic Extensions

I need some help with an aspect of Propositions 11 and 12 ... ...

Propositions 11 and 12 read as follows:

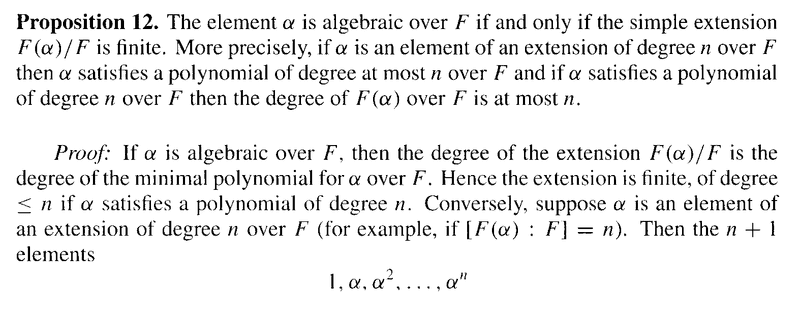
Now Proposition 11 states that the degree of ##F( \alpha )## over ##F## is equal to the degree of the minimum polynomial ... ... that is

##[ F( \alpha ) \ : \ F ] = \text{ deg } m_\alpha (x) = \text{ deg } \alpha##... ... BUT ... ...... ... Proposition 12 states that ... "if ##\alpha## is an element of an extension of degree ##n## over ##F##, then ##\alpha## satisfies a polynomial of degree at most ##n## over ##F## ... ... "Doesn't Proposition 11 guarantee that the polynomial (the minimum polynomial) is actually of degree equal to ##n##?Can someone please explain in simple terms how these statements are consistent?Help will be appreciated ...

Peter

 

[fresh_42]

I read it as follows:

1. In order to prove (##\alpha ## algebraic ##\Rightarrow \, F(\alpha) /F## finite), we don't care exact degrees.
2. The authors mentioned, that ##\deg [F(\alpha):F] = \deg m_\alpha(x)##.
3. In the direction of the proof which you quoted, all we have is that ##\alpha## is algebraic over ##F##. This means it satisfies some polynomial equation of degree ##n##. This polynomial doesn't need to be minimal, irreducible nor has ##\alpha ## to be outside of ##F##. That it is of finite degree is all that counts. We simply don't bother more than that.

 

[andrewkirk]

This one is easier than your usual challenges!

The trouble arises because the ##\alpha## in the second sentence is not necessarily the same as the one in the first sentence. It might have been clearer if they'd used ##\beta## instead of ##\alpha## in the second and subsequent sentences.

Let the extension of interest be ##F(\alpha) / F## where ##\alpha## is the root of an irreducible quadratic in ##F[x]##.
Consider a ##\beta## that is in ##F##. That ##\beta## is also in the extension. But it is a root of the degree-1 ##F[x]## polynomial ##x-\beta##.

On the other hand, ##\alpha## is also in the extension, and the minimal polynomial for that has degree two.

So some elements of the extension (the ones that are not already in ##F##) have degree two, and some (those that are in ##F##) have degree one.

 

[Math Amateur]

Thanks fresh_42 and Andrew ... appreciate your help ...

Still thinking over what you have said ...

Peter